Question

Multiple Choice The [(a) midpoint of mass, (b) mass point, (c) center of mass] of a system is the point that acts as if all the mass is concentrated at that point.

Question

Multiple Choice If an object of mass \(m\) is located on the \(x\)-axis a distance \(d\) from the origin, then the moment of the mass about the origin is [(a) \(0,\) (b) \(d\), (c) \(md\), (d) \(m]\).

Question

True or False A homogeneous lamina is made of material that has a constant density.

Question

Multiple Choice If a homogeneous lamina is symmetric about a line \(L\), then its [(a) centroid, (b) moment of mass, (c) axis] lies on \(L\).

Question

True or False The centroid of a lamina \(R\) always lies within \(R\).

Question

Multiple Choice The center of mass of a homogeneous lamina \(R\) is located at the [(a) centroid, (b) midpoint, (c) equilibrium point] of \(R,\) the geometric center of the lamina.

Question

\(m_{1}=20, m_{2}=50\) located, respectively, at \(4\) and \(10\)

Question

\(m_{1}=10, m_{2}=3\) located, respectively, at \(-2\) and \(3\)

Question

\(m_{1}=4, m_{2}=3, m_{3}=3, m_{4}=5\) located, respectively, at \(-1, 2, 4, 3\)

Question

\(m_{1}=7, m_{2}=3, m_{3}=2, m_{4}=4\) located, respectively, at \(6, -2, -4, -1\)

Question

\(m_{1}=4, m_{2}=8, m_{3}=1\) located, respectively, at the points \((0,2), (2,1), (4,8)\)

Question

\(m_{1}=6, m_{2}=2, m_{3}=10\) located, respectively, at the points \((-1,-1), (12,6), (-1,-2)\)

Question

\(m_{1}=4, m_{2}=3, m_{3}=3, m_{4}=5\) located, respectively, at the points \((-1,2), (2,3), (4,5), (3,6)\)

Question

\(m_{1}=8, m_{2}=6, m_{3}=3, m_{4}=5\) located, respectively, at the points \((-4,4), (0,5), (6,4), (-3,-5)\)

Question

\(y=2x+3, y=0, x=-1, x=2\)

Question

\(y=\dfrac{3-x}{2}, y=0, x=-1, x=3\)

Question

\(y=x^{2}, y=0, x=3\)

Question

\(y=x^{3}, x=2, y=0\)

Question

\(y=4x-x^{2}\) and the \(x\)-axis

Question

\(y=x^{2}+x+1, x=0, x=4\), and the \(x\)-axis

Question

\(y=\sqrt{x}, x=4, y=0\)

Question

\(y=\sqrt[3]{x}, x=8, y=0\)

Question

The solid torus formed by revolving the circle \((x-4)^{2}+y^{2}=9\) about the \(y\)-axis

Question

The solid torus formed by revolving the circle \(x^{2}+(y-2)^{2}=1\) about the \(x\)-axis

Question

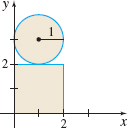
Question

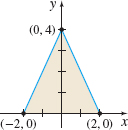
Question

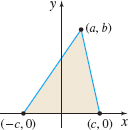
Question

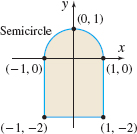
Question

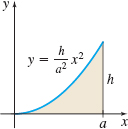
Question

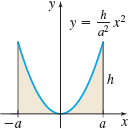
Question

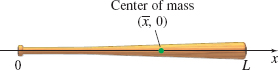
Center of Mass of a Baseball Bat Inspection of a baseball bat shows that it gets progressively thicker, that is, more massive, beginning at the handle and ending at the top. If the bat is aligned so that the \(x\)-axis runs through the center of the bat, the density \(\lambda\), in kilograms per meter, can be modeled by \(\lambda =kx,\) where \(k\) is the constant of proportionality. The mass \(M\) of a bat of length \(L\) meters is \(\int_{0}^{L}\lambda ~{\it dx}\). The center of mass \(\overline{x}\) of the bat is \(\dfrac{\int_{0}^{L}x\lambda ~{\it dx}}{M}\).

1. Find the mass of the bat.
2. Use the result of (a) to find the constant of proportionality \(k.\)
3. Find the center of mass of the bat.
4. Where is the “sweet spot” of the bat (the best place to make contact with the ball)? Explain why?
5. Give an explanation for the representation of the mass \(M\) by \(\int_{0}^{L}\lambda ~{\it dx}\).

466

Question

The Pappus Theorem for Volume Find the volume of the solid formed by revolving the region bounded by the graphs of \(y=\sqrt{x-1}, y=0\), and \(x=2\) about the \(y\)-axis.

Question

The Pappus Theorem for Volume Find the volume of the solid formed by revolving the triangular region bounded by the lines \(x=3,y=2\), and \(x+2y=9\) about the \(y\)-axis.

Question

The Pappus Theorem for Volume Find the volume of a right circular cone of radius \(R\) and height \(H.\)

Question

The Pappus Theorem for Volume Find the volume of the solid formed by revolving the triangular region whose vertices are at the points \((1,1),(5,3)\), and \((3,3)\) about the \(x\)-axis.

Question

Centroid of a Right Triangle Find the centroid of a triangular region with vertices at \(( 0,0) ,\) \(( 0,4) ,\) and \(( 3,0) .\)

Question

Centroid of a Triangle

1. Show that the centroid of a triangular region is located at the intersection of the medians. [Hint: Place the vertices of the triangle at \((a,0)\), \((b,0)\), and \((0,c)\), \(a<0\), \(b>0\), \(c>0\).
2. Show that the centroid of a triangular region divides each median into two segments whose lengths are in the ratio 2:1.

Question

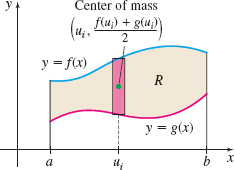
Derive formulas for finding the centroid of a lamina bounded by the graphs of \(y=f(x)\) and \(y=g(x)\) and the lines \(x=a\) and \(x=b\), as shown in the figure below.

Question

\(y=x^{2/3}\) and \(y=x^{2}\) from \(x=-1\) to \(x=1\)

Question

\(y=\dfrac{1}{x}\), and the lines \(y=\dfrac{1}{4},\) and \(x=1\)

Question

\(y=-x^{2}+2\) and \(y=\vert x\vert \)

Question

\(y=\sqrt{x}\) and \(y=x^{2}\)

Question

\(y=4-x^{2}\) and \(y=x+2\).

Question

\(y=9-x^{2}\) and \(y=|x|\).

Question

Laminas with Variable Density Suppose a lamina is determined by a region \(R\) enclosed by the graph of \(y=f(x)\), the \(x\)-axis, and the lines \(x=a\) and \(x=b\), where \(f\) is continuous and nonnegative on the closed interval [a,b]. Suppose further that the density of the material at \((x,y)\) is \(\rho =\rho (x)\), where \(\rho \) is continuous on \([a,b]\). Show that the center of mass \(( \bar{x},\bar{y})\) of \(R\) is given by \begin{eqnarray*} \bar{x}&=&\dfrac{M_{y}}{M}=\dfrac{\displaystyle \int_{a}^{b}\rho (x) x f(x)~{\it dx}}{\displaystyle \int_{a}^{b}\rho (x) f(x)\,{\it dx}} \\[5pt] \bar{y}&=&\dfrac{M_{x}}{M}=\dfrac{\dfrac{1}{2}\displaystyle \int_{a}^{b}\rho (x) [f(x)] ^{2} \,{\it dx}}{\displaystyle \int_{a}^{b} \rho (x) f(x)\,{\it dx}} \end{eqnarray*}

(Hint: Partition the interval \([a,b] \) into \(n\) subintervals, and use an argument similar to the one used for the centroid of a homogeneous lamina. Assume that if \(\Delta x\) is small, then \(R_{i}\) is homogeneous.)

Question

Lamina with Variable Density Use the results of Problem 45 to find the center of mass of a lamina enclosed by \(f(x) =\dfrac{1}{2}x^{2},\) the \(x\)-axis, and the lines \(x=1\) and \(x=e.\) Suppose the density at any point \(( x,y)\) of the lamina is \(\rho(x) =\dfrac{1}{x^{3}}.\)

Question

Lamina with Variable Density Use the results of Problem 45 to find the center of mass of the lamina enclosed by \(y=\sqrt{x+1}\), and the lines \(x=0\) and \(y=3\), where the density of the lamina is \(\rho (x)=x\).

Question

\(f(x)=x;[0,3];\rho (x)=x\)

Question

\(f(x)=3x-1;[1,4];\rho (x)=x\)

Question

\(f(x)=2x;[0,1];\rho (x)=x+1\)

Question

\(f(x)=x;[1,4];\rho (x)=x+1\)