Practicing Geology Exercise

How Big Is Our Planet?

How was it discovered that Earth is round with a circumference of 40,000 km? No one had looked down on Earth from space before the early 1960s, but its shape and size was understood long before that time. In 1492, Columbus set a westward course for India because he believed in a theory of geodesy that had been favored by Greek philosophers: we live on a sphere. His math was poor, however, so he badly underestimated Earth’s circumference. Instead of a shortcut, he took the long way around, finding a New World instead of the Spice Islands! Had Columbus properly understood the ancient Greeks, he might not have made this fortuitous mistake, because they had accurately measured Earth’s size more than 17 centuries earlier.

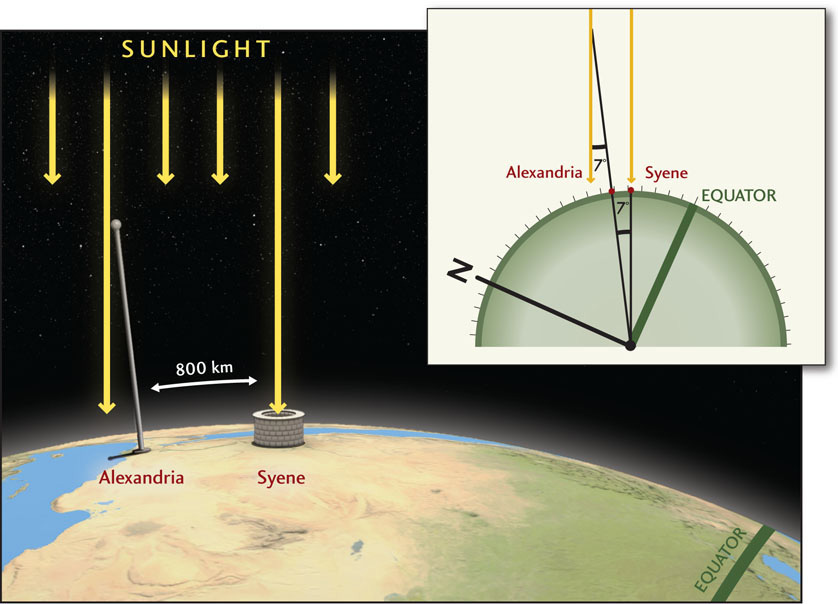
The credit for determining Earth’s size goes to Eratosthenes, a Greek who was chief librarian at the Great Library of Alexandria in Egypt. Sometime around 250 b.c., a traveler told him about an interesting observation. At noon on the first day of summer (June 21), a deep well in the city of Syene, about 800 km south of Alexandria, was completely lit up by sunlight because the Sun was directly overhead. Acting on a hunch, Eratosthenes did an experiment. He set up a vertical pole in his own city, and at high noon on the first day of summer, the pole cast a shadow.

Eratosthenes assumed that the Sun was very far away, so that the light rays falling on the two cities were parallel. Knowing that the Sun cast a shadow in Alexandria but was directly overhead at the same time in Syene, Eratosthenes could demonstrate with simple geometry that the ground surface must be curved. He knew that the most perfect curved surface is a sphere, so he hypothesized that Earth had a spherical shape (the Greeks admired geometric perfection). By measuring the length of the pole’s shadow in Alexandria, he calculated that if vertical lines through the two cities could be extended to Earth’s center, they would intersect at an angle of about 7°, which is about 1/50 of a full circle (360°). The distance between the two cities was known to be about 800 km in today’s measurements. From these figures, Eratosthenes calculated a circumference for Earth that is very close to the modern value:

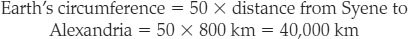
With this figure for Earth’s circumference, it was a simple matter to calculate its radius. Eratosthenes knew that, for any circle, the circumference is equal to 2π (pi) times the radius, where π is about 3.14. Therefore, he divided his estimate of Earth’s circumference by 2p to find its radius:

By these calculations, Eratosthenes arrived at a simple and elegant scientific model: Earth is a sphere with a radius of about 6370 km.

In this powerful demonstration of the scientific method, Eratosthenes made observations (the length of the shadow), formed a hypothesis (spherical shape), and applied some mathematical theory (spherical geometry) to propose a remarkably accurate model of Earth’s physical form. His model correctly predicted other types of measurements, such as the distance at which a ship’s tall mast would disappear over the horizon. Moreover, knowing Earth’s shape and size allowed Greek astronomers to calculate the sizes of the Moon and Sun and the distances of these bodies from Earth. This story makes clear why well-designed experiments and good measurements are central to the scientific method: they give us new information about the natural world.

BONUS PROBLEM: The volume of a sphere is given by

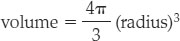
From this formula, calculate Earth’s volume in cubic kilometers.