CHAPTER 12 EXERCISES
Question 12.42
![]() 12.42 The effect of increased variation within groups. The One-
12.42 The effect of increased variation within groups. The One-
(a) The black dots are at the means of the three groups. Move these up and down until you get a configuration that gives a P-value of about 0.01. What is the value of the F statistic?
(b) Now increase the variation within the groups by sliding the standard deviation bar to the right. Describe what happens to the F statistic and the P-value.
(c) Using between-
and within- group variation, explain why the F statistic and P-value change in this way.
Question 12.43
![]() 12.43 The effect of increased variation between groups. Set the pooled standard error for the One-
12.43 The effect of increased variation between groups. Set the pooled standard error for the One-
(a) What is the F statistic? Give its P-value.
(b) Drag the mean of the second group up and the mean of the third group down. Describe the effect on the F statistic and its P-value. Explain why they change in this way.
12.43 (a) F can be made very small and the P-value close to 1. (b) F increases and P-value decreases.
Question 12.44
![]() 12.44 The effect of increased sample size. Set the pooled standard error for the One-
12.44 The effect of increased sample size. Set the pooled standard error for the One-
(a) What are the F statistic, its degrees of freedom, and the P-value?
(b) Slide the sample size bar to the right so n = 80. Also drag the black dots back to the values of 5.00, 4.50, and 5.25, respectively. What are the F statistic, its degrees of freedom, and the P-value?
(c) Explain why the F statistic and P-value change in this way as n increases.
Question 12.45
12.45 The multiple-
| Group | n | ˉx | s |
|---|---|---|---|
| DSL | 19 | 104.49 | 26.09 |
| Cable | 20 | 119.98 | 40.39 |
| Fiber | 8 | 83.87 | 31.78 |
(a) Plot the means versus the platform type. Does there appear to be a difference in pricing?
(b) Is it reasonable to assume that the variances are equal? Explain.
(c) The F statistic is 3.39. Give the degrees of freedom and either an approximate (from a table) or an exact (from software) P-value. What do you conclude?
12.45 (a) Cable has the highest prices; Fiber has the cheapest. (b) Yes, 40.39 < 2(26.09). (c) df = 2 and 44, 0.025 < P-value < 0.05.
Question 12.46
12.46 The two-
Question 12.47
12.47 Winery websites. As part of a study of British Columbia wineries, each of the 193 wineries were classified into one of three categories based on their website features. The Presence stage just had information about the winery. The Portals stage included order placement and online feedback. The Transactions Integration stage included direct payment or payment through a third party online. The researchers then compared the number of market integration features of each winery (for example, in-
| Stage | n | ˉx | s |
|---|---|---|---|
| Presence | 55 | 3.15 | 2.264 |
| Portals | 77 | 4.75 | 2.097 |
| Transactions | 61 | 4.62 | 2.346 |
(a) Plot the means versus the stage of website. Does there appear to be a difference in the average number of market integration features?
(b) Is this an observational study or an experiment? Explain your answer.
(c) Is it reasonable to assume the variances are equal? Justify your reasoning.
(d) The data are counts (integer values). Also, based on the means and standard deviations, the distributions are skewed (can’t have a negative count). Do you think this lack of Normality poses a problem for ANOVA? Explain your answer.
(e) The F statistic for these data is 9.594. Give the degrees of freedom and P-value. What do you conclude?
12.47 (a) Portals and Transactions have higher integration features than Presence. (b) An observational study. They are not imposing a treatment on the winery. (c) Yes; 2.346 < 2(2.097). (d) Our inference is based on sample means, which will be approximately Normal given the sample sizes. (e) F(2, 190); P-value < 0.001.
Question 12.48
12.48 Time levels of scale. Recall Exercise 7.62 (page 456). This experiment actually involved three groups. The last group was told the construction project would last 12 months. Here is a summary of the interval lengths (in days) between the earliest and latest completion dates.
| Group | n | ˉx | s |
|---|---|---|---|
| 1: 52 weeks | 30 | 84.1 | 55.8 |
| 2: 12 months | 30 | 104.6 | 70.1 |
| 3: 1 year | 30 | 139.6 | 73.1 |
(a) Is this an observational study or an experiment? Explain your answer.
(b) Use graphical methods to describe the three populations.
(c) Examine the conditions necessary for ANOVA. Summarize your findings.
Question 12.49
12.49 Time levels of scale, continued. Refer to the previous exercise.
(a) Run the ANOVA and report the results.
(b) Use a multiple-
comparisons method to compare the three groups. State your conclusions.(c) The researchers hypothesized that the more fine-
grained the time unit presented to a participant, the smaller the reported interval would be. To test this, they performed a simple linear regression using the group labels 1, 2, and 3 as the predictor variable. They found the slope (b = 27.8) significantly different from 0 (P < 0.005) and thus concluded the data supported their hypothesis. Do you think this is an appropriate way to test their hypothesis? Explain your answer.
12.49 (a) H0: μ1 = μ2 = μ3, Hα: not all of the μi are equal, F = 5.31, P-value = 0.0067. (b) The Bonferroni shows that group 2 is not significantly different from either group 1 or group 3, but group 3 is significantly different (larger) from group 1. (c) This is not appropriate. The regression assumes that group 2 (coded as 2) would have twice the effect of group 1 (coded as 1), and group 3 (coded as 3) would have three times the effect of group 1, etc. This is likely not true.
Question 12.50
12.50 Facebook recruitment of young adult smokers. Studies about tobacco use have had difficulties recruiting young adults. Because Facebook is visited daily by a very large percent of young adults, researchers decided to investigate the effectiveness of using Facebook to recruit young adults for a smoking cessation trial. Thirty-
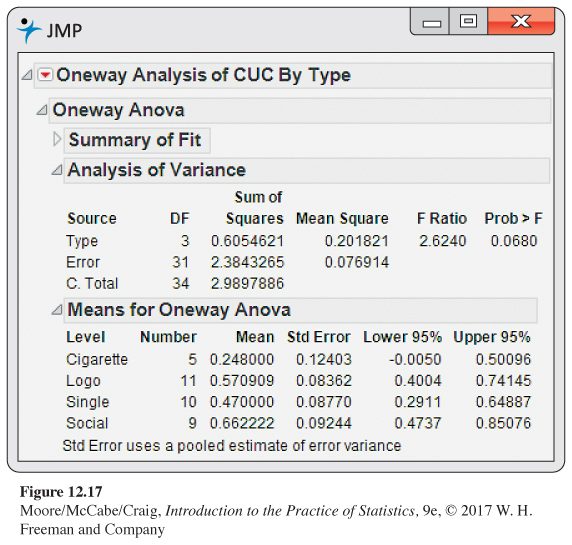
(a) Figure 12.17 contains the JMP output for the analysis of the CUCs by image type. State your conclusions based on this output.
(b) The researchers concluded there were significant differences between the means for Cigarette and Logo and between the means for Cigarette and Social. Perform these comparisons using the least-
significant differences (LSD) method. Do these comparisons have P-values below ⍺ = 0.05? (c) Given your results in part (a), should the researchers report the results in part (b)? Explain your answer.
Question 12.51
12.51 Organic foods and morals? Organic foods are often marketed using moral terms such as “honesty’’ and “purity.’’ Is this just a marketing strategy, or is there a conceptual link between organic food and morality? In one experiment, 62 undergraduates were randomly assigned to one of three food conditions (organic, comfort, and control).13 First, each participant was given a packet of four food types from the assigned condition and told to rate the desirability of each food on a seven-
(a) Make a table giving the sample size, mean, and standard deviation for each group. Is it reasonable to pool the variances?
(b) Generate a histogram for each of the groups. Can we feel confident that the sample means are approximately Normal? Explain your answer.
12.51 (a) See accompanying table. Pooling is appropriate, 0.621669 < 2(0.572914). (b) While the distributions aren’t Normal, there are no outliers or extreme departures from Normality that would invalidate the results. We can likely proceed with the ANOVA.
| Score | |||
|---|---|---|---|
| Level of food | N | Mean | Std dev |
| Comfort | 22 | 4.8873 | 0.5729 |
| Control | 20 | 5.0825 | 0.6217 |
| Organic | 20 | 5.5835 | 0.5936 |
Question 12.52
12.52 Organic foods and morals, continued. Refer to the previous exercise.
(a) Analyze the scores using analysis of variance. Report the test statistic, degrees of freedom, and P-value.
(b) Assess the assumptions necessary for inference by examining the residuals. Summarize your findings.
(c) Compare the groups using the least-
significant differences method. (d) A higher score is associated with a harsher moral judgment. Using the results from parts (a) and (b), write a short summary of your conclusions.
Question 12.53
12.53 Organic foods and friendly behavior? Refer to Exercise 12.51 for the design of the experiment. After rating the moral transgressions, the participants were told “that another professor from another department is also conducting research and really needs volunteers.’’ They were told that they would not receive compensation or course credit for their help and then were asked to write down the number of minutes (out of 30) that they would be willing to volunteer. This sort of question is often used to measure a person’s prosocial behavior.
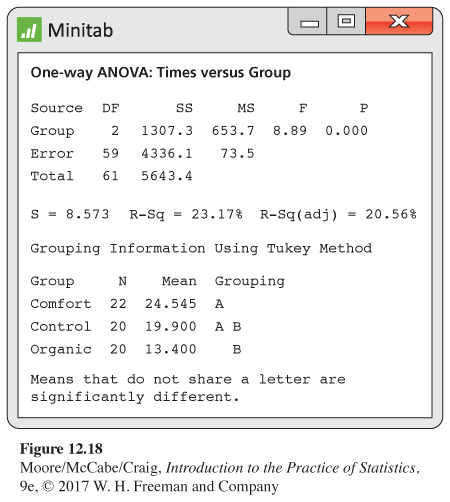
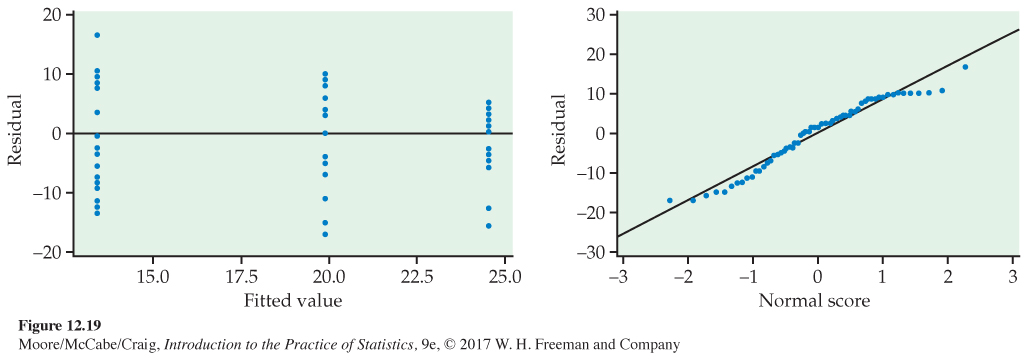
(a) Figure 12.18 contains the Minitab output for the analysis of this response variable. Write a one-
paragraph summary of your conclusions. (b) Figure 12.19 contains a residual plot and a Normal quantile plot of the residuals. Are there any concerns regarding the assumptions necessary for inference? Explain your answer.
12.53 (a) H0: μ1 = μ2 = μ3, Hα : not all of the μi are equal, F = 8.89, P-value = 0.000. There are significant differences in the number of minutes that the three groups are willing to volunteer. The Comfort group is willing to donate significantly more minutes than the Organic group. The Control group is in the middle, not significantly different from either the Comfort or Organic group. (b) The residual plot shows a possible violation of constant variance. The Normal quantile plot looks fine and shows a roughly Normal distribution.
Question 12.54
12.54 Massage therapy for osteoarthritis of the knee. Various studies have shown the benefits of massage to manage pain. In one study, 125 adults suffering from osteoarthritis of the knees were randomly assigned to one of five eight-
| Regimen | n | ˉx | s |
|---|---|---|---|
| 30 min massage 1 × /wk | 22 | −17.4 | 17.9 |
| 30 min massage 2 × /wk | 24 | −18.4 | 20.7 |
| 60 min massage 1 × /wk | 24 | −24.0 | 18.4 |
| 60 min massage 2 × /wk | 25 | −24.0 | 19.8 |
| Usual care, no massage | 24 | −6.3 | 14.6 |
(a) What proportion of adults dropped out of the study before completion?
(b) Is it reasonable to use the assumption of equal standard deviations when we analyze these data? Give a reason for your answer.
(c) Find the pooled standard deviation.
(d) The SS(Regimen) = 5060.346. Test the null hypothesis that the mean change in WOMAC-
Global score is the same for all regimens. (e) There are 10 pairs of means to compare. For the Bonferroni multiple-
comparisons method, the critical t -value is 2.863. Which pairs of means are found to be significantly different? Write a short summary of your analysis.
Question 12.55
12.55 Financial incentives for weight loss. The use of financial incentives has shown promise in promoting weight loss and healthy behaviors. In one study, 104 employees of the Children’s Hospital of Philadelphia, with BMIs of 30 to 40 kilograms per square meter (kg/m2), were each randomly assigned to one of three weight-
(a) Make a table giving the sample size, mean, and standard deviation for each group.
(b) Is it reasonable to pool the variances? Explain your answer.
(c) Generate a histogram for each of the programs. Can we feel confident that the sample means are approximately Normal? Defend your answer.
12.55 (a) See accompanying table. (b) Yes, the largest s is less than twice the smallest s; 11.501 < 2(9.078) = 18.156. (c) All three distributions are roughly Normal.
| Loss | |||
|---|---|---|---|
| Level of group | N | Mean | Std dev |
| Control | 35 | − 1.0086 | 11.5007 |
| Group | 34 | − 10.7853 | 11.1392 |
| Individual | 35 | − 3.7086 | 9.0784 |
Question 12.56
12.56 Financial incentives for weight loss, continued. Refer to the previous exercise.
(a) Analyze the change in weight using analysis of variance. Report the test statistic, degrees of freedom, P-value, and your conclusions.
(b) Even though you assessed the model assumptions in the previous exercise, let’s check the assumptions again by examining the residuals. Summarize your findings.
(c) Compare the groups using the least-
significant difference method. (d) Using the results from parts (a), (b), and (c), write a short summary of your conclusions.
Question 12.57
12.57 Changing the response variable. Refer to the previous two exercises, where we compared three weight-
(a) Convert the weight loss from pounds to kilograms by dividing each response by 2.2.
(b) Analyze these new weight changes using analysis of variance. Compare the test statistic, degrees of freedom, and P-value you obtain here with those reported in part (a) of the previous exercise. Summarize what you find.
12.57 (a) All weight loss values are divided by 2.2. (b) F = 7.77, df = 2 and 101, P-value = 0.0007. The results are identical with the transformed data.
Question 12.58
![]() 12.58 Do labels matter? A study was performed to examine the self-
12.58 Do labels matter? A study was performed to examine the self-
| Label | n | ˉx |
|---|---|---|
| Asian American | 130 | 2.93 |
| Specific ethnicity | 248 | 3.00 |
| Ethnicity American | 174 | 3.01 |
| Other | 68 | 3.39 |
(a) What are the numerator and denominator degrees of freedom for the F test?
(b) Using the formula on page 662 and the preceding results, calculate SSG.
(c) Given SSE = 797.25, use your result from part (b) to compute the F statistic.
(d) Compute the P-value and state your conclusions.
(e) Without doing any additional analysis, describe the pattern in the means that is likely responsible for your conclusions in part (d).
Question 12.59
12.59 Do we experience emotions differently? Do people from different cultures experience emotions differently? One study designed to examine this question collected data from 410 college students from five different cultures.17 The participants were asked to record, on a 1 (never) to 7 (always) scale, how much of the time they typically felt eight specific emotions. These were averaged to produce the global emotion score for each participant. Here is a summary of this measure:
| Culture | n | Mean (s) |
|---|---|---|
| European American | 46 | 4.39 (1.03) |
| Asian American | 33 | 4.35 (1.18) |
| Japanese | 91 | 4.72 (1.13) |
| Indian | 160 | 4.34 (1.26) |
| Hispanic American | 80 | 5.04 (1.16) |
Note that the convention of giving the standard deviations in parentheses after the means saves a great deal of space in a table such as this.
(a) From the information given, do you think that we need to be concerned that a possible lack of Normality in the data will invalidate the conclusions that we might draw using ANOVA to analyze the data? Give reasons for your answer.
(b) Is it reasonable to use a pooled standard deviation for these data? Why or why not?
(c) The ANOVA F statistic was reported as 5.69. Give the degrees of freedom and either an approximate (from a table) or an exact (from software) P-value. Sketch a picture of the F distribution that illustrates the P-value. What do you conclude?
(d) Without doing any additional formal analysis, describe the pattern in the means that appears to be responsible for your conclusion in part (c). Are there pairs of means that are quite similar?
12.59 (a) The variation in sample size is some cause for concern, but there can be no extreme outliers in a 1-to-7 scale. (b) Yes; 1.26 < 2(1.03). (c) F(4, 405), P-value < 0.0002. (d) Hispanic Americans are highest, Japanese in the middle, and the other three are the lowest.
Question 12.60
![]() 12.60 The emotions study, continued. Refer to the previous exercise. The experimenters also measured emotions in some different ways. For a period of a week, each participant carried a device that sounded an alarm at random times during a three-
12.60 The emotions study, continued. Refer to the previous exercise. The experimenters also measured emotions in some different ways. For a period of a week, each participant carried a device that sounded an alarm at random times during a three-
| Culture | n | Frequency mean (s) |
Intensity mean (s) |
Recall mean (s) |
|---|---|---|---|---|
| European American | 46 | 82.87 (18.26) | 2.79 (0.72) | 49.12 (22.33) |
| Asian American | 33 | 72.68 (25.15) | 2.37 (0.60) | 39.77 (23.24) |
| Japanese | 91 | 73.36 (22.78) | 2.53 (0.64) | 43.98 (22.02) |
| Indian | 160 | 82.71 (17.97) | 2.87 (0.74) | 49.86 (21.60) |
| Hispanic American | 80 | 92.25 (8.85) | 3.21 (0.64) | 59.99 (24.64) |
| F statistic | 11.89 | 13.10 | 7.06 |
(a) For each response variable, state whether or not it is reasonable to use a pooled standard deviation to analyze these data. Give reasons for your answer.
(b) Give the degrees of freedom for the F statistics and find the associated P-values. Summarize what you can conclude from these ANOVA analyses.
(c) Summarize the means, paying particular attention to similarities and differences across cultures and across variables. Include the means from the previous exercise in your summary.
(d) The European American and Asian American subjects were from the University of Illinois, the Japanese subjects were from two universities in Tokyo, the Indian subjects were from eight universities in or near Kolkata, and the Hispanic American subjects were from California State University at Fresno. Participants were paid $25 or an equivalent monetary incentive for the Japanese and Indians. Ads were posted on or near the campuses to recruit volunteers for the study. Discuss how these facts influence your conclusions and the extent to which you would generalize the results.
(e) The percents of female students in the samples were as follows: European American, 83%; Asian American, 67%; Japanese, 63%; Indian, 64%; and Hispanic American, 79%. Use a chi-
square test to compare these proportions (see Section 9.1, page 536) and discuss how this information influences your interpretation of the results that you have found in this exercise.
Question 12.61
12.61 Shopping and bargaining in Mexico. Price haggling and other bargaining behaviors among consumers have been observed for a long time. However, research addressing these behaviors, especially in a real-
| Bargainer | n | Average reduction |
|---|---|---|
| Hispanic male | 40 | 1.055 |
| Anglo male | 40 | 1.050 |
| Hispanic female | 40 | 2.310 |
(a) To compare the mean reductions in price, what are the degrees of freedom for the ANOVA F statistic?
(b) The reported test statistic is F = 8.708. Give an approximate (from a table) or exact (from software) P-value. What do you conclude?
(c) To what extent do you think the results of this study can be generalized? Give reasons for your answer.
12.61 (a) df = 2, 117. (b) P-value < 0.001. (c) Because the bargainer was the same person each time, the results would certainly not be generalizable.
Question 12.62
12.62 Restaurant ambiance and consumer behavior. There have been numerous studies investigating the effects of restaurant ambiance on consumer behavior. One study investigated the effects of musical genre on consumer spending.19 At a single high-
| Background music | Mean bill | n | s |
|---|---|---|---|
| Classical | 24.130 | 44 | 2.243 |
| Pop | 21.912 | 49 | 2.627 |
| None | 21.697 | 48 | 3.332 |
| Total | 22.531 | 141 | 2.969 |
(a) Plot the means versus the type of background music. Does there appear to be a difference in spending?
(b) Is it reasonable to assume that the variances are equal? Explain.
(c) The F statistic is 10.62. Give the degrees of freedom and either an approximate (from a table) or an exact (from software) P-value. What do you conclude?
(d) Refer back to part (a). Without doing any formal analysis, describe the pattern in the means that is likely responsible for your conclusion in part (c).
(e) To what extent do you think the results of this study can be generalized to other settings? Give reasons for your answer.
Question 12.63
12.63 Do isoflavones increase bone mineral density? Kudzu is a plant that was imported to the United States from Japan and now covers over seven million acres in the South. The plant contains chemicals called isoflavones that have been shown to have beneficial effects on bones. One study used three groups of rats to compare a control group with rats that were fed either a low dose or a high dose of isoflavones from kudzu.20 One of the outcomes examined was the bone mineral density in the femur (in grams per square centimeter). Here are the data:
| Treatment | Bone mineral density (g/cm2) | |||||||
|---|---|---|---|---|---|---|---|---|
| Control | 0.228 | 0.207 | 0.234 | 0.220 | 0.217 | 0.228 | 0.209 | 0.221 |
| 0.204 | 0.220 | 0.203 | 0.219 | 0.218 | 0.245 | 0.210 | ||
| Low dose | 0.211 | 0.220 | 0.211 | 0.233 | 0.219 | 0.233 | 0.226 | 0.228 |
| 0.216 | 0.225 | 0.200 | 0.208 | 0.198 | 0.208 | 0.203 | ||
| High dose | 0.250 | 0.237 | 0.217 | 0.206 | 0.247 | 0.228 | 0.245 | 0.232 |
| 0.267 | 0.261 | 0.221 | 0.219 | 0.232 | 0.209 | 0.255 | ||
(a) Use graphical and numerical methods to describe the data.
(b) Examine the assumptions necessary for ANOVA. Summarize your findings.
(c) Run the ANOVA and report the results.
(d) Use a multiple-
comparisons method to compare the three groups. (e) Write a short report explaining the effect of kudzu isoflavones on the femur of the rat.
12.63 (a) See accompanying table. (b) All three distributions appear to be reasonably close to Normal, and the standard deviations are suitable for pooling. (c) F = 7.72 (df 2 and 42), P-value = 0.001. (d) For Bonferroni, t** = 2.4937 and MSD = 0.01308. The high-dose mean is significantly different from the other two. (e) High doses of kudzu isoflavones increase BMD.
| n | ˉx | s | SE | |
|---|---|---|---|---|
| Control | 15 | 0.2189 | 0.01159 | 0.002992 |
| Low dose | 15 | 0.2159 | 0.01151 | 0.002972 |
| High dose | 15 | 0.2351 | 0.01877 | 0.004847 |
Question 12.64
![]() 12.64 Do poets die young? According to William Butler Yeats, “She is the Gaelic muse, for she gives inspiration to those she persecutes. The Gaelic poets die young, for she is restless, and will not let them remain long on earth.’’ One study designed to investigate this issue examined the age at death for writers from different cultures and genders.21 Three categories of writers examined were novelists, poets, and nonfiction writers. Most of the writers are from the United States, but Canadian and Mexican writers are also included.
12.64 Do poets die young? According to William Butler Yeats, “She is the Gaelic muse, for she gives inspiration to those she persecutes. The Gaelic poets die young, for she is restless, and will not let them remain long on earth.’’ One study designed to investigate this issue examined the age at death for writers from different cultures and genders.21 Three categories of writers examined were novelists, poets, and nonfiction writers. Most of the writers are from the United States, but Canadian and Mexican writers are also included.
(a) Use graphical and numerical methods to describe the data.
(b) Examine the assumptions necessary for ANOVA. Summarize your findings.
(c) Run the ANOVA and report the results.
(d) Use a contrast to compare the poets with the two other types of writers. Do you think that the quotation from Yeats justifies the use of a one-
sided alternative for examining this contrast? Explain your answer.(e) Use another contrast to compare the novelists with the nonfiction writers. Explain your choice for an alternative hypothesis for this contrast.
(f) Use a multiple-
comparisons procedure to compare the three means. How do the conclusions from this approach compare with those using the contrasts?
Question 12.65
12.65 Exercise and healthy bones. Many studies have suggested that there is a link between exercise and healthy bones. Exercise stresses the bones and this causes them to get stronger. One study examined the effect of jumping on the bone density of growing rats.22 There were three treatments: a control with no jumping, a low-
| Group | Bone density (mg/cm3) | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Control | 611 | 621 | 614 | 593 | 593 | 653 | 600 | 554 | 603 | 569 |
| Low jump | 635 | 605 | 638 | 594 | 599 | 632 | 631 | 588 | 607 | 596 |
| High jump | 650 | 622 | 626 | 626 | 631 | 622 | 643 | 674 | 643 | 650 |
(a) Make a table giving the sample size, mean, and standard deviation for each group of rats. Is it reasonable to pool the variances?
(b) Run the analysis of variance. Report the F statistic with its degrees of freedom and P-value. What do you conclude?
12.65 (a) See accompanying table. Yes; 27.364 < 2(16.594). (b) F = 7.98 (df = 2 and 27), P-value = 0.002.
| n | ˉx | s | |
|---|---|---|---|
| Control | 10 | 601.1 | 27.364 |
| Low jump | 10 | 612.5 | 19.329 |
| High jump | 10 | 638.7 | 16.594 |
Question 12.66
12.66 Exercise and healthy bones, continued. Refer to the previous exercise.
(a) Examine the residuals. Is the Normality assumption reasonable for these data?
(b) Use the Bonferroni or another multiple-
comparisons procedure to determine which pairs of means differ significantly. Summarize your results in a short report. Be sure to include a graph.
Question 12.67
12.67 Contrasts of interest. Refer to Exercise 12.61 (page 692). Given the group means and F statistic, we can determine that MSE = 2.421. Use this value and the other information in Exercise 12.61 to do the following.
(a) Test if there is a difference between the sexes.
(b) Test if there is a difference between nationalities.
(c) Explain why this study would have benefited from also including an Anglo female bargainer.
12.67 (a) ψsex = μ1 − μ3; csex = − 1.255, t = − 3.6; P-value < 0.001. (b) ψnat = μ1 − μ2; cnat = 0.005, t = 0.014; P-value > 0.25. (c) The conclusions from the contrasts were limited to only comparing sex among Hispanics and nationalities among males. Including an Anglo female would alleviate this limited inference.
Question 12.68
![]() 12.68 Orthogonal polynomial contrasts. Recall the Facebook friends study (page 648). Previous research has shown that the bigger one’s social network, the higher one’s social attractiveness. In fact, the relationship between the number of friends and social attractiveness is approximately linear. A reasonable question to ask is whether this is same sort of pattern exists within an online social network. With orthogonal polynomial contrasts, we can assess the contributions of different polynomial trends to the overall pattern. Given the five equally spaced levels of the factor in this study, we can investigate up to a quartic (x4) trend. The derivation of the coefficients is beyond the scope of this book, so we will just investigate the trends here. The coefficients for the linear, quadratic, and cubic trends follow:
12.68 Orthogonal polynomial contrasts. Recall the Facebook friends study (page 648). Previous research has shown that the bigger one’s social network, the higher one’s social attractiveness. In fact, the relationship between the number of friends and social attractiveness is approximately linear. A reasonable question to ask is whether this is same sort of pattern exists within an online social network. With orthogonal polynomial contrasts, we can assess the contributions of different polynomial trends to the overall pattern. Given the five equally spaced levels of the factor in this study, we can investigate up to a quartic (x4) trend. The derivation of the coefficients is beyond the scope of this book, so we will just investigate the trends here. The coefficients for the linear, quadratic, and cubic trends follow:
| Trend | a1 | a2 | a3 | a4 | a5 |
|---|---|---|---|---|---|
| Linear | −2 | −1 | 0 | 1 | 2 |
| Quadratic | 2 | −1 | −2 | −1 | 2 |
| Cubic | −1 | 2 | 0 | −2 | 1 |
(a) Plot the ai versus i for the linear trend. Describe the pattern. Suppose that all the μi were constant. What would the value of ψ equal?
(b) Plot the ai versus i for the quadratic trend. Describe the pattern. Suppose that all the μi were constant. What would the value of ψ equal? Suppose that μi = 5i (that is, a linear trend). What would the value of ψ equal?
(c) Test the hypotheses that there is a linear, quadratic, and cubic trend. What do you conclude?
Question 12.69
12.69 A comparison of different types of scaffold material. One way to repair serious wounds is to insert some material as a scaffold for the body’s repair cells to use as a template for new tissue. Scaffolds made from extracellular material (ECM) are particularly promising for this purpose. Because they are made from biological material, they serve as an effective scaffold and are then resorbed. Unlike biological material that includes cells, however, they do not trigger tissue rejection reactions in the body. One study compared six types of scaffold material.23 Three of these were ECMs and the other three were made of inert materials (MAT). There were three mice used per scaffold type. The response measure was the percent of glucose phosphated isomerase (Gpi) cells in the region of the wound. A large value is good, indicating that there are many bone marrow cells sent by the body to repair the tissue.
| Material | Gpi (%) | ||
|---|---|---|---|
| ECM1 | 55 | 70 | 70 |
| ECM2 | 60 | 65 | 65 |
| ECM3 | 75 | 70 | 75 |
| MAT1 | 20 | 25 | 25 |
| MAT2 | 5 | 10 | 5 |
| MAT3 | 10 | 15 | 10 |
(a) Make a table giving the sample size, mean, and standard deviation for each of the six types of material. Is it reasonable to pool the variances? Note that the sample sizes are small and the data are rounded.
(b) Run the analysis of variance. Report the F statistic with its degrees of freedom and P-value. What do you conclude?
12.69 (a) See accompanying table. Pooling is not appropriate, the largest s is more than twice the smallest s; 8.6603 > 2(2.8868) = 5.7736. (b) F = 137.94 (df = 5 and 12), P-value < 0.0005.
| n | Mean | s | |
|---|---|---|---|
| ECM1 | 3 | 65.00% | 8.66% |
| ECM2 | 3 | 63.33% | 2.89% |
| ECM3 | 3 | 73.33% | 2.89% |
| MAT1 | 3 | 23.33% | 2.89% |
| MAT2 | 3 | 6.67% | 2.89% |
| MAT3 | 3 | 11.67% | 2.89% |
Question 12.70
12.70 A comparison of different types of scaffold material, continued. Refer to the previous exercise.
(a) Examine the residuals. Is the Normality assumption reasonable for these data?
(b) Use the Bonferroni or another multiple-
comparisons procedure to determine which pairs of means differ significantly. Summarize your results in a short report. Be sure to include a graph. (c) Use a contrast to compare the three ECM materials with the three other materials. Summarize your conclusions. How do these results compare with those that you obtained from the multiple-
comparisons procedure in part (b)?
Question 12.71
12.71 Contrasts for the massage study. Refer to Exercise 12.54 (page 690). There are several comparisons of interest in this study. They are (1) usual care versus the average of the massage groups; (2) the average of the two 30-
(a) Express each contrast in terms of the means (μ’s) of the treatments.
(b) Give estimates with standard errors for each of the contrasts.
(c) Perform the significance tests for the contrasts. Summarize the results of your tests and your conclusions.
12.71 (a) ψ1 = μC − 0.25(μ30×1 + μ30×2 + μ60×1 + μ60×2), ψ2 = 0.5(μ30×1 + μ30×2) − 0.5(μ60×1 + μ60×2), ψ3 = 0.5(μ60×1 − μ60×2) − 0.5(μ30×1 − μ30×2). (b) c1 = 14.65, c2 = 6.1, c3 = − 0.5. SEC1=4.209, SEC2=SEC3=3.784. (c) t1 = 3.481, P-value = 0.0007; t2 = 1.612, P-value = 0.1097; t3 = − 0.132, P-value = 0.8952. The first two are significant, the third is not.
Question 12.72
12.72 A dandruff study. Analysis of variance (ANOVA) methods are often used in clinical trials where the goal is to assess the effectiveness of one or more treatments for a particular medical condition. One such study compared three treatments for dandruff and a placebo. The treatments were 1% pyrithione zinc shampoo (PyrI), the same shampoo but with instructions to shampoo two times (PyrII), 2% ketoconazole shampoo (Keto), and a placebo shampoo (Placebo). After six weeks of treatment, eight sections of the scalp were examined and given a score that measured the amount of scalp flaking on a 0 to 10 scale. The response variable was the sum of these eight scores. An analysis of the baseline flaking measure indicated that randomization of patients to treatments was successful in that no differences were found between the groups. At baseline, there were 112 subjects in each of the three treatment groups and 28 subjects in the Placebo group. During the clinical trial, three dropped out from the PyrII group and six from the Keto group. No patients dropped out of the other two groups.
(a) Find the mean, standard deviation, and standard error for the subjects in each group. Summarize these, along with the sample sizes, in a table and make a graph of the means.
(b) Run the analysis of variance on these data. Write a short summary of the results and your conclusion. Be sure to include the hypotheses tested, the test statistic with degrees of freedom, and the P-value.
Question 12.73
12.73 The dandruff study, continued. Refer to the previous exercise.
(a) Plot the residuals versus case number (the first variable in the data set). Describe the plot. Is there any pattern that would cause you to question the assumption that the data are independent?
(b) Examine the standard deviations for the four treatment groups. Is there a problem with the assumption of equal standard deviations for ANOVA in this data set? Explain your answer.
(c) Create Normal quantile plots for each treatment group. What do you conclude from these plots?
(d) Obtain the residuals from the analysis of variance and create a Normal quantile plot of these. What do you conclude?
12.73 (a) The plot shows granularity, but otherwise, independence is not violated. (b) Yes; 1.6 < 2(0.93) = 1.86. (c) The individual Normal quantile plots for each shampoo show a lot of granularity due to using integer values but most look at least roughly Normal. (d) The Normal quantile plot for the residuals shows that the residuals are Normally distributed.
Question 12.74
12.74 Comparing each pair of dandruff treatments. Refer to Exercise 12.72. Use the Bonferroni or another multiple-
Question 12.75
12.75 Testing several contrasts from the dandruff study. Refer to Exercise 12.72. There are several natural contrasts in this experiment that describe comparisons of interest to the experimenters. They are (1) Placebo versus the average of the other three treatments, (2) Keto versus the average of the two Pyr treatments, and (3) PyrI versus PyrII.
(a) Express each of these three contrasts in terms of the means (μ’s) of the treatments.
(b) Give estimates with standard errors for each of the contrasts.
(c) Perform the significance tests for the contrasts. Summarize the results of your tests and your conclusions.
12.75 (a) ψ1 = 1/3(μPyr1 + μPyr2 + μKeto) − μPlacebo, ψ2 = 0.5(μPyr1 + μPyr2) − μKeto, ψ3 = μPyr1 − μPyr2. (b) sp = 1.1958. c1 = − 12.51, SEC1 = 0.2355; c2 = 1.269, SEC2 = 0.1413; c3 = 0.191, SEC3 = 0.1609. (c) H0: ψi = 0, Ha: ψ1 ≠ 0; t1 = − 53.17, P-value1 < 0.0005; t2 = 8.98, P-value2 < 0.0005; t3 = 1.19, P-value3 = 0.2359. The Placebo mean is significantly higher than the average of the other three, while the Keto mean is significantly lower than the average of the two Pyr means. The difference between the Pyr means is not significant (meaning the second application of the shampoo is of little benefit).
Question 12.76
12.76 Changing the response variable. Refer to Exercise 12.69 (page 694), where we compared six types of scaffold material to repair wounds. The data are given as percents ranging from 5 to 75.
(a) Convert these percents into their decimal form by dividing by 100. Calculate the transformed means, standard deviations, and standard errors and summarize them, along with the sample sizes, in a table.
(b) Explain how you could have calculated the table entries directly from the table you gave in part (a) of Exercise 12.51.
(c) Analyze the decimal forms of the percents using analysis of variance. Compare the test statistic, degrees of freedom, P-value, and conclusion you obtain here with the corresponding values that you found in Exercise 12.51.
Question 12.77
12.77 More on changing the response variable. Refer to the previous exercise and Exercise 12.69 (page 694). A calibration error was found with the device that measured Gpi, which resulted in a shifted response. Add 5% to each response and redo the calculations. Summarize the effects of transforming the data by adding a constant to all responses.
12.77 The means increase by 5%, but everything else remains the same.
Question 12.78
![]() 12.78 Linear transformation of the response variable. Refer to the previous two exercises. Can you suggest a general conclusion regarding what happens to the test statistic, degrees of freedom, P-value, and conclusion when you perform analysis of variance on data that have been transformed by multiplying the raw data by a constant and then adding another constant? (That is, if y is the original data, we analyze y*, where y* = a + by and a and b ≠ 0 are constants.)
12.78 Linear transformation of the response variable. Refer to the previous two exercises. Can you suggest a general conclusion regarding what happens to the test statistic, degrees of freedom, P-value, and conclusion when you perform analysis of variance on data that have been transformed by multiplying the raw data by a constant and then adding another constant? (That is, if y is the original data, we analyze y*, where y* = a + by and a and b ≠ 0 are constants.)
Question 12.79
![]() 12.79 More on the Facebook friends study. Refer to the Facebook friends study that we began to examine in Example 12.3 (page 648). The explanatory variable in this study is the number of Facebook friends, with possible values of 102, 302, 502, 702, and 902. When using ANOVA, we treat the explanatory variable as categorical. An alternative analysis is to use simple linear regression. Perform this analysis and summarize the results. Plot the residuals from the regression model versus the number of Facebook friends. What do you conclude?
12.79 More on the Facebook friends study. Refer to the Facebook friends study that we began to examine in Example 12.3 (page 648). The explanatory variable in this study is the number of Facebook friends, with possible values of 102, 302, 502, 702, and 902. When using ANOVA, we treat the explanatory variable as categorical. An alternative analysis is to use simple linear regression. Perform this analysis and summarize the results. Plot the residuals from the regression model versus the number of Facebook friends. What do you conclude?
12.79 All distributions are reasonably Normal and standard deviations are close enough to justify pooling. For PRE1, F = 1.13, P-value = 0.329; for PRE2, F = 0.11, P-value = 0.895. There is no reason to believe that the mean pretest scores differ between methods.
Question 12.80
![]() 12.80 Using the table of group means and standard deviations. Refer to Exercise 12.6 (page 655). Using the table of group means and standard deviations, construct an ANOVA table similar to that on page 662.
12.80 Using the table of group means and standard deviations. Refer to Exercise 12.6 (page 655). Using the table of group means and standard deviations, construct an ANOVA table similar to that on page 662.
(a) Based on the F statistic and degrees of freedom, compute the P-value. What do you conclude?
(b) Perform pairwise comparisons using the LSD method to determine which coffeehouses have different average ages.
Question 12.81
12.81 Planning another emotions study. Scores on an emotional scale were compared for five different cultures in Exercise 12.59 (page 691). Suppose that you are planning a new study using the same outcome variable. Your study will use European American, Asian American, and Hispanic American students from a large university.
(a) Explain how you would select the students to participate in your study.
(b) Use the data from Exercise 12.59 to perform power calculations to determine sample sizes for your study.
(c) Write a report that could be understood by someone with limited background in statistics and that describes your proposed study and why you think it is likely that you will obtain interesting results.
12.81 (b) Answers will vary with choice of Hα and desired power. For example, with the alternative μ1 = μ2 4.4, μ3 = 5, and σ = 1.2, three samples of size 75 will produce power 0.78.
Question 12.82
![]() 12.82 Planning another isoflavone study. Exercise 12.63 (page 692) gave data for a bone health study that examined the effect of isoflavones on rat bone mineral density. In this study, there were three groups. Controls received a placebo, and the other two groups received either a low or a high dose of isoflavones from kudzu. You are planning a similar study of a new kind of isoflavone. Use the results of the study described in Exercise 12.63 to plan your study. Write a proposal explaining why your study should be funded.
12.82 Planning another isoflavone study. Exercise 12.63 (page 692) gave data for a bone health study that examined the effect of isoflavones on rat bone mineral density. In this study, there were three groups. Controls received a placebo, and the other two groups received either a low or a high dose of isoflavones from kudzu. You are planning a similar study of a new kind of isoflavone. Use the results of the study described in Exercise 12.63 to plan your study. Write a proposal explaining why your study should be funded.
Question 12.83
![]() 12.83 Planning another restaurant ambiance study. Exercise 12.62 (page 693) gave data for a study that examined the effect of background music on total food spending at a high-
12.83 Planning another restaurant ambiance study. Exercise 12.62 (page 693) gave data for a study that examined the effect of background music on total food spending at a high-
12.83 The design can be similar, although the types of music might be different. Bear in mind that spending at a casual restaurant will likely be less than at the restaurants examined in Exercise 12.62; this might also mean that the standard deviations could be smaller. A pilot study might be necessary to get an idea of the size of the standard deviations. Decide how big a difference in mean spending you would want to detect; then do some power computations.