We have now come full circle in the circular flow diagram, Figure 3-1. We began by examining the supply of goods and services, and we have just discussed the demand for them. How can we be certain that all these flows balance? In other words, what ensures that the sum of consumption, investment, and government purchases equals the amount of output produced? In this classical model, the interest rate is the price that has the crucial role of equilibrating supply and demand.

70

There are two ways to think about the role of the interest rate in the economy. We can consider how the interest rate affects the supply and demand for goods or services. Or we can consider how the interest rate affects the supply and demand for loanable funds. As we will see, these two approaches are two sides of the same coin.

The following equations summarize the discussion of the demand for goods and services in Section 3-3:

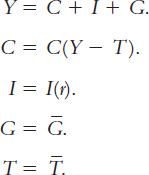
The demand for the economy’s output comes from consumption, investment, and government purchases. Consumption depends on disposable income, investment depends on the real interest rate, and government purchases and taxes are the exogenous variables set by fiscal policymakers.

To this analysis, let’s add what we learned about the supply of goods and services in Section 3-1. There we saw that the factors of production and the production function determine the quantity of output supplied to the economy:

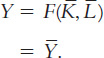
Now let’s combine these equations describing the supply and demand for output. If we substitute the consumption function and the investment function into the national income accounts identity, we obtain

Y = C(Y − T) + I(r) + G.

Because the variables G and T are fixed by policy, and the level of output Y is fixed by the factors of production and the production function, we can write

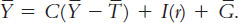
This equation states that the supply of output equals its demand, which is the sum of consumption, investment, and government purchases.

71

Notice that the interest rate r is the only variable not already determined in the last equation. This is because the interest rate still has a key role to play: it must adjust to ensure that the demand for goods equals the supply. The higher the interest rate, the lower the level of investment, and thus the lower the demand for goods and services, C + I + G. If the interest rate is too high, then investment is too low and the demand for output falls short of the supply. If the interest rate is too low, then investment is too high and the demand exceeds the supply. At the equilibrium interest rate, the demand for goods and services equals the supply.

This conclusion may seem somewhat mysterious: how does the interest rate get to the level that balances the supply and demand for goods and services? The best way to answer this question is to consider how financial markets fit into the story.

Because the interest rate is the cost of borrowing and the return to lending in financial markets, we can better understand the role of the interest rate in the economy by thinking about the financial markets. To do this, rewrite the national income accounts identity as

Y − C − G = I.

The term Y − C − G is the output that remains after the demands of consumers and the government have been satisfied; it is called national saving or simply saving (S). In this form, the national income accounts identity shows that saving equals investment.

To understand this identity more fully, we can split national saving into two parts—one part representing the saving of the private sector and the other representing the saving of the government:

S = (Y − T − C) + (T − G) = I.

The term (Y − T − C) is disposable income minus consumption, which is private saving. The term (T − G) is government revenue minus government spending, which is public saving. (If government spending exceeds government revenue, then the government runs a budget deficit and public saving is negative.) National saving is the sum of private and public saving. The circular flow diagram in Figure 3-1 reveals an interpretation of this equation: this equation states that the flows into the financial markets (private and public saving) must balance the flows out of the financial markets (investment).

To see how the interest rate brings financial markets into equilibrium, substitute the consumption function and the investment function into the national income accounts identity:

Y − C(Y − T) − G = I(r).

72

Next, note that G and T are fixed by policy and Y is fixed by the factors of production and the production function:

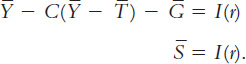
The left-hand side of this equation shows that national saving depends on income Y and the fiscal-policy variables G and T. For fixed values of Y, G, and T, national saving S is also fixed. The right-hand side of the equation shows that investment depends on the interest rate.

Figure 3-9 graphs saving and investment as a function of the interest rate. The saving function is a vertical line because in this model saving does not depend on the interest rate (we relax this assumption later). The investment function slopes downward: as the interest rate decreases, more investment projects become profitable.

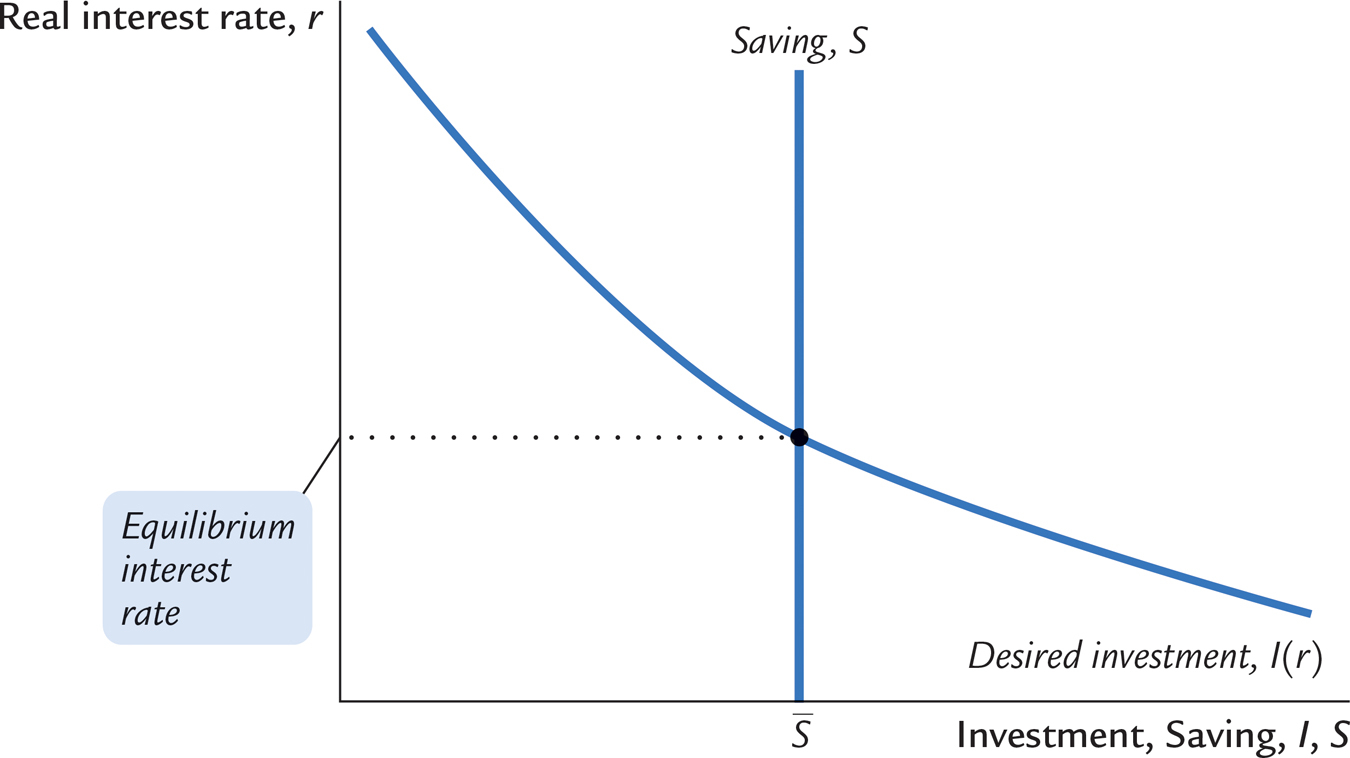
From a quick glance at Figure 3-9, one might think it was a supply-and-demand diagram for a particular good. In fact, saving and investment can be interpreted in terms of supply and demand. In this case, the “good” is loanable funds, and its “price” is the interest rate. Saving is the supply of loanable funds—households lend their saving to investors or deposit their saving in a bank that then loans the funds out. Investment is the demand for loanable funds—investors borrow from the public directly by selling bonds or indirectly by borrowing from banks. Because investment depends on the interest rate, the quantity of loanable funds demanded also depends on the interest rate.

The interest rate adjusts until the amount that firms want to invest equals the amount that households want to save. If the interest rate is too low, investors want more of the economy’s output than households want to save. Equivalently, the quantity of loanable funds demanded exceeds the quantity supplied. When this happens, the interest rate rises. Conversely, if the interest rate is too high, households want to save more than firms want to invest; because the quantity of loanable funds supplied is greater than the quantity demanded, the interest rate falls. The equilibrium interest rate is found where the two curves cross. At the equilibrium interest rate, households’ desire to save balances firms’ desire to invest, and the quantity of loanable funds supplied equals the quantity demanded.

73

We can use our model to show how fiscal policy affects the economy. When the government changes its spending or the level of taxes, it affects the demand for the economy’s output of goods and services and alters national saving, investment, and the equilibrium interest rate.

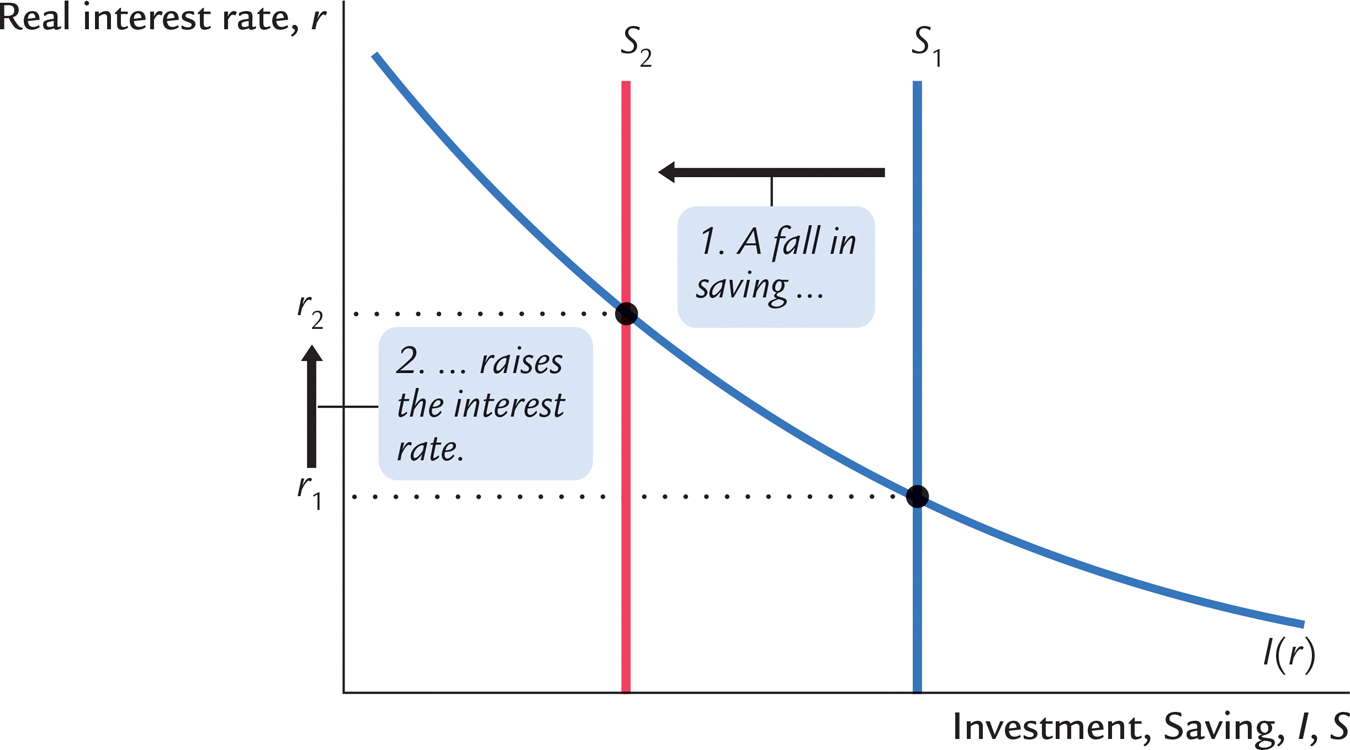
An Increase in Government Purchases Consider first the effects of an increase in government purchases by an amount ΔG. The immediate impact is to increase the demand for goods and services by ΔG. But because total output is fixed by the factors of production, the increase in government purchases must be met by a decrease in some other category of demand. Disposable income Y − T is unchanged, so consumption C is unchanged as well. Therefore, the increase in government purchases must be met by an equal decrease in investment.

To induce investment to fall, the interest rate must rise. Hence, the increase in government purchases causes the interest rate to increase and investment to decrease. Government purchases are said to crowd out investment.

To grasp the effects of an increase in government purchases, consider the impact on the market for loanable funds. Because the increase in government purchases is not accompanied by an increase in taxes, the government finances the additional spending by borrowing—that is, by reducing public saving. With private saving unchanged, this government borrowing reduces national saving. As Figure 3-10 shows, a reduction in national saving is represented by a leftward shift in the supply of loanable funds available for investment. At the initial interest rate, the demand for loanable funds exceeds the supply. The equilibrium interest rate rises to the point where the investment schedule crosses the new saving schedule. Thus, an increase in government purchases causes the interest rate to rise from r₁ to r₂.

74

A Decrease in Taxes Now consider a reduction in taxes of ΔT. The immediate impact of the tax cut is to raise disposable income and thus to raise consumption. Disposable income rises by ΔT, and consumption rises by an amount equal to ΔT times the marginal propensity to consume MPC. The higher the MPC, the greater the impact of the tax cut on consumption.

Because the economy’s output is fixed by the factors of production and the level of government purchases is fixed by the government, the increase in consumption must be met by a decrease in investment. For investment to fall, the interest rate must rise. Hence, a reduction in taxes, like an increase in government purchases, crowds out investment and raises the interest rate.

We can also analyze the effect of a tax cut by looking at saving and investment. Because the tax cut raises disposable income by ΔT, consumption goes up by MPC × ΔT. National saving S, which equals Y − C − G, falls by the same amount as consumption rises. As in Figure 3-10, the reduction in saving shifts the supply of loanable funds to the left, which increases the equilibrium interest rate and crowds out investment.

So far, we have discussed how fiscal policy can change national saving. We can also use our model to examine the other side of the market—the demand for investment. In this section we look at the causes and effects of changes in investment demand.

One reason investment demand might increase is technological innovation. Suppose, for example, that someone invents a new technology, such as the railroad or the computer. Before a firm or household can take advantage of the innovation, it must buy investment goods. The invention of the railroad had no value until railroad cars were produced and tracks were laid. The idea of the computer was not productive until computers were manufactured. Thus, technological innovation leads to an increase in investment demand.

Investment demand may also change because the government encourages or discourages investment through the tax laws. For example, suppose that the government increases personal income taxes and uses the extra revenue to provide tax cuts for those who invest in new capital. Such a change in the tax laws makes more investment projects profitable and, like a technological innovation, increases the demand for investment goods.

Figure 3-11 shows the effects of an increase in investment demand. At any given interest rate, the demand for investment goods (and also for loanable funds) is higher. This increase in demand is represented by a shift in the investment schedule to the right. The economy moves from the old equilibrium, point A, to the new equilibrium, point B.

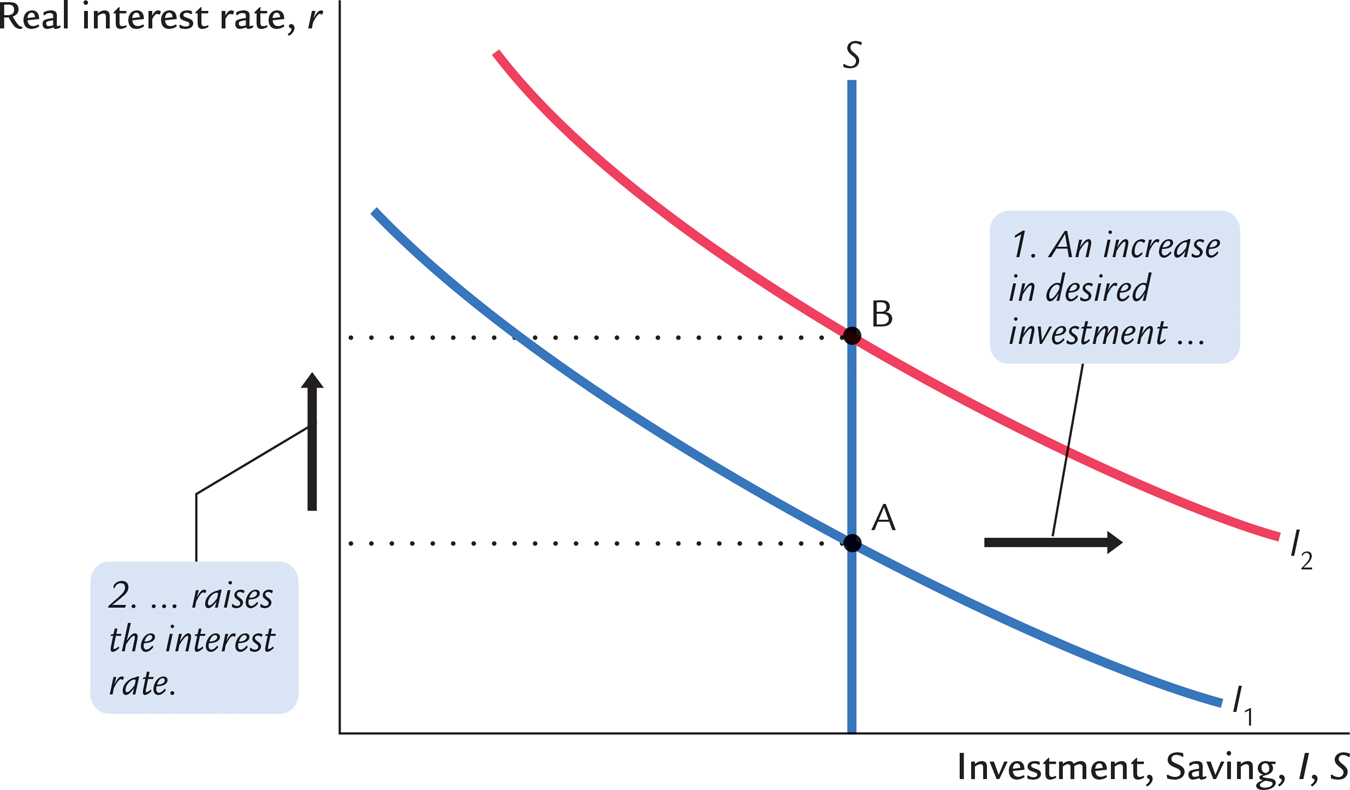
The surprising implication of Figure 3-11 is that the equilibrium amount of investment is unchanged. Under our assumptions, the fixed level of saving determines the amount of investment; in other words, there is a fixed supply of loanable funds. An increase in investment demand merely raises the equilibrium interest rate.

75

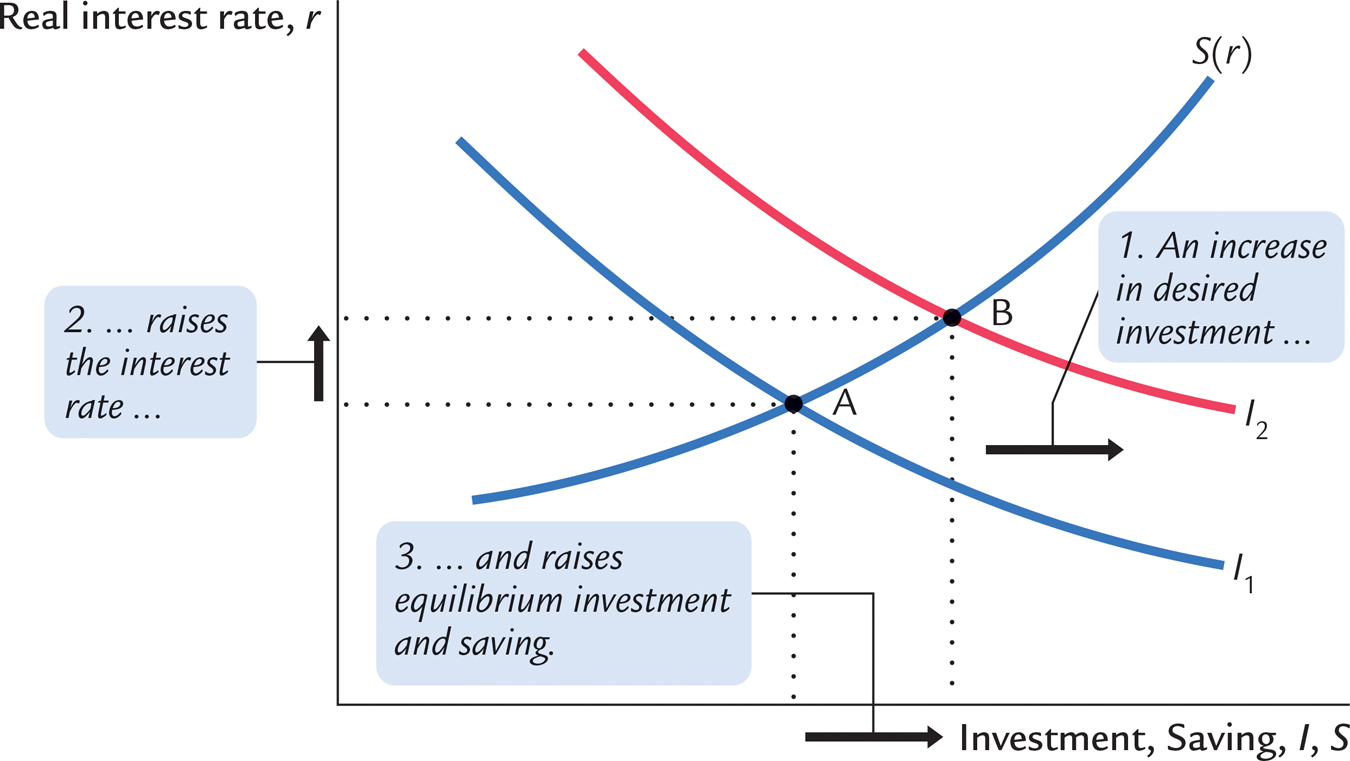
We would reach a different conclusion, however, if we modified our simple consumption function and allowed consumption (and its flip side, saving) to depend on the interest rate. Because the interest rate is the return to saving (as well as the cost of borrowing), a higher interest rate might reduce consumption and increase saving. If so, the saving schedule would be upward sloping rather than vertical.

With an upward-sloping saving schedule, an increase in investment demand would raise both the equilibrium interest rate and the equilibrium quantity of investment. Figure 3-12 shows such a change. The increase in the interest rate causes households to consume less and save more. The decrease in consumption frees resources for investment.

76