Chapter 4

Central Tendency and Variability

MPI/Getty Images

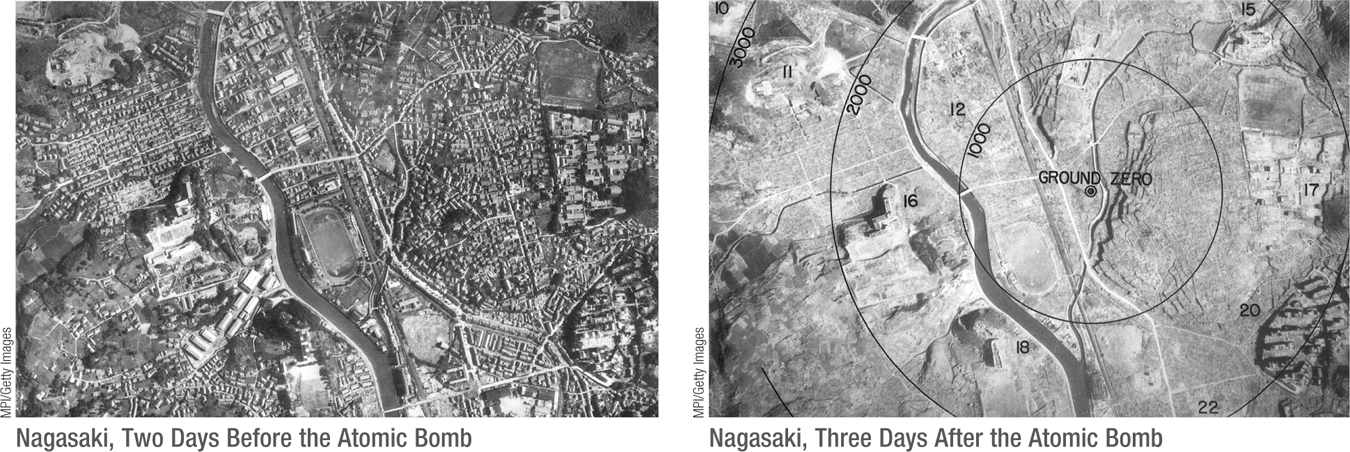
Chance variability in the cloud cover diverted a B-29 bomber from Kokura, Japan, on August 9, 1945. The World War II bomber banked instead toward its secondary target, the city of Nagasaki. When the atomic bomb exploded a few hundred feet above a tennis court, all of the buildings and most of the people who lived in the city of Nagasaki simply disappeared in less than a second. Chance was kinder to the people in Kokura; they survived. But there is even more to the story.

Five years after the bombing, an American statistician named W. Edwards Deming persuaded Japan’s leading engineers and businesspeople that a simple statistical insight could lead the devastated nation to economic recovery: Low variability means high reliability. Consumers want things that work and are willing to pay more for reliable products. For Japan, controlling variability translated into developing thousands of small manufacturing solutions that transformed Japanese products from cheap junk into merchandise of exceptional quality. Consider that this took place in a small country where the landscape, people, and morale had just been ravaged by war.

Variability is the central idea driving this powerful insight, and in this chapter, we learn about three ways to measure the variability of any observations we make: range, variance, and standard deviation. But to fully understand variability, we first have to know what observations are varying from—that is, the middle, or central tendency, of a distribution.