schacterbrief3e

16.3 Measuring Correlation

Most of us have learned from experience that memory works better when we’ve had a good night’s sleep. So if you were to predict that well-rested students will score better on a memory test than sleep-deprived students will, you will be right more often than wrong. But you won’t be right in every single instance. Some sleepy students will outscore some well-rested students. How often your prediction will be right depends on the strength of the correlation on which it is based: If the correlation between sleep and test performance is strong, then the prediction will be right almost all of the time, and if it is weak, then the prediction will be right less often. The correlation coefficient is a mathematical measure of the strength of a correlation, and it is symbolized by the letter r (as in “relationship”). The value of r can range from −1 to 1, and numbers outside that range are meaningless. What, then, do the numbers inside that range mean?

correlation coefficient

A mathematical measure of both the direction and strength of a correlation, which is symbolized by the letter r.

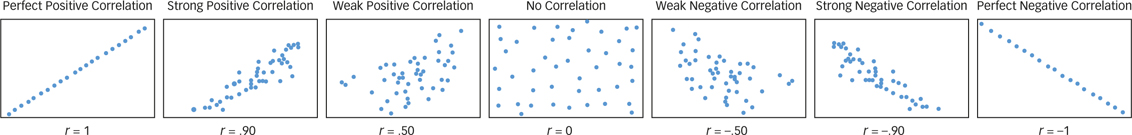
If every time the value of one variable increases by a fixed amount the value of the second variable also increases by a fixed amount, then the relationship between the variables is called a perfect positive correlation and r = 1 (see FIGURE A.6). For example, if every 30-minute increase in sleep were associated with a 10% increase in memory, then sleep and memory would be perfectly positively correlated.
If every time the value of one variable increases by a fixed amount the value of the second variable decreases by a fixed amount, then the relationship between the variables is called a perfect negative correlation and r = −1 (see FIGURE A.6). For example, if every 30-minute increase in sleep were associated with a 10% decrease in memory, then sleep and memory would be perfectly negatively correlated.
If every time the value of one variable increases by a fixed amount the value of the second variable neither increases nor decreases systematically, then the two variables are said to be uncorrelated and r = 0 (see FIGURE A.6). For example, if a 30-minute increase in sleep were sometimes associated with an increase in memory, sometimes associated with a decrease in memory, and sometimes associated with no change in memory at all, then sleep and memory would be uncorrelated.

Perfect correlations are extremely rare. In the real world, sleep and memory performance are positively correlated (i.e., as one increases, the other usually increases), but they are imperfectly correlated (i.e., every 1-minute increase in sleep does not lead to exactly one extra point on a memory test). When variables are imperfectly correlated, then the absolute value of r will lie somewhere between 0 and 1. FIGURE A.6 shows a variety of different correlations. The sign of r (plus or minus) tells us whether the correlation is positive or negative, and the absolute value of r (which varies from 0 to 1) tells us about its strength.

FIGURE A.6 Examples of Correlations This figure shows correlations of different signs and strengths. Notice how the pattern of data changes as r moves from 1 to −1.

A-5