9-2 Energy slowly moves outward from the solar interior through several processes
While thermonuclear fusion is the source of the Sun’s energy, this process cannot take place everywhere within the Sun. Extremely high temperatures—in excess of 107 K—are required for atomic nuclei to fuse together to form larger nuclei. The temperature of the Sun’s visible surface, about 5800 K, is far too low for these reactions to occur there. Hence, fusion of atoms releasing energy can be taking place only within the Sun’s interior. But precisely where does it take place? And how does the energy produced by fusion make its way to the surface, where it is emitted into space in the form of photons?
208
COSMIC CONNECTIONS The Proton-Proton Chain
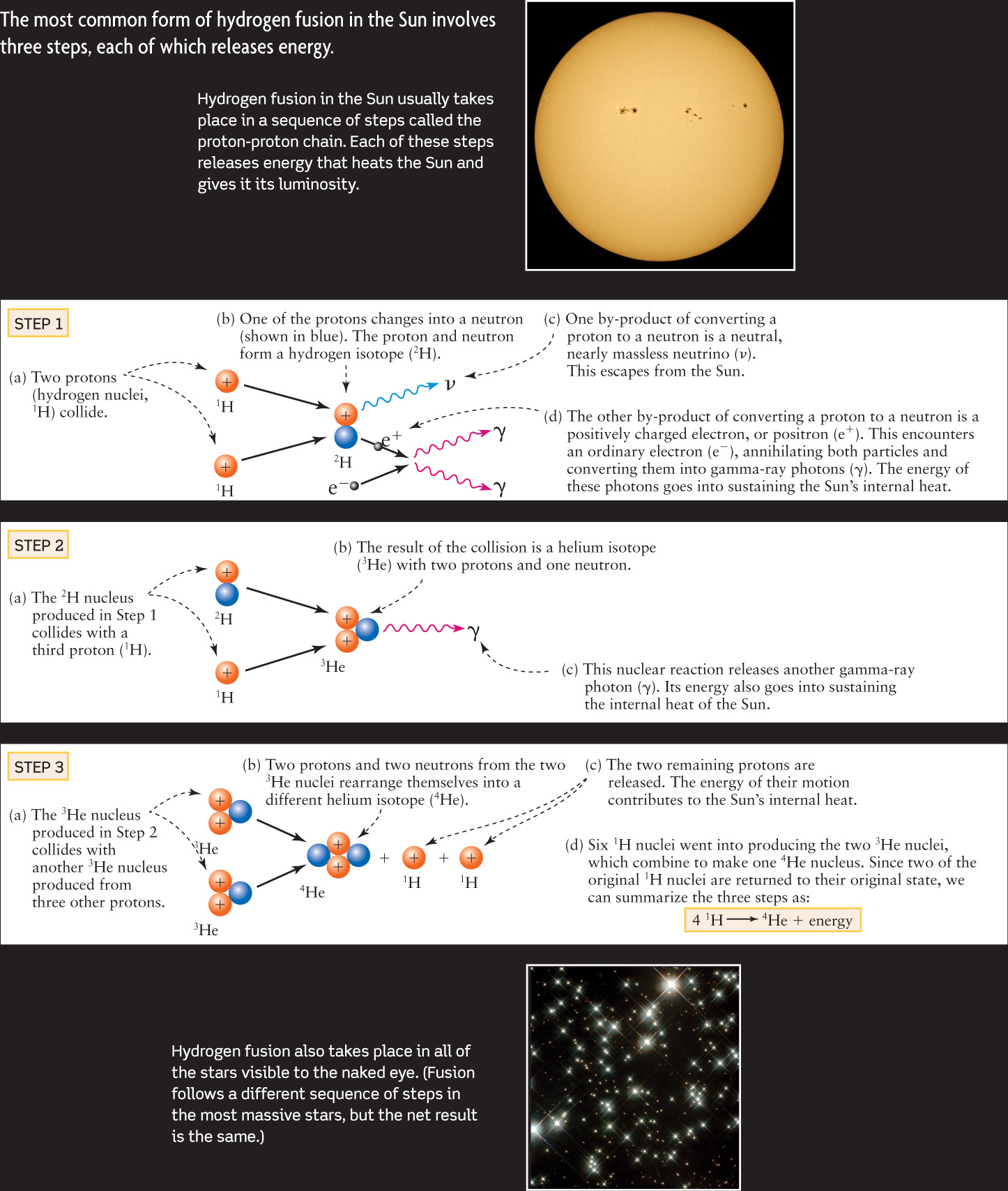
209
To answer these questions, we must understand conditions in the Sun’s interior. Ideally, we would send an exploratory spacecraft to probe deep into the Sun; in practice, the Sun’s intense heat would vaporize even the sturdiest spacecraft. Instead, astronomers use the laws of physics to construct a theoretical model of the Sun.
Hydrostatic and Thermal Equilibrium
There are several competing processes at work here. First, it is important to note that the Sun is neither growing nor shrinking, nor is it quickly becoming either hotter or cooler. To understand the nature of this stability, imagine a slab of material inside the Sun (Figure 9-1a). In equilibrium, the slab on average will move neither up nor down. (In fact, there are upward and downward motions of material inside the Sun, but these motions average out in the long run.) Balance, or equilibrium, is maintained by a balance among three competing forces that act on this imaginary slab:
- The downward force from the overlying, heavy layers of solar material above the slab due to their weight.
- The upward forces generated by expanding hot gases pushing the slab upward.
- The slab’s weight itself—that is, the downward gravitational pull it feels from the rest of the Sun.

To be stationary, the upward pushing forces from below must perfectly balance both the slab’s weight and the weight of overlying layers from above. Because the slab itself has weight, the upward forces below the slab must be greater than that above the slab for everything to be equal. In other words, force across a slab, better described as pressure, has to increase with increasing depth. For the same reason, pressure from the weight of overlying water increases as you dive deeper into the ocean (Figure 9-1b) or, in the opposite sense, atmospheric pressure decreases as one climbs up a mountain because of less overlying atmosphere pressing down on you.
In much the same way, we can make inferences about the slab’s density. If it is too dense, its weight will be too great and it will sink; if the density is too low, the slab will rise. If things are going to remain suspended with balanced forces, the density of solar material must have a certain value at each depth within the solar interior. The same principle applies to objects that float beneath the surface of the ocean. Scuba divers wear weight belts to increase their average density so that they will neither rise nor sink but will stay submerged at the same level. Astronomers refer to this balanced equilibrium state of a star, such as the Sun, as being in hydrostatic equilibrium.
Another consideration is that the Sun’s interior is so hot that it is completely gaseous. Gases compress and become more dense when you apply greater pressure to them, so density must increase along with pressure as you go to greater depths within the Sun. Furthermore, when you compress a gas, its temperature tends to rise, so the temperature must also increase as you move toward the Sun’s center.
While the temperature in the solar interior is different at different depths, the temperature at each depth remains constant in time. All the energy generated by thermonuclear reactions in the Sun’s core must be transported to the Sun’s glowing surface, where it can be radiated into space. If too much energy flowed from the core to the surface to be radiated away, the Sun’s interior would cool down; the Sun’s interior would heat up if too little energy flowed to the surface. This principle describing the Sun is called thermal equilibrium.
Question
ConceptCheck 9-5: If our Sun were much less massive and only one-half the diameter, how would the pressure at the Sun’s center be different from what it actually is?
Question
ConceptCheck 9-6: If our Sun were not in thermal equilibrium and too little energy successfully made it to the surface, how would the Sun’s core be different?
210
Transporting Energy Outward from the Sun’s Core
But exactly how is energy transported from the Sun’s center where it is produced outward to its surface? There are three methods of energy transport: conduction, convection, and radiative diffusion. Only the last two are important inside the Sun.
If you heat one end of a metal bar with a blowtorch, energy flows to the other end of the bar so that it too becomes warm. The efficiency of this method of energy transport, called conduction, varies significantly from one substance to another. For example, copper is a good conductor of heat, but wood is not—copper cooking pots often have wooden handles to keep the chef from being burned. Conduction is not an efficient means of energy transport in substances with low average densities, including the gases inside the Sun.
Inside the Sun, energy moves from center to surface by two other means: convection and radiative diffusion. Convection is the circulation of fluids—gases or liquids—between hot and cool regions. Hot gases rise toward a star’s surface, while cool gases sink back down toward the star’s center. This physical movement of gases transports heat energy outward in a star, just as the physical movement of water boiling in a pot transports energy from the bottom of the pot (where the heat is applied) to the cooler water at the surface (see Figure 5-6).
In radiative diffusion, photons emitted from the thermonuclear inferno at a star’s center spread outward toward the star’s surface. Individual photons are absorbed and reemitted by atoms and electrons inside the star. The overall result is a general outward migration from the hot core, where photons are constantly created, toward the cooler surface, where they escape into space.
Question
ConceptCheck 9-7: Why is the energy transport process of conduction relatively unimportant when studying how energy moves toward the Sun’s surface?
Modeling the Sun
To construct a model of a star like the Sun, astronomers express the ideas of hydrostatic equilibrium, thermal equilibrium, and energy transport as a set of equations, most often done using the metric system of measurement. To ensure that the model applies to the particular star under study, they also make use of astronomical observations of the star’s surface. (For example, to construct a model of the Sun, they use the data that the Sun’s surface temperature is 5800 K, its luminosity is 3.9 × 1026 W, and the gas pressure and density at the surface are almost zero.) The astrophysicists then use a computer to solve their set of equations and calculate conditions layer by layer in toward the star’s center. The result is a model of how temperature, pressure, and density increase with increasing depth below the star’s surface.
Table 9-1 and Figure 9-2 show a theoretical model of the Sun that was calculated in just this way. Different models of the Sun use slightly different assumptions, but all models give essentially the same results as those shown here. From such computer models we have learned that at the Sun’s center the density is 160,000 kg/m3 (14 times the density of lead!), the temperature is 1.55 × 107 K, and the pressure is 3.4 × 1011 atm. (One atmosphere, or 1 atm, is the average atmospheric pressure at sea level on Earth.)
| Distance from the Sun’s center (solar radii) | Fraction of luminosity | Fraction of mass | Temperature (× 106°F) | Density (kg/m3) | Pressure relative to pressure at center |
|---|---|---|---|---|---|
| 0.0 | 0.00 | 0.00 | 27.9 | 160000 | 1 |
| 0.1 | 0.42 | 0.07 | 23.4 | 90000 | 0.46 |
| 0.2 | 0.94 | 0.35 | 17.1 | 40000 | 0.15 |
| 0.3 | 1.00 | 0.64 | 12 | 13000 | 0.04 |
| 0.4 | 1.00 | 0.85 | 8.6 | 4000 | 0.007 |
| 0.5 | 1.00 | 0.94 | 6.1 | 1000 | 0.001 |
| 0.6 | 1.00 | 0.98 | 3.9 | 400 | 0.0003 |
| 0.7 | 1.00 | 0.99 | 2.2 | 80 | 4 × 10−5 |
| 0.8 | 1.00 | 1.00 | 1.3 | 20 | 4 × 10−6 |
| 0.9 | 1.00 | 1.00 | 0.5 | 2 | 3 × 10−7 |
| 1.0 | 1.00 | 1.00 | 0.01 | 0.00030 | 4 × 10−13 |
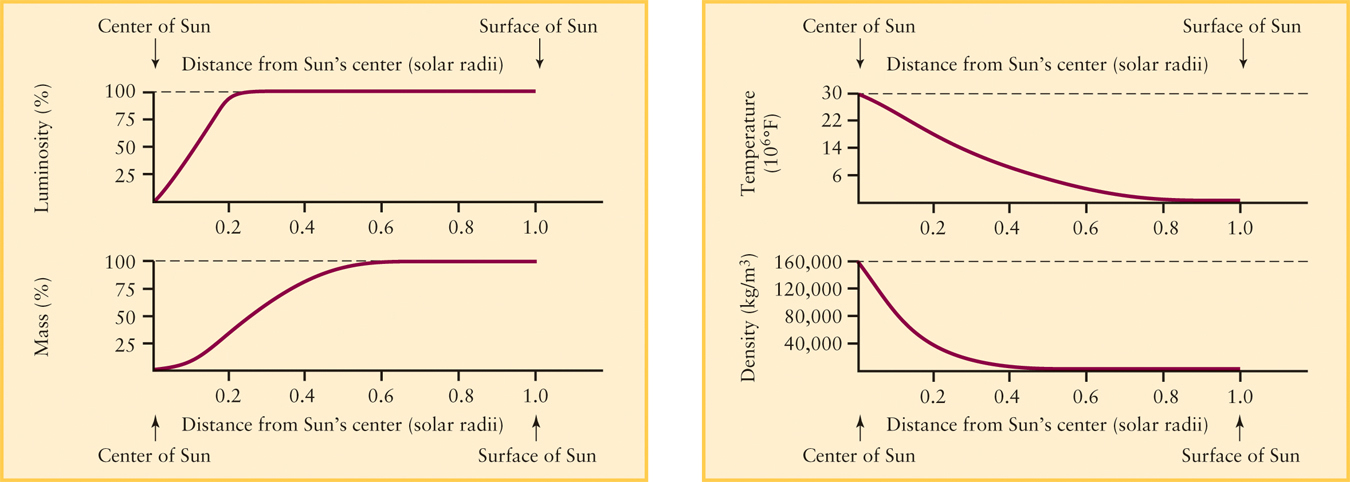
Table 9-1 and Figure 9-2 show that the solar luminosity rises to 100% at about one-quarter of the way from the Sun’s center to its surface. In other words, the Sun’s energy production occurs within a volume that extends out only to 0.25 R⊙. (The symbol R⊙ denotes the solar radius, or radius of the Sun as a whole, equal to 696,000 km.) Outside 0.25 R⊙, the density and temperature are too low for thermonuclear reactions to take place. Also note that 94% of the total mass of the Sun is found within the inner 0.5 R⊙. Hence, the outer 0.5 R⊙ contains only a relatively small amount of material.
211
Precisely how energy flows from the Sun’s center outward depends on how easily photons move through the gas. If the solar gases are comparatively transparent, photons can travel moderate distances before being scattered or absorbed, and energy is thus transported by radiative diffusion. If the gases are comparatively opaque, photons cannot get through the gas easily and heat builds up. Convection then becomes the most efficient means of energy transport. The gases start to churn, with hot gas moving upward and cooler gas sinking downward.
From the center of the Sun out to about 0.71 R⊙, energy is transported by radiative diffusion. Hence, this region is called the radiative zone. Beyond about 0.71 R⊙, the temperature is low enough (a mere 2 × 106 K or so) for electrons and hydrogen nuclei to join into hydrogen atoms. These atoms are very effective at absorbing photons, much more so than at absorbing free electrons or nuclei, and this absorption chokes off the outward flow of photons. Therefore, beyond about 0.71 R⊙, radiative diffusion is not an effective way to transport energy. Instead, convection dominates the energy flow in this outer region, which is why it is called the convective zone. Figure 9-3 shows these aspects of the Sun’s internal structure.
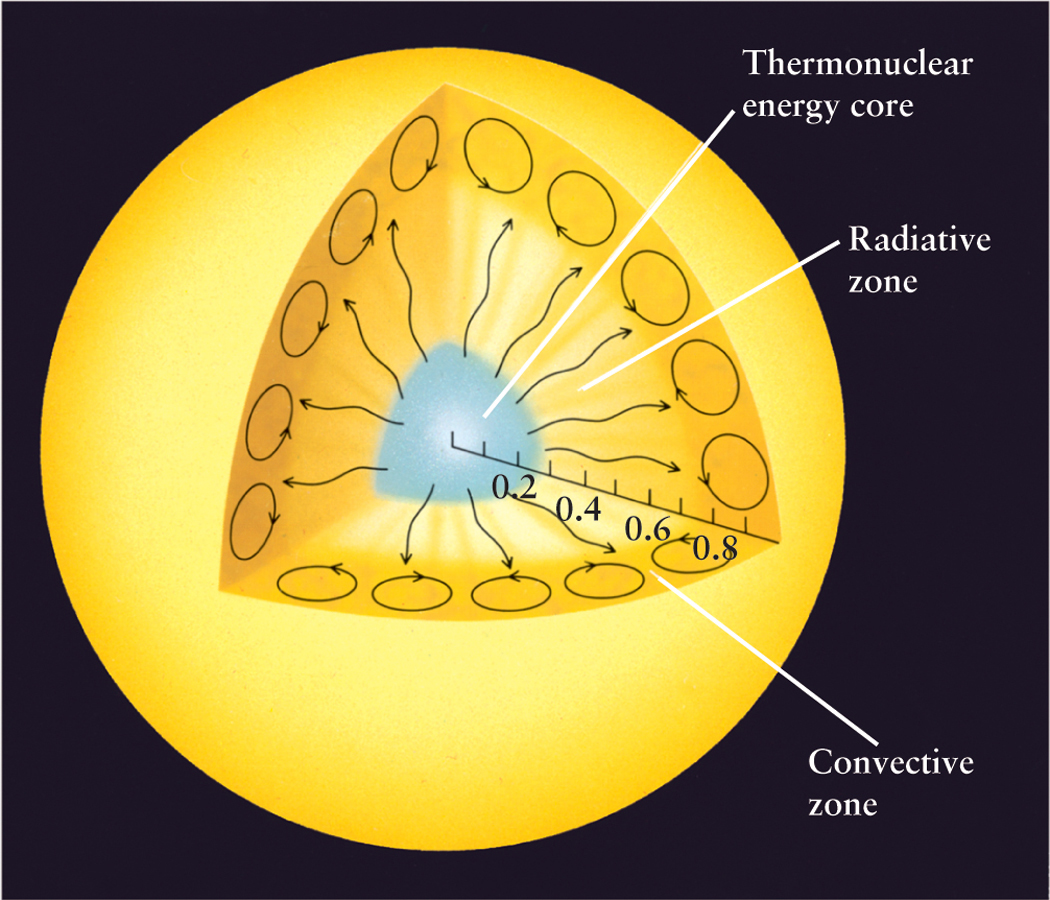
Although energy travels through the radiative zone in the form of photons, the photons have a difficult time of it. As shown in Table 9-1, the material in this zone is extremely dense, so photons from the Sun’s core take a long time to diffuse through the radiative zone. As a result, it takes approximately 170,000 years for energy created at the Sun’s center to travel 696,000 km to the solar surface and finally escape as sunlight. The energy flows outward at an average rate of 50 cm per hour, or about 20 times slower than a snail’s pace.
Once the energy escapes from the Sun, it travels much faster—at the speed of light. Thus, solar energy that reaches you today took only 8 minutes to travel the 150 million km from the Sun’s surface to Earth. But this energy was actually produced by thermonuclear reactions that took place in the Sun’s core hundreds of thousands of years ago.
Question
ConceptCheck 9-8: Which of the following decreases when we move from the Sun’s central core outward: temperature, mass, or luminosity?
Question
CalculationCheck 9-2: By what percentage does the Sun’s temperature drop from its central core temperature moving out to a distance of one-half its radius?
212
Probing the Sun’s Interior
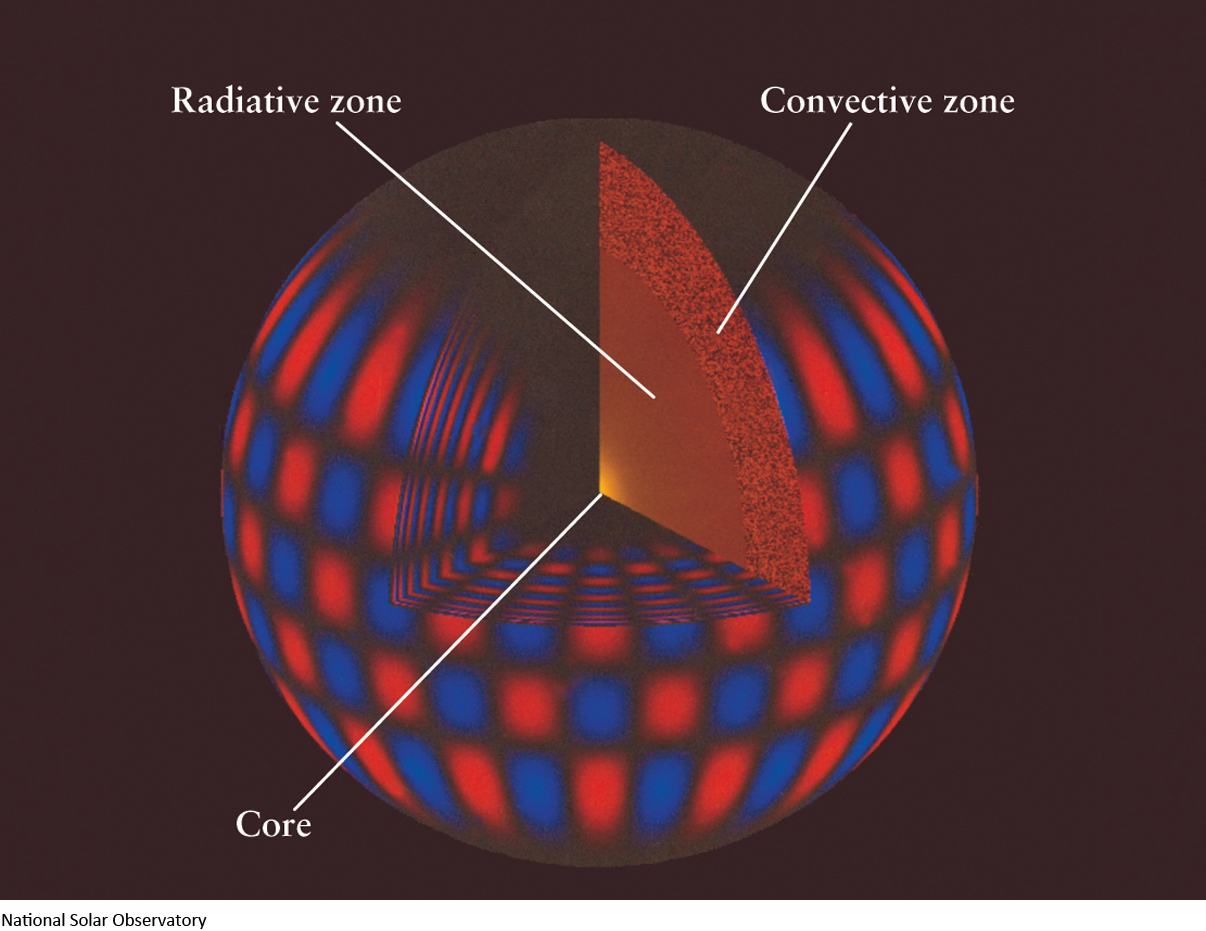
If the Sun’s interior is not visible from the surface, how might you go about figuring out what is inside? For that matter, how might you determine if a melon is ripe at your local grocery store without cutting it open? Vibrations are a useful tool for examining the hidden interiors of all kinds of objects. Much like food shoppers who tap melons to listen for particular vibrations and much like geologists who determine the structure of Earth’s interior by using seismographs to record vibrations during earthquakes, one powerful technique to infer what is going on beneath the Sun’s surface involves measuring vibrations of the Sun as a whole. This field of solar research is called helioseismology.
The Sun oscillates in millions of ways as a result of waves resonating in its interior. Figure 9-4 is a computer-generated illustration of one such mode of vibration. Helioseismologists can deduce information about the solar interior from measurements of these oscillations. For example, they have been able to set limits on the amount of helium in the Sun’s core and convective zone and to determine the thickness of the transition region between the radiative zone and convective zone. They have also found that the convective zone is thicker than previously thought.
Another approach is to carefully measure everything that comes out of the Sun and then determine how it must have been formed. As part of the process of thermonuclear fusion, protons change into neutrons and release particles called neutrinos. Like photons, neutrinos are particles that have no electric charge. Unlike photons, however, neutrinos interact only very weakly with matter and are highly difficult to capture. Even the vast bulk of the Sun offers little impediment to the passage of neutrinos, so neutrinos must be streaming out of the core and into space. Indeed, the conversion of hydrogen into helium at the Sun’s center produces 1038 neutrinos each second. Every second, about 1014 neutrinos created within the Sun must pass through each square meter of Earth. The challenge is that neutrinos are exceedingly difficult to detect. Just as neutrinos pass unimpeded through the Sun, they also pass through Earth almost as if it were not there. When we are careful about how we capture neutrinos, we are able to confirm that the Sun’s energy is indeed caused by thermonuclear reactions just like our computer models tell us.
Question
ConceptCheck 9-9: What can be determined from carefully monitoring the Sun’s vibrations?