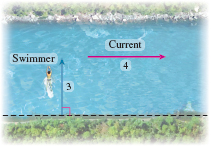
Graphing a Resultant Vector

A swimmer swims at a constant speed of 3 miles per hour (mi/h) and heads directly across a river whose current is 4 mi/h.

1. Draw vectors representing the swimmer and the current.
2. Draw the resultant vector.
3. Interpret the result.

Solution (a) We represent the swimmer by a vector pointing directly across the river and the current by a vector pointing parallel to a straight shore line. Since the swimmer’s speed is \(3 \,\rm{mi/h}\), and the current’s speed is \(4 \,\rm{mi/h}\), the length of the vector representing the swimmer is \(3\) units, and the length of the vector representing the current is \(4\) units. See Figure 16.

(b) The resultant vector is the sum of the vectors in (a). See Figure 17.

(c) The resultant vector shows the true speed of the swimmer and true direction of the swim. In fact, the true speed is \(5 \,\rm{mi/h}\) and the true direction can be described in terms of the angle \(\theta \) as \( \theta =\tan ^{-1}\dfrac{4}{3}\approx 53.1^{\circ}.\)