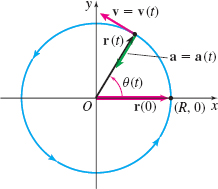
EXAMPLE 3Finding the Position, Velocity, and Acceleration of a Particle Moving Along a Circle
(a) Find the position r=r(t) of a particle that moves counterclockwise along a circle of radius R with a constant speed v0.
(b) Find the velocity and acceleration of the particle.
(c) Find the magnitude of the acceleration.
Solution For convenience, we place the circle of radius R in the xy-plane, with its center at the origin, and assume that at time t=0 the particle is on the positive x-axis.
(a) The particle is moving counterclockwise along the circle, as shown in Figure 28. If θ(t) is the angle between the positive x-axis and the position vector of the particle r= r(t), then the vector r=r(t) is r(t)=Rcos[θ(t)]i+Rsin[θ(t)]j
787
Notice that r(0)=Ri, as required. Also for the motion to be counterclockwise, the function θ=θ(t) must be increasing.
(b) The velocity v of the particle is v(t)=drdt=−Rsin[θ(t)]dθdti+Rcos[θ(t)]dθdtjUse the Chain Rule.
and the speed v of the particle is v(t)=‖
Since we know the speed is constant, v(t)=v_{0}. Also, since the function \theta\;=\;\theta ( t) is increasing, \dfrac{d\theta }{dt}>0. As a result, \begin{eqnarray*} v_{0}&=&R\frac{d\theta }{dt} \\[4pt] \frac{d\theta }{dt}&=&\frac{v_{0}}{R} \end{eqnarray*}
Since \dfrac{d\theta }{dt} is the rate at which the angle \theta is changing, the quantity \dfrac{v_{0}}{R} is the angular speed \omega of the particle. That is, \begin{equation*} \frac{d\theta }{dt}=\omega \end{equation*}
We solve this differential equation, using \theta ( 0)\;=\;0 as the initial condition. \begin{eqnarray*} d\theta &=&\omega dt \\[3pt] \theta ( t) &=&\omega t+k \\[3pt] \theta ( 0) &=&k=0 \end{eqnarray*}
So, \theta (t)=\omega t. Now substitute \theta (t)=\omega t into the vector function \mathbf{r=r}( t) [statement (1)] to obtain \begin{equation*} \mathbf{r}(t)=R\;\cos ( \omega t) \mathbf{i}+R\;\sin ( \omega t) \mathbf{j} \end{equation*}
Then the velocity \mathbf{v} and acceleration \mathbf{a} of the particle are \begin{eqnarray*} \mathbf{v}\;=\;\mathbf{v}(t) &=& -R\omega \sin ( \omega t) \mathbf{i} +R\omega \cos ( \omega t) \mathbf{j} \\[3pt] \mathbf{a}\;=\;\mathbf{a}(t) &=& -R\omega ^{2}\cos ( \omega t) \mathbf{i }-R\omega ^{2}\sin ( \omega t) \mathbf{j}=-\omega ^{2}\left[ R\;\cos ( \omega t) \mathbf{i}+R\;\sin ( \omega t) \mathbf{ j}\right]\\[3pt] &=&-\omega ^{2}\mathbf{r}(t) \end{eqnarray*}
(c) Since \omega=\dfrac{v_{0}}{R}, the magnitude of the acceleration is \begin{equation*} \bbox[5px, border:1px solid black, #F9F7ED] {\left\Vert \mathbf{a}(t)\right\Vert\;=\;\omega ^{2}\left\Vert \mathbf{r}(t)\right\Vert\;=\;\omega ^{2}R=\dfrac{v_{0}^{2}}{R}}\tag{2} \end{equation*}