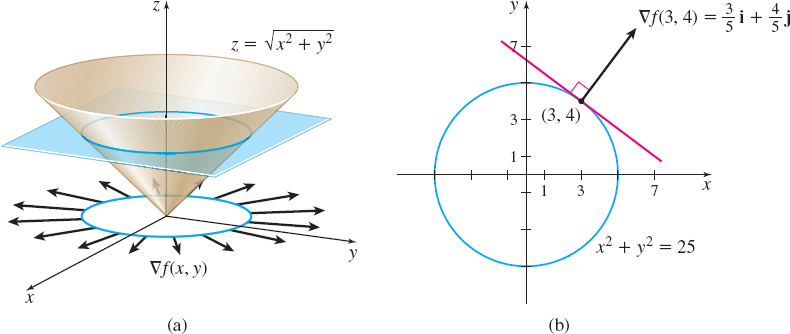
Using Properties of the Gradient

For the function \(f(x,y)=\sqrt{x^{2}+y^{2}}\), graph the level curve containing the point \((3,4)\) and graph the gradient \({\bf\nabla\! }f(x,y)\) at this point.

Solution See Figure 8(a). The graph of the equation \(z=\sqrt{x^{2}+y^{2}}\) is the upper half of a circular cone whose traces are circles. So, the level curves are concentric circles centered at \(( 0,0)\). Because \(f(3,4)=\sqrt{9+16}=5\), the level curve through \((3,4)\) is the circle \(x^{2}+y^{2}=25\). Since \[ \begin{equation*} {\bf\nabla}\! f(x,y)=\frac{x}{\sqrt{x^{2}+y^{2}}}\mathbf{i}+\frac{y}{\sqrt{ x^{2}+y^{2}}}\mathbf{j} \end{equation*} \]

the gradient at \((3,4)\) is \[ \begin{equation*} {\bf\nabla}\! f(3,4)=\frac{3}{5}\mathbf{i}+\frac{4}{5}\mathbf{j} \end{equation*} \]

This vector is orthogonal to the level curve \(x^{2}+y^{2}=25\), as shown in Figure 8(b).