EXAMPLE 1Finding an Equation of a Tangent Plane to a Surface
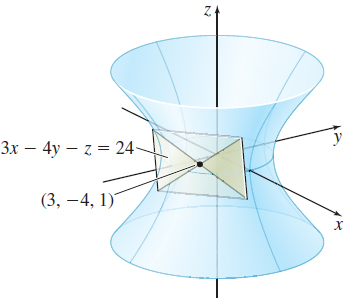
Figure 11 x2+y2−z2=24
Find an equation of the tangent plane to the hyperboloid of one sheet x2+y2−z2=24 at the point (3,−4,1).
Solution The surface is given by the function F(x,y,z)=x2+y2−z2−24=0. The partial derivatives of F are Fx(x,y,z)=2xFy(x,y,z)=2yFz(x,y,z)=−2z
At the point (3,−4,1), the partial derivatives are Fx(3,−4,1)=6Fy(3,−4,1)=−8Fz(3,−4,1)=−2
An equation of the tangent plane at (3,−4,1) is 6(x−3)−8(y+4)−2(z−1)=03x−4y−z=24