Find all local maxima, local minima, and saddle points for $$z=f(x,y)=x^{2}+xy+y^{2}-6x+6$$

Solution  The critical points of $f$ are solutions of the system of equations $$\left\{ \begin{array}{rcll} f_{x}(x,y)&=&2x+y-6&=0 \\[3pt] f_{y}(x,y)&=&x+2y &=0 \end{array} \right.$$

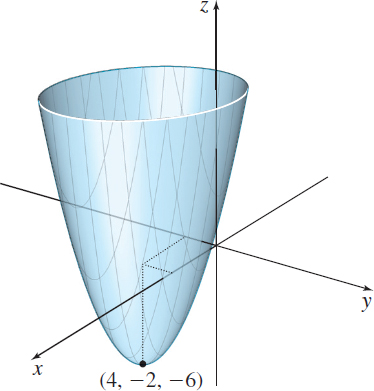
Figure 16 $$\begin{array}{l} z=f(x,y)\\ \,\,\,\, =x^{2}+xy+y^{2}-6x+6 \\ \end{array}$$

The only solution is $x=4$ and $y=-2,$ so $(4,-2)$ is the only critical point.

The second-order partial derivatives of $f$ are $$f_{xx}(x,y)=2\qquad f_{xy}(x,y)=f_{yx}( x,y) =1 \qquad f_{yy}(x,y)=2$$

The function $f$ meets the requirements of the Second Partial Derivative test at the critical point $(4,-2)$. Now $$A=f_{xx}(4,-2)=2\qquad B=f_{xy}(4,-2)=1 \qquad C=f_{yy}(4,-2)=2$$ $$AC-B^{2}=( 2) ( 2) -1^{2}=3>0$$

Since $AC-B^{2}>0$ and $A= f_{xx}(4,-2)=2>0,$ the Second Partial Derivative Test guarantees that $f$ has a local minimum at $(4,-2)$. The local minimum value is $z=f(4,-2)=-6$. See Figure 16.