Finding Surface Area

Find the surface area of the part of the paraboloid \(z=f(x,y)=1-x^{2}-y^{2}\) that lies above the \(xy\)-plane.

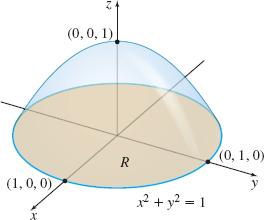
Solution Figure 39 shows the part of the surface \(z=1-x^{2}-y^{2}\) that lies above the \(xy\)-plane and its projection onto the \(xy\)-plane, the region \(R\) enclosed by the circle \(x^{2}+y^{2}=1\). We begin by finding the partial derivatives of \(z=f(x,y)\). \[ f_{x}(x,y)=-2x\qquad f_{y}(x,y)=-2y \]

Since \(f_x\) and \(f_y\) are continuous on \(R\), the surface area \(S\) of the part of \(z=f(x,y) =1-x^{2}-y^{2}\) that lies above \(R\) is \begin{eqnarray*} S&=&\displaystyle\iint\limits_{\kern-3ptR}\sqrt{[f_{x}(x,y)]^{2}+[f_{y} (x,y)]^{2} +1} \,{\it dA}=\displaystyle\iint\limits_{\kern-3ptR}\sqrt{ (-2x)^{2}+(-2y)^{2}+1}\,{\it dA}\\ &=&\displaystyle\iint\limits_{\kern-3ptR}\sqrt{4(x^{2}+y^{2})+1} \,{\it dA} \end{eqnarray*}

Since both the region \(R\) (a circle) and the integrand involve \(x^{2}+y^{2}\) , we use polar coordinates \((r,\theta)\). For the region \(R,\) we have \(0\leq r\leq 1\) and \(0\leq \theta \leq 2\pi\). Then \[ \begin{equation*} \begin{array}{rcl} S& =&\displaystyle\iint\limits_{\kern-3ptR}\sqrt{4(x^{2}+y^{2})+1}\,d\!A\underset{\underset{\underset{{\color{#0066A7}{\hbox {\({dx}\, {dy}=r\,{dr} d\theta\)}}}} {\color{#0066A7}{\hbox{\(x^{2}+y^{2}=r^{2}\)}}}} {\color{#0066A7}{\uparrow}}}{=} \int_{0}^{2\pi }\int_{0}^{1}\sqrt{4r^{2}+1}r\,dr\,d\theta \notag \\ &=&\int_{0}^{2\pi }\dfrac{1}{12}\big[ ( 4r^{2}+1) ^{3/2}\big] _{0}^{1}\,d\theta =\dfrac{1}{12}(5\sqrt{5}-1)\int_{0}^{2\pi }\,d\theta = \dfrac{1}{12}(5\sqrt{5}-1)2\pi\\[9pt] &=&\dfrac{\pi }{6}(5\sqrt{5}-1) \end{array} \end{equation*} \]