Describing a Parametric Surface

Describe the parametric surface \(S\) parametrized by \[ \begin{equation*} \mathbf{r}(u,v) =( 3+\cos v) \cos u\,\mathbf{i} +( 3+\cos v) \sin u\,\mathbf{j}+\sin v\mathbf{k} \end{equation*} \]

where \(0\leq u\leq 2\pi \) and \(0\leq v\leq 2\pi .\)

Solution We begin by finding some \(u\)-coordinate curves. Since the parametrization involves trigonometric functions, we choose \(0,\dfrac{\pi }{2},\pi ,\) and \(\dfrac{3\pi }{2}.\) Then the \(u\)-coordinate curves are \[ \begin{equation*} \begin{array}{rcl@{\qquad}rrcl} \mathbf{r}(u,0) &=&4\cos u\,\mathbf{i}+4\sin u\,\mathbf{j} & \mathbf{r}\left( u,\dfrac{\pi }{2}\right) &=&3\cos u\,\mathbf{i}+3\sin u\,\mathbf{j+k}\\[5pt] \mathbf{r}( u,\pi ) &=&2\cos u\,\mathbf{i}+2\sin u\,\mathbf{j} & \mathbf{r}\left( u,\dfrac{3\pi }{2}\right) &=&3\cos u\,\mathbf{i}+3\sin u\,\mathbf{j-k} \end{array} \end{equation*} \]

We identify the graphs of each of these curves by using parametric equations. For example, for \(\mathbf{r}(u,0) \), we have \(x=4\cos u,\) \(y=4\sin u,\) and \[ x^{2}+y^{2}=( 4\cos u) ^{2}+( 4\sin u)^{2}=4^{2}( \cos ^{2}u+\sin ^{2}u) =4^{2} \]

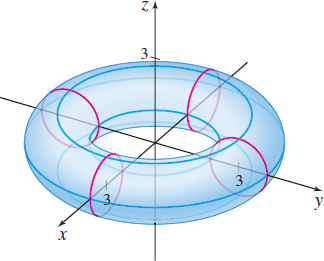
a circle of radius \(4\) centered at the origin. Similarly, \(\mathbf{r=r} ( u,\pi ) \) is a circle of radius \(2\) centered at the origin. The graphs of \(\mathbf{r}\left( u,\dfrac{\pi }{2}\right) \) and \(\mathbf{r}\left( u,\dfrac{3\pi }{2}\right) \) are both circles of radius \(3,\) but the center of \(\mathbf{r=r}\left( u,\dfrac{\pi }{2}\right) \) is at \(( 0,0,1)\) and the center of \(\mathbf{r=r}\left( u,\dfrac{3\pi }{2}\right) \) is at \(( 0,0,-1) .\) Do you see why? See the blue circles in Figure 42.

Using \(0,\dfrac{\pi }{2},\pi ,\) and \(\dfrac{3\pi }{2}\) for \(u,\) we obtain the \(v\)-coordinate curves: \[ \begin{array}{rcl@{\qquad}llcl} \mathbf{r}( 0,v) &=&( 3+\cos v) \mathbf{i}+\sin v\,\mathbf{k} & \mathbf{r}\left( \dfrac{\pi }{2},v\right) =( 3+\cos v) \,\mathbf{j}+\sin v\,\mathbf{k} \\[5pt] \mathbf{r}( \pi ,v) &=&-( 3+\cos v) \,\mathbf{i}+\sin v\, \mathbf{k} & \mathbf{r}\left( \dfrac{3\pi }{2},v\right) =-( 3+\cos v) \,\mathbf{j}+\sin v\,\mathbf{k} \end{array} \]

Again, we identify the graphs of these curves as circles. Each graph is a circle of radius \(1,\) but the centers of the circles \(\mathbf{r=r}(0,v) ,\) \(\mathbf{r=r}( \pi ,v) ,\) \(\mathbf{r=r}\left( \dfrac{\pi }{2},v\right)\), and \(\mathbf{r=r}\left( \dfrac{3\pi }{2},v\right) \) are \(( 3,0,0) \), \(( -3,0,0) ,\) \((0,3,0) ,\) and \(( 0,-3,0) ,\) respectively. See the red circles in Figure 42.

The surface \(S\) is a torus. This particular torus results from revolving a circle of radius \(1\) about a circle of radius \(3\) centered at the origin.