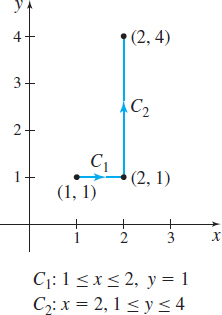$\mathbf{F}=\mathbf{F}(x,y)=(2xy+24x)\mathbf{i}+(x^{2}+16)\mathbf{j}$ is a conservative vector field, since $\mathbf{F}$ is the gradient of $f(x,y)=x^{2}y+12x^{2}+16y$. Use this fact to find $$\int_{C}\left[ (2xy+24x)\,dx+(x^{2}+16)\,dy\right]$$

where $C$ is any piecewise-smooth curve joining the points $(1,1)$ and $(2,4)$.

Solution We use two methods to find $\int_{C}[(2xy+24x)\,dx+(x^{2}+16)\,dy]$.

Method I uses the potential function $f(x,y)=x^{2}y+12x^{2}+16y$ whose gradient is $$\nabla\! \ f=(2xy+24x)\mathbf{i}+(x^{2}+16)\mathbf{j}=\mathbf{F}(x,y)$$

and the Fundamental Theorem of Line Integrals. $$\begin{eqnarray*} \hspace{-250pt}\int_{C}[(2xy+24x)\,dx+(x^{2}+16)\,dy]=\big[ f(x,y)\big]_{(1,1)}^{(2,4)}=f(2,4)-f(1,1)\\[4pt] \hspace{0pt}= [ ( 2^{2}) ( 4) +12(2^{2}) +16( 4) ] -[ ( 1^{2}) (1) +12( 1^{2}) +16( 1) ] =99 \end{eqnarray*}$$

Figure 23

Method II uses the fact that the given line integral is independent of the path, so it can be integrated along any piecewise-smooth curve joining $(1,1)$ and $(2,4)$. We choose the path shown in Figure 23 since it makes the integration easy. So, along $C_{1},$ $dy=0$,

and along $C_{2},$ $dx=0.$ Then $$\begin{eqnarray*} \hspace{-1.0pc}\int_{C}[(2xy+24x)\,dx+(x^{2}+16)\,dy]&=&\int_{C_{1}}[(2xy+24x)\,dx+(x^{2}+16)\,dy]\\[4pt] &&+\int_{C_{2}}[(2xy+24x)\,dx+(x^{2}+16)\,dy] \notag \\[4pt] &=& \int_{1}^{2}(2x+24x)\,dx+\int_{1}^{4}(4+16)\,dy=39+60=99 \end{eqnarray*}$$