Finding the Values of an Inverse Sine Function

Find the exact value of:

  1. \(\sin ^{-1}\dfrac{\sqrt{3}}{2}\)
  2. \(\sin ^{-1}\left( - \dfrac{\sqrt{2}}{2}\right)\)
  3. \(\sin ^{-1}\left( \sin \dfrac{ \pi }{8} \right)\)
  4. \(\sin ^{-1}\left( \sin \dfrac{5\pi }{8 } \right)\)

Solution (a) We seek an angle whose sine is \(\dfrac{\sqrt{3}}{2} .\) Since \(-1{<}\dfrac{\sqrt{3}}{2}{<}1,\) let \(y=\sin ^{-1}\dfrac{\sqrt{3}}{2}\). Then by definition, \(\sin y=\dfrac{\sqrt{3}}{2}\), where \(-\dfrac{\pi }{2} \leq y\leq \dfrac{\pi }{2}\). Although \(\sin y=\dfrac{\sqrt{3}}{2}\) has infinitely many solutions, the only number in the interval \(\left[ -\dfrac{ \pi }{2},\dfrac{\pi }{2}\right] \), for which \(\sin y=\dfrac{\sqrt{3}}{2}\), is \(\dfrac{\pi }{3}.\) So \(\sin ^{-1}\dfrac{\sqrt{3}}{2}=\dfrac{\pi }{3}\).

(b) We seek an angle whose sine is \(-\dfrac{\sqrt{2}}{2}.\) Since \( -1<-\dfrac{\sqrt{2}}{2}<1\), let \(y=\sin ^{-1}\left( -\dfrac{\sqrt{2}}{2}\right)\). Then by definition, \(\sin y=-\dfrac{\sqrt{2}}{2}\), where \(-\dfrac{\pi }{2}\leq y\leq \dfrac{\pi }{2}\). The only number in the interval \( \left[ -\dfrac{\pi }{2},\dfrac{\pi }{2}\right] ,\) whose sine is \(-\dfrac{ \sqrt{2}}{2},\) is \(-\dfrac{\pi }{4}\). So \(\sin ^{-1}\left( -\dfrac{\sqrt{2}}{ 2}\right) =-\dfrac{\pi }{4}\).

(c) Since the number \(\dfrac{\pi }{8}\) is in the interval \(\left[ -\dfrac{\pi }{2},\dfrac{\pi }{2}\right]\), we use (1). \[ \sin ^{-1}\left( \sin \dfrac{\pi }{8}\right) =\dfrac{\pi }{8} \]

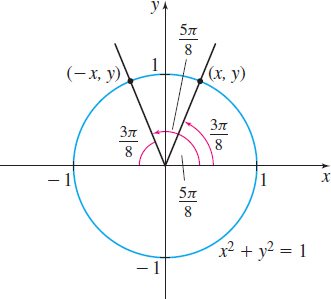
(d) Since the number \(\dfrac{5\pi }{8}\) is not in the closed interval \(\left[ -\dfrac{\pi }{2},\dfrac{\pi }{2}\right] ,\) we cannot use (1). Instead, we find a number \(x\) in the interval \(\left[ -\dfrac{\pi }{2} ,\dfrac{\pi }{2}\right] \) for which \(\sin x=\sin \dfrac{5\pi }{8}.\) Using Figure 84, we see that \(\sin \dfrac{5\pi }{8}=y=\sin \dfrac{3\pi }{8}.\) The number \(\dfrac{3\pi }{8}\) is in the interval \( \left[ -\dfrac{\pi }{2},\dfrac{\pi }{2}\right]\), so \[ \sin ^{-1}\left( \sin \dfrac{5\pi }{8}\right) =\sin ^{-1}\left( \sin \dfrac{ 3\pi }{8}\right) =\dfrac{3\pi }{8} \]