Solving Trigonometric Equations

Solve the equations:

1. \(\sin \theta =\dfrac{1}{2}\)
2. \(\cos \theta =0.4\)

Give a general formula for all the solutions and list all the solutions in the interval \([ -2\pi ,2\pi ] .\)

62

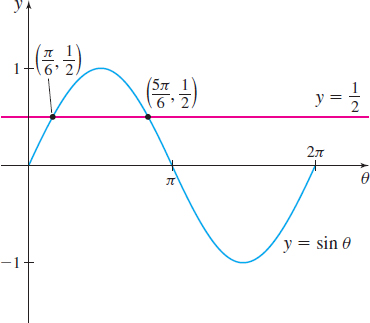
Solution (a) Use the inverse sine function \(y =\sin ^{-1} x\), \(-\dfrac{\pi}{2}\leq y\leq \dfrac{\pi}{2}\). \[ \begin{array}{l} \sin \theta = \frac{1}{2} \\ \,\,\,\theta = \sin ^{ - 1} \frac{1}{2}\, \quad {\color{#0066A7}{- \frac{\pi }{2} \le \theta \le \frac{\pi }{2}}} \\ \,\,\theta = \frac{\pi }{6} \\ \end{array} \]

Over the interval \([ 0,2\pi ] ,\) there are two angles \(\theta \) for which \(\sin \theta =\dfrac{1}{2}\). See Figure 89.

All the solutions of \(\sin \theta =\dfrac{1}{2}\) are given by the general formula \[ \theta =\dfrac{\pi }{6}+2k\pi\quad {\rm \ or \ }\quad \theta =\dfrac{5\pi }{6}+2k\pi,\qquad {\rm where }\ k \ \hbox{is any integer} \]

The solutions in the interval \([-2\pi, 2\pi] \) are \[ \left\{ -\dfrac{11\pi }{6},-\dfrac{7\pi }{6},\dfrac{\pi }{6 },\dfrac{5\pi }{6}\right\} \]

(b) A calculator must be used to solve \(\cos \theta =0.4\). Then \[ \theta =\cos ^{-1}\left( 0.4\right) \approx 1.159279 \quad 0\leq \theta \leq \pi \]

Rounded to three decimal places, \(\theta \,{=}\,\cos ^{-1}0.4=1.159\) radians. But there is another angle \(\theta \) in the interval \([ 0,2\pi ] \) for which \(\cos \theta =0.4\), namely, \(\theta \approx 2\pi -1.159\) \(\approx 5.124\) radians.

Because the cosine function has period \(2\pi \), all the solutions of \(\cos \theta =0.4\) are given by the general formulas \[ \theta \approx 1.159+2k\pi \quad \hbox{or} \quad \theta \approx 5.124+2k\pi,\qquad \hbox{ where } k \hbox{ is any integer} \]

The solutions in the interval \([ -2\pi ,2\pi ] \) are \(\left\{ -5.124,-1.159, 1.159, 5.124\right\}\).