Find the average rate of change of $f( x) =3x^{2}$:

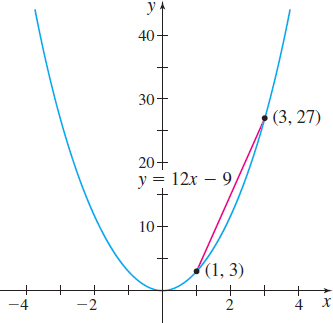
Figure 17 $f(x)=3x^2$

Solution (a) The average rate of change of $f( x) =3x^{2}$ from $1$ to $3$ is $$\dfrac{\Delta y}{\Delta x}=\dfrac{f( 3) -f( 1) }{3-1}= \dfrac{27-3}{3-1}=\dfrac{24}{2}=12$$

See Figure 17.

Notice that the average rate of change of $f( x) =3x^{2}$ from $1$ to $3$ is the slope of the line containing the points $( 1,3)$ and $( 3,27) .$

(b) The average rate of change of $f( x) =3x^{2}$ from $1$ to $x$ is $$\begin{eqnarray*} \dfrac{\Delta y}{\Delta x}&=&\dfrac{f( x) -f( 1) }{x-1}= \dfrac{3x^{2}-3}{x-1}=\dfrac{3( x^{2}-1) }{x-1}\nonumber \\ &=&\dfrac{3(x-1) ( x+1) }{x-1}=3( x+1) =3x+3 \end{eqnarray*}$$

provided $x\neq 1$.