Constructing a Model from a Verbal Description

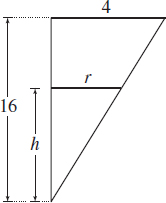
A liquid is poured into a container in the shape of a right circular cone with radius 4 meters and height 16 meters, as shown in Figure 33. Express the volume \(V\) of the liquid as a function of the height \(h\) of the liquid.

Solution The formula for the volume of a right circular cone of radius \(r\) and height \(h\) is \[ V=\dfrac{1}{3}\pi r^{2}h \]

The volume depends on two variables, \(r\) and \(h\). To express \(V\) as a function of \(h\) only, we use the fact that a cross section of the cone and the liquid form two similar triangles. See Figure 34.

Corresponding sides of similar triangles are in proportion. Since the cone's radius is 4 meters and its height is 16 meters, we have \begin{eqnarray*} \dfrac{r}{h} &=&\dfrac{4}{16}=\dfrac{1}{4} \\[4pt] r &=&\dfrac{1}{4}h \end{eqnarray*}

21

Then \[ \begin{array}{l} V = \frac{1}{3}\pi r^2 h\mathop = \limits_ {\color{#0066A7}{\uparrow}} \frac{1}{3}\pi \left( {\frac{1}{4}h} \right)^2 h = \frac{1}{{48}}\pi h^3 \\ \quad \quad \quad \;\;\;{\color{#0066A7}{r = \;\frac{1}{4}h}} \\ \end{array} \]

So \(V=V( h) =\dfrac{1}{48}\pi h^{3}\) expresses the volume \(V\) as a function of the height of the liquid. Since \(h\) is measured in meters, \(V\) will be expressed in cubic meters.