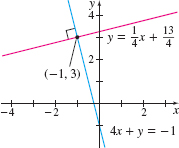
Finding an Equation of a Line that Is Perpendicular to a Given Line

Find an equation of the line that contains the point \(( -1,3)\) and is perpendicular to the line \(4x+y=-1.\)

Solution  We begin by writing the equation of the given line in slope-intercept form to find its slope. \begin{eqnarray*} 4x+y &=&-1 \\[0pt] y &=&-4x-1 \end{eqnarray*}

This line has a slope of \(-4.\) Any line perpendicular to this line will have slope \(\dfrac{1}{4}.\) Because the point \( ( -1,3)\) is on this line, we use the point-slope form of a line. \begin{eqnarray*} y-y_{1} &=&m( x-x_{1}) \\[3pt] y-3 &=&\dfrac{1}{4} [ x- ( -1) ] \\[4pt] y-3 &=&\dfrac{1}{4}x+\dfrac{1}{4} \\[4pt] y &=&\dfrac{1}{4}x+\dfrac{13}{4} \end{eqnarray*}

Figure 32 shows the graphs of \(4x+y=-1\) and \(y=\dfrac{1}{4}x+\dfrac{13}{4}\).