Solve the triangle with side $a=6,$ side $b=8$, and angle $A=35^\circ$, which is opposite side $a.$

Solution Because two sides and an opposite angle are known (SSA), we use the Law of Sines to find angle $B$. $$\dfrac{\sin A}{a}=\dfrac{\sin B}{b}$$

Since $a=6,$ $b=8,$ and $A=35 {{}^\circ},$ we have $$\begin{eqnarray*} \dfrac{\sin 35 {{}^\circ}}{6} &=&\dfrac{\sin B}{8} \end{eqnarray*}$$

A-37

$$\begin{eqnarray*} \sin B &=&\dfrac{8\sin 35 {{}^\circ}}{6}\approx 0.765 \end{eqnarray*}$$ $$B_{1}\approx 49.9 {{}^\circ}\quad\hbox{or}\quad B_{2}\approx 180 {{}^\circ}-49.9 {{}^\circ}=130.1 {{}^\circ}$$

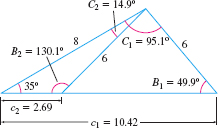
For both choices of $B$, we have $A+B < 180 {{}^\circ}$. So, there are two triangles, one containing the angle $B_{1}\approx 49.9 {{}^\circ}$ and the other containing the angle $B_{2}\approx 130.1 {{}^\circ}$. The third angle $C$ is either $$\begin{eqnarray*} C_{1}&=&180^\circ-A-B_{1} \underset{\underset{\underset{\color{#0066A7}{\hbox{\({B_{1}=49.9{{}^\circ}}\)}}}{\color{#0066A7}{\hbox{\({A=35{{}^\circ}}\)}}}}{\color{#0066A7}{\displaystyle\uparrow }}}{\approx} 95.1^\circ \qquad \hbox{or}\qquad C_{2}=180^\circ-A-B \underset{\underset{\underset{\color{#0066A7}{\hbox{\({B_{2}=130.1{{}^\circ}}\)}}}{\color{#0066A7}{\hbox{\({A=35{{}^\circ}}\)}}}}{\color{#0066A7}{\displaystyle\uparrow }}} {\approx} 14.9^\circ \end{eqnarray*}$$

Figure 63

The third side $c$ satisfies the Law of Sines, so $$\begin{array}{rl@{\qquad}rl} \dfrac{\sin A}{a}&=\dfrac{\sin C_{1}}{c_{1}} & \dfrac{\sin A}{a}&=\dfrac{\sin C_{2}}{c_{2}} \\ \dfrac{\sin 35{{}^\circ}}{6}&=\dfrac{\sin 95.1{{}^\circ}}{c_{1}} & \dfrac{\sin 35{{}^\circ}}{6}&=\dfrac{\sin 14.9{{}^\circ}}{c_{2}} \\ c_{1}&=\dfrac{6\sin 95.1{{}^\circ}}{\sin 35{{}^\circ}}\approx 10.42 & c_{2}&=\dfrac{6\sin 14.9{{}^\circ}}{\sin 35{{}^\circ}}\approx 2.69 \end{array}$$ The two solved triangles are illustrated in Figure 63.