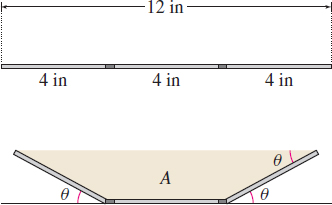
Constructing a Rain Gutter
A rain gutter is to be constructed using a piece of aluminum \(12\) in wide. After marking a length of \(4 \,{\rm in}\). from each edge, the piece of aluminum is bent up at an angle \(\theta\), as illustrated in Figure 19. The area \(A\) of a cross section of the opening, expressed as a function of \(\theta ,\) is \[ A(\theta) =16~\sin ~\theta ( \cos \theta +1)\qquad 0 \leq \theta \leq \dfrac{\pi }{2} \]
Find the angle \(\theta\) that maximizes the area \(A\). (This bend will allow the most water to flow through the gutter.)
Step 1 We locate all critical numbers in the open interval \(\left( 0,\dfrac{\pi }{2}\right)\). \[ \begin{eqnarray*} A^\prime (\theta ) &=& 16\sin \theta (-\!\sin \theta) +16\cos \theta ( \cos \theta +1) \hspace{12pc} \color{#0066A7}{{\hbox{Product Rule}}}\\[3pt] &=& 16[-\!\sin ^{2}\theta +\cos^{2}\theta +\cos \theta] \\[3pt] &=& 16[ ( \cos ^{2}\theta -1) +\cos ^{2}\theta +\cos \theta ] \hspace{15pc} \color{#0066A7}{{\hbox{$\kern-.5pt-\!\sin^2\theta=\cos^2\theta-1$}}}\\[3pt] &=& 16[2\cos^{2}\theta +\cos \theta -1] = 16(2\cos \theta -1) (\cos \theta +1) \end{eqnarray*} \]
The critical numbers satisfy the equation \(A^\prime (\theta ) =0, 0<\theta<\frac{\pi}{2}\). \[ \begin{eqnarray*} \begin{array}{rl@{ }l@{ }rl} 16(2\;\cos \theta -1) ( \cos \theta +1) &= 0 \\[3pt] 2\;\cos \theta -1 &= 0 & \quad\hbox{ or }\quad & \cos \theta +1 &=0 \\[3pt] \cos \theta &= \dfrac{1}{2} & \hbox{ }& \cos \theta &=-1\\[3pt] \theta &= \dfrac{\pi }{3} \;{\rm or}\; \dfrac{5\pi }{3} & \hbox{ }& \theta &= \pi \end{array} \end{eqnarray*} \]
Of these solutions, only \(\dfrac{\pi }{3}\) is in the interval \(\left( 0,\dfrac{\pi }{2}\right).\) So, \(\dfrac{\pi }{3}\) is the only critical number.
271
Step 2 We evaluate \(A\) at the critical number \(\dfrac{\pi }{3}\) and at the endpoints \(0\) and \(\dfrac{\pi }{2}\).
| \(\theta \) | \({16\sin \theta ( \cos \theta +1)} \) | \(A(\theta )\) | |
|---|---|---|---|
| \(0\) | \(16\sin 0\left( \cos 0+1\right) =0\) | \(0\) | |
| \(\dfrac{\pi }{3}\) | \[ \begin{eqnarray*} 16&\sin \dfrac{\pi }{3}\left( \cos \dfrac{\pi }{3} +1\right) \\ &= 16\left(\dfrac{\sqrt{3}}{2}\right) \left(\dfrac{1}{2}+1\right) \\ &= 16\left( \dfrac{3\sqrt{3}}{4}\right) =12\sqrt{3} \end{eqnarray*} \] | \(\approx\!20.8\) | \(\longleftarrow\) absolute maximum value |
| \(\dfrac{\pi }{2}\) | \[ \begin{eqnarray*} 16&\sin \dfrac{\pi }{2}\left(\cos \dfrac{\pi }{2}+1\right) \\ &=16( 1) (0+1) =16 \end{eqnarray*} \] | \(16\) |
Step 3 If the aluminum is bent at an angle of \(\dfrac{\pi }{3}\), the area of the opening is maximum. The maximum area is about \(20.8 \,{\rm in}^{2}\).