Find the arc length of $f(x) =x^{2/3}$ from $x=-1$ to $x=8.$

Solution Since the derivative $f'(x) =\dfrac{2}{3x^{1/3}}$ does not exist at $x=0$, we cannot use the arc length formula (1) to find the length of the graph from $x=-1$ to $x=8.$

442

From Figure 52, we observe that the length of the graph of $f$ from $x=-1$ to $x=8$ is the same as the length of the graph of $x=g_{1}( y) =-y^{3/2}$ from $y=0$ to $y=1$ plus the length of the graph of $x=g_{2}( y) =y^{3/2}$ from $y=0$ to $y=4.$

Figure 52 $f(x) =x^{2/3}, -1\leq x \leq 8.$

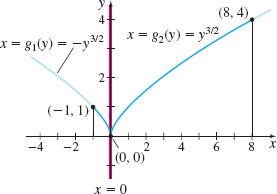
We investigate $x=g_{1}(y) =-y^{3/2}$ first. Since $g_{1}' (y) =-\dfrac{3}{2}y^{1/2}$ is continuous for all $y\geq 0.$ we can use arc length formula (2) to find the arc length $s_{1}$ of $g_{1}$ from $y=0$ to $y=1$. $$\begin{eqnarray*} s_{1}&=&\int_{0}^{1}\sqrt{1+[g_{1}'(y)]^{2}}{\it dy} \underset{\underset{\color{#0066A7}{\hbox{\(g_{1}'(y)\)} =-\frac{3}{2}y^{1/2}}}{\color{#0066A7}{\uparrow }}}{=}\int_{0}^{1} \sqrt{1+\left(\frac{3}{2}y^{1/2}\right) ^{2}}~{\it dy}\\[-11pt] &=&\int_{0}^{1}\sqrt{1+ \frac{9}{4}y}~{\it dy}=\frac{1}{2}\int_{0}^{1}\sqrt{4+9y}~{\it dy} \end{eqnarray*}$$

We use the substitution $u=4+9y$. Then ${\it du}=9~{\it dy}$ or, equivalently, ${\it dy}= \dfrac{{\it du}}{9}$. The limits of integration are $u=4$ when $y=0$, and $u=13$ when $y=1.$ $$\begin{equation*} s_{1}=\frac{1}{2}\displaystyle \int_{4}^{13}\sqrt{u}~\frac{{\it du}}{9}=\frac{1}{18}\left[ \frac{u^{3/2}}{\dfrac{3}{2}}\right] _{4}^{13}=\frac{1}{27}\big( 13\sqrt{13}-8\big) \end{equation*}$$

Now we investigate $x=g_{2}(y)$. Since $g_{2}' (y) =\dfrac{3}{2}y^{1/2}$ is continuous for all $y\geq 0,$ we can use arc length formula (2) to find the arc length $s_{2}$ of $g_{2}$ from $y=0$ to $y=4$. $$\begin{array}{rcl@{\qquad}l} s_{2} &=&\int_{0}^{4}\sqrt{1+[g_{2}'(y)] ^{2}}~{\it dy}=\int_{0}^{4}\sqrt{1+\left(\dfrac{3}{2}y^{1/2}\right)^{~2}} ~{\it dy}\\ &=&\int_{0}^{4}\sqrt{1+\dfrac{9}{4}y}~{\it dy} =\frac{1}{2}\int_{0}^{4}\sqrt{4+9y}~{\it dy} &\color{#0066A7}{\hbox{Let \(u=4+9y \); }}\\ &&&\color{#0066A7}{\hbox{\(du=9dy \).}}\\ &=&\frac{1}{2}\int_{4}^{40}\sqrt{u}~\frac{{\it du}}{9}=\frac{1}{18}\left[ \frac{u^{3/2}}{\frac{3}{2}}\right] _{4}^{40}=\frac{1}{27}\big( 80\sqrt{10}-8\big) \end{array}$$

The arc length $s$ of $y=f(x) =x^{2/3}$ from $x=-1$ to $x=8$ is the sum $$\begin{eqnarray*} s&=&s_{1}+s_{2}=\frac{1}{27}\big( 13\sqrt{13}-8\big) +\dfrac{1}{27}\big( 80\sqrt{10}-8\big)\\[4pt] &=&\frac{1}{27} \big( 80\sqrt{10}+13\sqrt{13}-16\big) \end{eqnarray*}$$