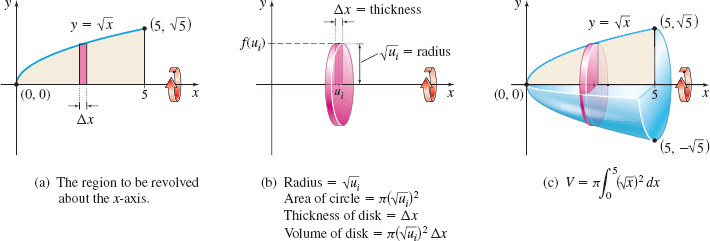
Using the Disk Method: Revolving About the \(x\)-Axis

Find the volume of the solid of revolution generated by revolving the region bounded by the graph of \(y=\sqrt{x}\), the \(x\)-axis, and the line \(x=5\) about the \(x\)-axis.

Solution We begin by graphing the region to be revolved. See Figure 16(a). Figure 16(b) shows a typical disk and Figure 16(c) shows the solid of revolution. Using the disk method, the volume \(V\) of the solid of revolution is \[ V=\pi \int_{0}^{5}( \sqrt{x}) ^{2}~{\it dx}=\pi \int_{0}^{5} x~{\it dx}= \pi \!\left[\dfrac{x^{2}}{2}\right] _{0}^{5}=\dfrac{25}{2} \pi \hbox{ cubic units} \]