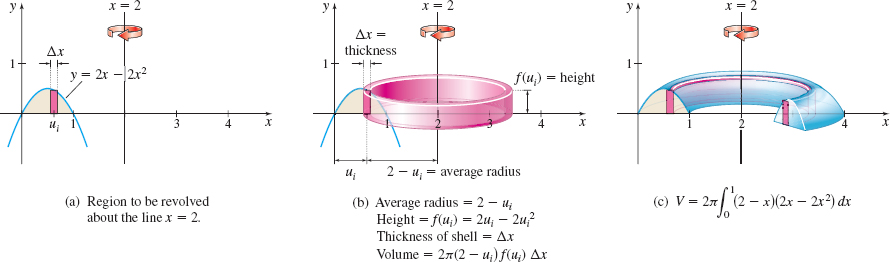
Using the Shell Method: Revolving About the Line \(x=2\)

Find the volume \(V\) of the solid of revolution generated by revolving the region bounded by the graph of \(y=2x-2x^{2}\) and the \(x\)-axis about the line \(x=2\).

Solution The region bounded by the graph of \(y=2x-2x^{2}\) and the \(x\)-axis is illustrated in Figure 37(a). A typical shell formed by revolving the region about the line \(x=2\), as shown in Figure 37(b), has an average radius of \(2-u_{i}\), height \(f(u_{i}) = 2u_{i}-2u_{i}^{2}\), and thickness \(\Delta x\). The solid of revolution is depicted in Figure 37(c).

431

The volume \(V\) of the solid is \begin{eqnarray*} V&=&2\pi \displaystyle \int_{0}^{1}(2-x)(2x-2x^{2})~{\it dx}=4\pi \displaystyle \int_{0}^{1}(x^{3}-3x^{2}+2x)~{\it dx}\\[4pt] &=&4\pi \left[ \dfrac{x^{4}}{4}-x^{3}+x^{2} \right] _{0}^{1}=\pi \hbox{ cubic units} \end{eqnarray*}