Using the Slicing Method to Find the Volume of a Solid

Find the volume \(V\) of the solid whose base is the region bounded by the graphs of \(y=\sqrt{x}\) and \(y=\dfrac{1}{8}x^{2}\), if slices perpendicular to the base along the \(x\)-axis have cross sections that are squares.

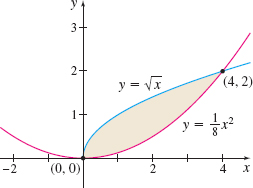
Solution We begin by graphing the region bounded by the graphs of \(y=\sqrt{x}\) and \(y=\dfrac{1}{8}x^{2}\), as shown in Figure 43. The points of intersection of the two graphs are found by solving the equation \begin{eqnarray*} \sqrt{x} &=&\dfrac{1}{8}x^{2} \\[2pt] x &=&\dfrac{1}{64}x^{4} \\[2pt] x^{4}-64x &=&0 \\[2pt] x( x^{3}-64) &=&0 \\[2pt] x &=&0 \quad\hbox{or}\quad x=4 \end{eqnarray*}

The two graphs intersect at the points \(\left( 0,0\right)\) and (4,2).

The solid with slices perpendicular to the base along the \(x\)-axis that are squares is shown in Figure 44(a). See Figure 44(b). A slice perpendicular to the \(x\)-axis at \(x_{i}\) is a square with side \( s_{i}=\displaystyle\sqrt{x_{i}}-\dfrac{1}{8}x_{i}^{2}.\) The area \(A_{i}\) of the cross section at \(x_{i}\) is \[ A_{i}=s_{i}^{2}=\left( \sqrt{x_{i}}-\dfrac{1}{8} x_{i}^{2}\right) ^{2} \]

437

See Figure 44(c). The volume \(V_{i}\) of a typical slice is \begin{eqnarray*} V_{i}&=& \hbox{(Area of the cross section)(Thickness of the slice)}\\[3pt] &=&A (x_{i}) \Delta x=\left( \sqrt{x_{i}}-\dfrac{1}{8}x_{i}^{2}\right) ^{2}\Delta x \end{eqnarray*}

The volume \(V\) of the solid is \begin{eqnarray*} V &=&\int_{0}^{4}A(x) {\it dx}=\int_{0}^{4}\left( \sqrt{x}-\dfrac{1}{8} x^{2}\right) ^{2}{\it dx}=\int_{0}^{4}\left( x-\dfrac{1}{4}x^{5/2}+\dfrac{1}{64} x^{4}\right) {\it dx} \notag \\[5pt] &=&\left[ \dfrac{x^{2}}{2}-\dfrac{1}{14}x^{7/2}+\dfrac{1}{320}x^{5}\right] _{0}^{4}=8-\dfrac{128}{14}+\dfrac{1024}{320}=\dfrac{72}{35}\hbox{ cubic units } \end{eqnarray*}