Use Trigonometric Substitution to Find a Definite Integral

Find the area under the graph of \(y=\sqrt{x^{2}-1}\) (the upper half of the right branch of the hyperbola \(y^{2}=x^{2}-1\)) from 1 to 3. See Figure 9.

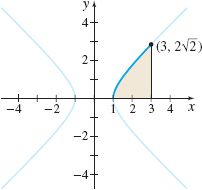
Solution The area \(A\) we seek is \(A= \int_{1}^{3}\sqrt{x^{2}-1 }\,dx\). The integral contains a square root of the form \(\sqrt{x^{2}-a^{2}}\), where \(a=1\), so we use the trigonometric substitution \(x=\sec \theta \), \( 0 \le \theta \lt\dfrac{\pi }{2},\) \(\pi \le \theta \lt\dfrac{3\pi }{2}.\) Then \(dx=\sec \theta \tan \theta \,d\theta \). Since the upper limit \(x=3\) does not result in a nice angle \((\theta =\sec ^{-1}\,3)\), we find the indefinite integral first and then use the Fundamental Theorem of Calculus.

With \(x=\sec \theta \hbox{ and } dx=\sec \theta \tan \theta d\theta ,\) we have \[ \begin{eqnarray*} A&=&\int \sqrt{x^{2}-1}\,dx=\int \sqrt{\sec ^{2}\theta -1}\sec \theta \tan \theta \,d\theta =\int \tan \theta \cdot \sec \theta \tan \theta \,d\theta\\[5pt] &=&\int \tan ^{2}\theta \sec \theta d\theta \end{eqnarray*} \]

Since \(\tan \theta \) is raised to an even power and \(\sec \theta \) to an odd power, we use the identity \(\tan ^{2}\theta =\sec ^{2}\theta -1.\)Then \[ \begin{eqnarray*} A &=&\int \sqrt{x^{2}-1}\,dx=\int \tan ^{2}\theta \sec \theta d\theta =\int \left( \sec ^{2}\theta -1\right) \sec \theta d\theta\\[4pt] &=&\int \sec ^{3}\theta d\theta -\int \sec \theta d\theta \\[4pt] &=&\dfrac{1}{2}\left[ \sec \theta \tan \theta +\ln \left\vert \sec \theta +\tan \theta \right\vert \right] -\ln \left\vert \sec \theta +\tan \theta \right\vert +C\\[4pt] &=&\dfrac{1}{2}\sec \theta \tan \theta -\dfrac{1}{2}\ln \left\vert \sec \theta +\tan \theta \right\vert +C \end{eqnarray*} \]

Now we express \(\tan \theta \) in terms of \(x=\sec \theta ,\) and apply the Second Fundamental Theorem of Calculus. \[ \begin{eqnarray*} A&=&\int_{1}^{3}\sqrt{x^{2}-1}\, dx\underset{\underset{\underset{\color{#0066A7}{\hbox{\(\tan \theta =\sqrt{x^{2} -1}\)}}}{\color{#0066A7}{\hbox{\(\sec \theta =x\)}}}}{\color{#0066A7}{\uparrow }}} {=}\left[ \frac{1}{2}x\sqrt{x^{2}-1}-\frac{1}{2}\ln \left\vert x+ \sqrt{x^{2}-1}\right\vert \right] _{1}^{3}\\ &=&\dfrac{3}{2}\sqrt{8}-\dfrac{1}{2}\ln ( 3+\sqrt{8}) =3\sqrt{2} -\dfrac{1}{2}\ln (3+2\sqrt{2}) \end{eqnarray*} \]