Finding the Area Enclosed by a Rose

Find the area enclosed by the graph of \(r=2\cos ( 3\theta ) \), a rose with three petals.

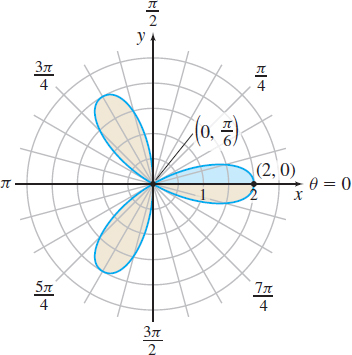
Solution Figure 52 shows the rose. The area of the blue shaded region in quadrant I equals one-sixth of the area \(A\) enclosed by the graph.* The shaded region in quadrant I is swept out beginning with the ray \(\theta =0.\) It ends at the point \(( 0,\theta ) ,\) \(0\lt\theta \lt\dfrac{\pi }{2},\) where \(\theta \) is the solution of the equation. \[ \begin{eqnarray*} 2\cos ( 3\theta ) &=&0\qquad 0\lt\theta \lt\dfrac{\pi }{2} \\[4pt] \cos ( 3\theta ) &=&0 \\[4pt] 3\theta &=&\dfrac{\pi }{2}+2k\pi \\[4pt] \theta &=&\dfrac{\pi }{6}+\dfrac{2k\pi }{3} \end{eqnarray*} \]

* We need to exploit symmetry here since there are intervals on which \(r\lt0,\) and the area formula requires that \(r>0.\)

Since \(0\lt\theta \lt\dfrac{\pi }{2},\) we have \(\theta =\dfrac{\pi }{6}.\) The area of the shaded region in quadrant I swept out by the rays \(\theta =0\) and \(\theta =\dfrac{\pi }{6}\) is given by \(\int_{0}^{\pi /6}\dfrac{1}{2} r^{2}d\theta ,\) and the area \(A\) of the region we seek is \(6\) times this area. \[ \begin{eqnarray*} A &=& 6\int_{0}^{\pi /6}\dfrac{1}{2}r^{2}\,d\theta =3\int_{0}^{\pi /6} 4\cos ^{2}( 3\theta ) ~d\theta =12\int_{0}^{\pi /6}\cos ^{2}( 3\,\theta )~d\theta\\[4pt] &=& 12\int_{0}^{\pi /6}\frac{1+\cos ( 6\theta ) }{2}~d\theta\qquad {\color{#0066A7}{\hbox{\(\cos ^{2}( 3\theta ) =\dfrac{1+\cos ( 6\theta ) }{2}\)}}}\\\\[4pt] &=&6 \left[ \theta +\dfrac{1}{6}\sin ( 6\theta ) \right] _{0}^{\pi /6}=6\left( \frac{\pi }{6}\right) =\pi \end{eqnarray*} \]