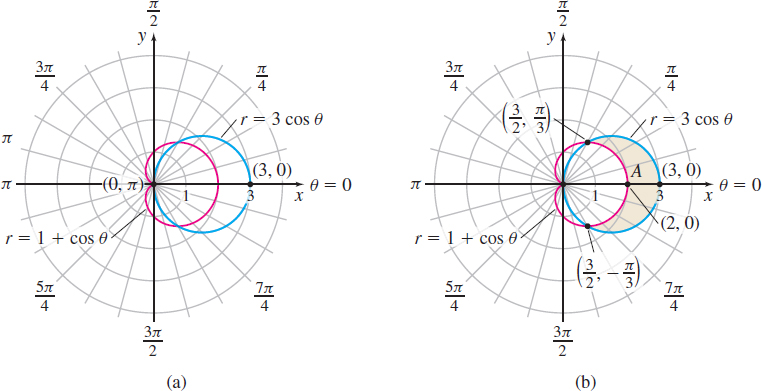
Finding the Area of the Region Enclosed by the Graphs of Two Polar Equations

Find the area of the region that lies outside the cardioid \(r=1+\cos \theta \) and inside the circle \(r=3\cos \theta \).

Solution We begin by graphing each equation. See Figure 54(a). Then we find the points of intersection of the two graphs by solving the equation, \[ \begin{eqnarray*} 3\cos \theta & =&1+\cos \theta \\[3pt] 2\cos \theta & =&1 \\[3pt] \cos \theta & =&\frac{1}{2} \end{eqnarray*} \] \[ \begin{equation*} \theta =-\frac{\pi }{3}\;\hbox{or}\;\theta =\frac{\pi }{3} \end{equation*} \]

The graphs intersect at the points \(\left( \dfrac{3}{2},-\dfrac{\pi }{3} \right) \) and \(\left( \dfrac{3}{2},\dfrac{\pi }{3}\right) \).

The area \(A\) of the region that lies outside the cardioid and inside the circle is shown as the shaded portion in Figure 54(b). Notice that the area \( A\) is the difference between the area of the region enclosed by the circle \( r=3\cos \theta \) swept out by the rays \(\theta =-\dfrac{\pi }{3}\) and \( \theta =\dfrac{\pi }{3}\), and the area of the region enclosed by the cardioid \(r=1+\cos \theta \) swept out by the same rays. So, \[ \begin{array}{@rcl} A& =&\int_{-\pi /3}^{\pi /3}\frac{1}{2}(3\cos \theta )^{2}d\theta -\int_{-\pi /3}^{\pi /3}\frac{1}{2}(1+\cos \theta )^{2}d\theta =\frac{1}{2} \int_{-\pi /3}^{\pi /3}[ 9\cos ^{2}\theta -( 1+2\cos \theta +\cos^{2}\theta ) ] \,d\theta \notag \\[12pt] & =&\frac{1}{2}\int_{-\pi /3}^{\pi /3}(8\cos ^{2}\theta -1-2\cos \theta )~d\theta = \frac{1}{2}\int_{-\pi /3}^{\pi /3} \left[ 8\left( \frac{1+\cos ( 2\theta ) }{2}\right) -1-2\cos \theta \right] d\theta \notag \\[12pt] & =&\frac{1}{2}\int_{-\pi /3}^{\pi /3}[ 3+4\cos ( 2\theta ) -2\cos \theta ] ~d\theta =\frac{1}{2}\Big[ 3\theta +2\sin ( 2\theta ) -2\sin \theta \Big] _{-\pi /3}^{\pi /3}=\pi \end{array} \]