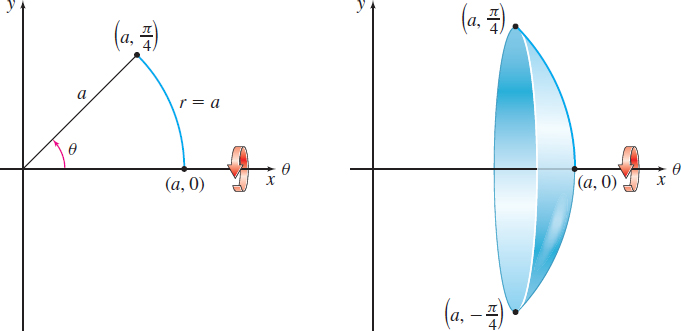
EXAMPLE 6Finding the Surface Area of a Solid of Revolution
Find the surface area of the solid of revolution generated by revolving the arc of the circle r=a, a>0, 0≤θ≤π4, about the polar axis.
Solution See Figure 55. We find the surface area S using formula (1). Since r=f(θ)=a,f′(θ)=0. Then S=2π∫βαf(θ)sinθ√[f(θ)]2+[f′(θ)]2 dθ=2π∫π/40asinθ√a2dθ=2πa2∫π/40sinθdθ=2πa2[−cosθ]π/40=2πa2(−√22+1)=πa2(2−√2)