Find a rectangular equation of the curve whose parametric equations are $$\begin{equation*} x( t) =R\, \cos t \qquad y( t) =R\, \sin t \end{equation*}$$

where $R>0$ is a constant. Graph the plane curve and indicate its orientation.

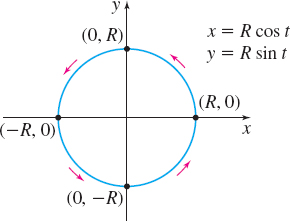
Figure 3 The orientation is counterclockwise.

Solution The presence of the sine and cosine functions in the parametric equations suggests using the Pythagorean Identity $\cos ^{2}t+\sin ^{2}t=1$. Then $$\begin{array}{rcl@{\quad}crcl} \left( \dfrac{x}{R}\right) ^{2}+\left( \dfrac{y}{R}\right) ^{2}& =&1 &\qquad\color{#0066A7}{\cos \;t = \tfrac{x}{R}\quad \sin t=\dfrac{y}{R}}\\ x^{2}+y^{2}& =& R^{2} \end{array}$$

The graph of the rectangular equation is a circle with center at the origin and radius $R$. In the parametric equations, as the parameter $t$ increases, the points $(x,y)$ on the circle are traced out in the counterclockwise direction, as shown in Figure 3.