Find the length $s$ of one arch of the cycloid: $$\begin{equation*} x(t) =a(t-\sin t)\qquad y(t) =a(1-\cos t)\quad a>0 \end{equation*}$$

Figure 17 shows the graph of the cycloid.

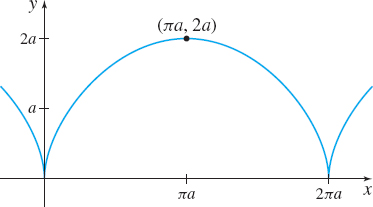
Figure 17 $x(t) =a(t-\sin t)$, $y(t) =a(1-\cos t),\quad a>0$.

Solution One arch of the cycloid is obtained when $t$ varies from $0$ to $2\pi .$ Since $$\begin{equation*} \dfrac{dx}{dt}=a-a\cos t=a(1-\cos t)\qquad\hbox{and}\qquad \dfrac{dy}{dt}=a\sin t \end{equation*}$$

are both continuous and are never simultaneously $0$ on $( 0,2\pi)$, the cycloid is smooth on $[ 0,2\pi ]$. The arc length $s$ is $$\begin{eqnarray*} s&=&\int_{a}^{b}\sqrt{\left( \dfrac{dx}{dt}\right) ^{2}+\left( \dfrac{dy}{dt} \right) ^{2}}~dt=\int_{0}^{2\pi } \sqrt{a^{2}(1-\cos t)^{2}+a^{2}\sin ^{2}t} \\ &=&a \int^{2\pi}_0 \sqrt{( 1-2\cos t+\cos ^{2}t) +\sin ^{2}t} ~dt = a \int^{2\pi}_0 \sqrt{1-2\cos t+1}~dt\\ &=& \sqrt{2} a \int^{2\pi}_0 \sqrt{1-\cos t}~dt \end{eqnarray*}$$

To integrate $\sqrt{1-\cos t}$ we use a half-angle identity. Since $\sin \dfrac{t}{2}\geq 0$ if $0\leq t \leq 2\pi ,$ we have $\sin \dfrac{t}{2}=\sqrt{\dfrac{1-\cos t}{2}}.$ Then $$\begin{equation*} \sqrt{1-\cos t}=\sqrt{2}\sin \frac{t}{2} \end{equation*}$$

Now the arc length $s$ from $t=0$ to $t=2\pi$ is $$s= \sqrt{2}a \int_{0}^{2\pi } \sqrt{1 - \cos t}\, dt=\sqrt{2} a \int_{0}^{2\pi }\sqrt{2} \sin \frac{t}{2}~dt=2a\left[ -2\cos \frac{t}{2}\right] _{0}^{2\pi }=8a$$