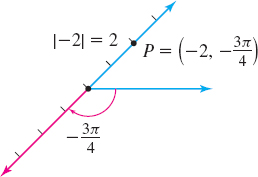
Plotting a Point Using Polar Coordinates

Plot the point \(P\) whose polar coordinates are \(\left( -2,-\dfrac{3\pi }{4}\right)\). Then find three other polar coordinates of the same point with the properties:

(a) \(r>0\) and \(0<\theta <2\pi\)

(b) \(r>0\) and \(-2\pi <\theta <0\)

(c) \(r<0\) and \(0<\theta <2\pi\)

Solution  The point \(\left( -2,-\dfrac{3\pi }{4}\right)\) is located by first drawing the angle \(-\dfrac{3\pi }{4}\). Then \(P\) is on the extension of the terminal side of \(\theta \) through the pole at a distance \(2\) units from the pole, as shown in Figure 31.

(a) The point \(P=( r,\theta ) ,\) \(r>0,\) \(0<\theta<2\pi\) is \(\left( 2,\dfrac{\pi }{4}\right)\), as shown in Figure 32(a).

(b) The point \(P=( r,\theta ) ,\) \(r>0,\) \(-2\pi <\theta<0\) is \(\left( 2,-\dfrac{7\pi }{4}\right)\), as shown in Figure 32(b).

(c) The point \(P=( r,\theta ) ,\) \(r<0,\) \(0<\theta<2\pi\) is \(\left( -2,\dfrac{5\pi }{4}\right)\), as shown in Figure 32(c).