Finding the Area Between the Graphs of Two Functions

Find the area of the region enclosed by the graphs of \(f(x)=10x-x^{2}\) and \(g(x)=3x-8\).

Solution First we graph the two functions. See Figure 4.

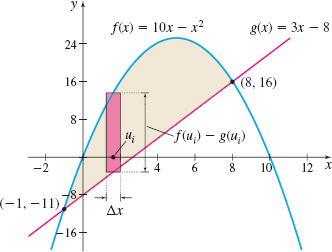
The area we seek lies between the points of intersection of the graphs. Finding the \(x\)-values of the points of intersection will identify the limits of integration. We solve the equation \(f(x) =g(x)\) to find these values. \begin{eqnarray*} 10x-x^{2} &=&3x-8 \qquad \qquad {\color{#0066A7}{f( x ) =g (x)}} \\[1pt] x^{2}-7x-8 &=&0 \\[1pt] (x+1)(x-8)&=&0 \\[1pt] x &=&-1 \hbox{ or } x=8 \end{eqnarray*}

407

The limits of integration are \(a=-1\) and \(b=8.\) Since \(f(x) \geq g(x)\) on \(\left[ -1,8\right],\) the area \(A\) is given by \begin{eqnarray*} A &=&\int_{a}^{b}[f(x)-g(x)]~{\it dx}=\int_{-1}^{8}[(10x-x^{2})-(3x-8)]~{\it dx}\\[5pt] &=& \int_{-1}^{8}(-x^{2}+7x+8)~{\it dx}=\left[ \dfrac{-x^{3}}{3}+\dfrac{7x^{2}}{2}+8x \right] _{-1}^{8} \\[5pt] &=&\left( -\dfrac{512}{3}+224+64\right) -\left( \dfrac{1}{3}+\dfrac{7}{2}-8\right) =\dfrac{243}{2}=121.5\hbox{ square units} \end{eqnarray*}