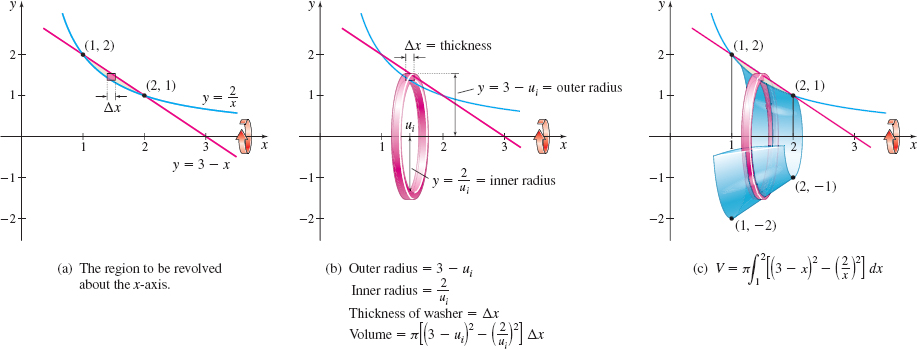
Using the Washer Method: Revolving About the \(x\)-Axis

Find the volume \(V\) of the solid of revolution generated by revolving the region bounded by the graphs of \(y=\dfrac{2}{x}\) and \(y=3-x\) about the \(x\)-axis.

Solution We begin by graphing the two functions. See Figure 24(a). The \(x\)-coordinates of the points of intersection of the graphs satisfy the equation \begin{eqnarray*} \dfrac{2}{x} &=&3-x \\[3pt] x^{2}-3x+2 &=&0 \\[3pt] ( x-1) ( x-2) &=&0 \end{eqnarray*}

So, the region to be revolved lies between \(x=1\) and \(x=2\). Notice that the graph of \(y=3-x\) lies above the graph of \(y=\dfrac{2}{x}\) on the interval \(\left[ 1,\,2\right]\).

As illustrated in Figure 24(b), if we partition the \(x\)-axis, the volume \(V_{i}\) of a typical washer is \begin{equation*} V_{i}=\pi \left[ \left( \hbox{Outer radius}\right) ^{2}-\left( \hbox{Inner radius}\right) ^{2}\right] \Delta x=\pi \left[ (3-u_{i})^{2}-\left( \frac{2}{u_{i}}\right) ^{2}\right] \Delta x \end{equation*}

The volume \(V\) of the solid of revolution is \begin{eqnarray*} V&=&\pi \displaystyle \int_{1}^{2}\left[ (3-x)^{2}-\left( \dfrac{2}{x}\right) ^{2}\right]~{\it dx}=\pi \displaystyle \int_{1}^{2}\left( 9-6x+x^{2}-\dfrac{4}{x^{2}}\right) ~{\it dx}\\[4pt] &=&\pi \left[9x-3x^{2}+\dfrac{x^{3}}{3}+\dfrac{4}{x}\right] _{1}^{2}=\dfrac{\pi}{3}\hbox{ cubic units} \end{eqnarray*}