8.2Gibbs Free Energy Is a Useful Thermodynamic Function for Understanding Enzymes
Gibbs Free Energy Is a Useful Thermodynamic Function for Understanding Enzymes
Enzymes speed up the rate of chemical reactions, but the properties of the reaction—
The free-energy change provides information about the spontaneity but not the rate of a reaction
As discussed in Chapter 1, the free-
A reaction can take place spontaneously only if ΔG is negative. Such reactions are said to be exergonic.
A system is at equilibrium and no net change can take place if ΔG is zero.
A reaction cannot take place spontaneously if ΔG is positive. An input of free energy is required to drive such a reaction. These reactions are termed endergonic.
The ΔG of a reaction depends only on the free energy of the products (the final state) minus the free energy of the reactants (the initial state). The ΔG of a reaction is independent of the molecular mechanism of the transformation. For example, the ΔG for the oxidation of glucose to CO2 and H2O is the same whether it takes place by combustion or by a series of enzyme-
catalyzed steps in a cell. The ΔG provides no information about the rate of a reaction. A negative ΔG indicates that a reaction can take place spontaneously, but it does not signify whether it will proceed at a perceptible rate. As will be discussed shortly (Section 8.3), the rate of a reaction depends on the free energy of activation (ΔG‡), which is largely unrelated to the ΔG of the reaction.
219
The standard free-energy change of a reaction is related to the equilibrium constant
As for any reaction, we need to be able to determine ΔG for an enzyme-
Consider the reaction

The ΔG of this reaction is given by
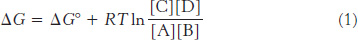
in which ΔG° is the standard free-
Units of energy
A kilojoule (kJ) is equal to 1000 J.
A joule (J) is the amount of energy needed to apply a 1-
A kilocalorie (kcal) is equal to 1000 cal.
A calorie (cal) is equivalent to the amount of heat required to raise the temperature of 1 gram of water from 14.5°C to 15.5°C. 1 kJ = 0.239 kcal.
A convention has been adopted to simplify free-
A simple way to determine ΔG°′ is to measure the concentrations of reactants and products when the reaction has reached equilibrium. At equilibrium, there is no net change in reactants and products; in essence, the reaction has stopped and ΔG = 0. At equilibrium, equation 1 then becomes
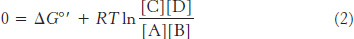
and so
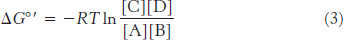
The equilibrium constant under standard conditions, 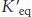 is defined as
is defined as

Substituting equation 4 into equation 3 gives

which can be rearranged to give

220
Substituting R = 8.315 × 10−3 kJ mol−1 deg−1 and T = 298 K (corresponding to 25° C) gives

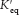 (at 25°C)
(at 25°C)
|
|
ΔG°′ |
|
|---|---|---|
|
|
kJ mol−1 |
kcal mol−1 |
|
10−5 |
28.53 |
6.82 |
|
10−4 |
22.84 |
5.46 |
|
10−3 |
17.11 |
4.09 |
|
10−2 |
11.42 |
2.73 |
|
10−1 |
5.69 |
1.36 |
|
1 |
0.00 |
0.00 |
|
10 |
−5.69 |
−1.36 |
|
102 |
−11.42 |
−2.73 |
|
103 |
−17.11 |
−4.09 |
|
104 |
−22.84 |
−5.46 |
|
105 |
−28.53 |
−6.82 |
where ΔG°′ is here expressed in kilojoules per mole because of the choice of the units for R in equation 7. Thus, the standard free energy and the equilibrium constant of a reaction are related by a simple expression. For example, an equilibrium constant of 10 gives a standard free-
As an example, let us calculate ΔG°′ and ΔG for the isomerization of dihydroxyacetone phosphate (DHAP) to glyceraldehyde 3- . The standard free-
. The standard free-
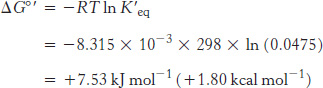
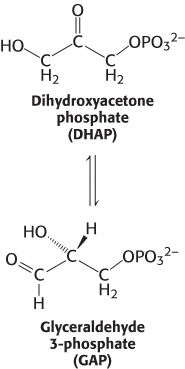
Under these conditions, the reaction is endergonic. DHAP will not spontaneously convert into GAP.
Now let us calculate ΔG for this reaction when the initial concentration of DHAP is 2 × 10−4 M and the initial concentration of GAP is 3 × 10−6 M. Substituting these values into equation 1 gives
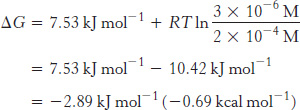
This negative value for the ΔG indicates that the isomerization of DHAP to GAP is exergonic and can take place spontaneously when these species are present at the preceding concentrations. Note that ΔG for this reaction is negative, although ΔG°′ is positive. It is important to stress that whether the ΔG for a reaction is larger, smaller, or the same as ΔG°′ depends on the concentrations of the reactants and products. The criterion of spontaneity for a reaction is ΔG, not ΔG°′. This point is important because reactions that are not spontaneous based on ΔG°′ can be made spontaneous by adjusting the concentrations of reactants and products. This principle is the basis of the coupling of reactions to form metabolic pathways (Chapter 15).
Enzymes alter only the reaction rate and not the reaction equilibrium
Because enzymes are such superb catalysts, it is tempting to ascribe to them powers that they do not have. An enzyme cannot alter the laws of thermodynamics and consequently cannot alter the equilibrium of a chemical reaction. Consider an enzyme-
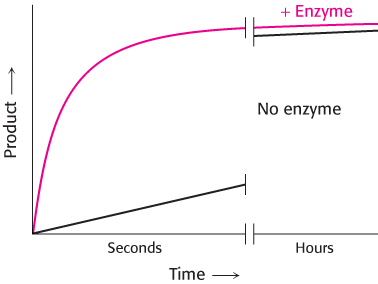
221
Why does the rate of product formation level off with time? The reaction has reached equilibrium. Substrate S is still being converted into product P, but P is being converted into S at a rate such that the amount of P present stays the same.
Let us examine the equilibrium in a more quantitative way. Suppose that, in the absence of enzyme, the forward rate constant (kF) for the conversion of S into P is 10−4 s−1 and the reverse rate constant (kR) for the conversion of P into S is 10−6 s−1. The equilibrium constant K is given by the ratio of these rate constants:
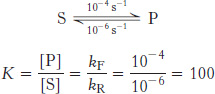
The equilibrium concentration of P is 100 times that of S, whether or not enzyme is present. However, it might take a very long time to approach this equilibrium without enzyme, whereas equilibrium would be attained rapidly in the presence of a suitable enzyme (Table 8.1). Enzymes accelerate the attainment of equilibria but do not shift their positions. The equilibrium position is a function only of the free-