Every model of imperfect competition that we’ve looked at so far—collusion, Bertrand, Cournot, and Stackelberg—has assumed that the industry’s producers all sell the same product. Often, however, a more realistic description of an industry is a set of firms that make similar but not identical products. When consumers buy cars, breakfast cereals, pest-control services, or many other products, they must choose between competing versions and brands, each with its own unique features, produced by a small number of companies. A market in which multiple varieties of a common product type are available is called a differentiated product market.

How can we analyze a “market” when the products aren’t the same? Shouldn’t each product be considered to exist in its own separate market? Not always—it is often possible to treat the products as interacting in a single market. The key is to explicitly account for the way consumers are willing to substitute among the products.

To see how a Bertrand oligopoly works with differentiated products, think back to the Bertrand model we studied in Section 11.3. There, two companies (Walmart and Target in our example) competed by setting prices for an identical product (the Sony PS4). Now, however, instead of thinking of the firms’ products as identical as we did in Section 11.3, we assume that consumers view the products as being somewhat distinct. Maybe this is because, even though a PS4 console is the same regardless of where customers buy it, the stores have different locations and customers care about travel costs. Or, perhaps the stores have different atmospheres or return policies or credit card programs that matter to certain customers. The specific source of the product distinction isn’t important. Regardless of its source, this differentiation helps the stores exert more market power and earn more profit. When products were identical, the incentive to undercut price was so intense that firms competed the market price right down to marginal cost and earned zero economic profit as a result. That is not the outcome in the differentiated-product Bertrand model, as we see in the following example.

448

Suppose there are two main manufacturers of snowboards, Burton and K2. Because many snowboarders view the two companies’ products as similar but not identical, if either firm cuts its prices, it will gain market share from the other. But because the firms’ products aren’t perfect substitutes, the price-cutting company won’t take all of the business away from the other company just because it sets its price a bit lower. Some people are still going to prefer the competitor’s product, even at a higher price.

This product differentiation means that each firm faces its own demand curve, and each product’s price has a different effect on the firm’s demand curve. So, Burton’s demand curve might be

q_B = 900 – 2p_B + p_K

As you can see, the quantity of boards Burton sells goes down when it raises the price it charges for its own boards, p_B. On the other hand, Burton’s quantity demanded goes up when K2 raises its price, p_K. In this example, we’ve assumed that Burton’s demand is more sensitive to changes in its own price than to changes in K2’s price. (For every $1 change in p_B , there is a 2-unit decrease in quantity demanded; this ratio is 1 to 1—and positive—for changes in p_K.) This is a realistic assumption in many markets.

K2 has a demand curve that looks similar, but with the roles of the two firms’ prices reversed:

q_K = 900 – 2p_K + p_B

The responses of each company’s quantity demanded to price changes reflect consumers’ willingness to substitute across varieties of the industry’s product. But this substitution is limited; a firm can’t take over the entire market with a 1 cent price cut, as it can in the identical-products Bertrand model.

To determine the equilibrium in a Bertrand oligopoly model with differentiated products, we follow the same steps we used for all the other models: Assume each company sets its price to maximize its profit, taking the prices of its competitors as given. That is, we look for a Nash equilibrium. To make things simple, we assume that both firms have a marginal cost of zero.10

449

Burton’s total revenue is

TR_B = p_B × q_B = p_B × (900 – 2p_B + p_K )

Notice that we’ve written total revenue in terms of Burton’s price, rather than its quantity. This is because in a Bertrand oligopoly, Burton chooses the price it will charge rather than how much it will produce. Writing total revenue in price terms lets us derive the marginal revenue curve in price terms as well. Namely, marginal revenue is

MR_B = 900 – 4p_B + p_K

(Recall that the marginal revenue curve of a linear demand curve is just the demand curve with the price coefficient doubled.) We can solve for Burton’s profit-maximizing price through the usual step of setting this marginal revenue equal to the marginal cost (zero in this case). Doing so and rearranging give

MR_B = 900 – 4p_B + p_K = 0

4 p_B = 900 + p_K

p_B = 225 + 0.25p_K

Notice how this again gives a firm’s (Burton’s) optimal action as a function of the other firm’s action (K2’s). In other words, this equation describes Burton’s reaction curve. But here, the actions are price choices rather than quantity choices as in the Cournot model.

K2 has a reaction curve, too. It looks similar, but is a little different than Burton’s because K2’s demand curve is slightly different. Going through the same steps as above, we have

MR_K = 900 – 4p_K + p_B = 0

4p_K = 900 + p_B

p_K = 225 + 0.25p_B

An interesting detail to note about these reaction curves in the Bertrand differentiated-product model is that a firm’s optimal price increases when its competitor’s price increases. If Burton thinks K2 will charge a higher price, for example, Burton wants to raise its price. That is, the reaction curves are upward-sloping. This is the opposite of the quantity reaction curves in the Cournot model (review Figure 11.4). There, a firm’s optimal response to a competitor’s output change is to do the opposite: If a firm expects its competitor to produce more, then it should produce less.

Differentiated Bertrand Equilibrium: A Graphical Approach Figure 11.5 plots Burton and K2’s reaction curves. The vertical axis shows Burton’s optimal profit-maximizing price; the horizontal axis represents K2’s optimal profit-maximizing price. The positive slope of Burton’s reaction curve indicates that Burton’s profit-maximizing price rises when K2 charges more. The positive slope of K2’s reaction curve indicates that K2’s profit-maximizing price rises when Burton charges more. If Burton expects K2 to charge $100, then Burton should price its boards at $250 (point A). If instead Burton believes K2 will price at $200, then it should price at $275 (point B). A K2 price of $400 will make Burton’s optimal response $325 (point C), and so on. K2’s reaction curve works the same way.

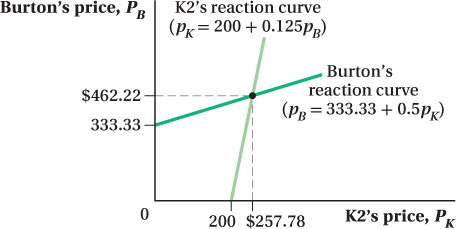
The point where the two reaction curves cross, E, is the Nash equilibrium. There, both firms are doing as well as they can given the other’s actions. If either were to decide on its own to change its price, that firm’s profit would decline.

Differentiated Bertrand Equilibrium: A Mathematical Approach We can algebraically solve for this Nash equilibrium as we did in the Cournot model—by finding the point at which the reaction curve equations equal one another. Mechanically, that means we substitute one reaction curve into the other, solve for one firm’s optimal price, and then use it to solve for the other firm’s optimal price.

450

First, we plug K2’s reaction curve into Burton’s and solve for Burton’s equilibrium price:

p_B = 225 + 0.25p_K

p_B = 225 + 0.25 × (225 + 0.25p_B)

p_B = 225 + 56.25 + 0.0625p_B

0.9375p_B = 281.25

p_B = 300

Substituting this price into K2’s reaction curve gives its equilibrium price:

p_K = 225 + 0.25p_B = 225 + (0.25 × 300) = 225 + 75 = 300

At equilibrium, both firms charge the same price, $300. This isn’t too surprising. After all, the two firms face similar-looking demand curves and have the same (zero) marginal costs. Interestingly, that particular implication of the identical-products Bertrand oligopoly that we looked at in Section 11.3 (both firms charge the same price in equilibrium) holds here. The difference is that the price no longer equals marginal cost. Instead, equilibrium prices are above marginal cost ($300 is certainly more than zero!).

To figure out the quantity each firm sells, we plug each firm’s price into its demand curve equation. Burton’s quantity demanded is q_B = 900 – 2(300) + 300 = 600 boards. K2 sells q_K = 900 – 2(300) + 300 = 600 boards also. Again, the fact that both firms sell the same quantity is not surprising because they have similar demand curves and charge the same price. Total industry production is therefore 1,200 boards, which is two-thirds of what it would be if both firms charged their marginal costs (each firm in that case would make 900 boards, meaning total production of 1,800 boards). In the Bertrand model where the firms produce differentiated products, each firm earns a profit of 600 × (300 – 0) = $180,000.

451

In this example, both firms had demand curves that were mirror images of each other. If instead the firms had different demand curves, we would go about solving for equilibrium prices, quantities, and profits the same way, but these probably wouldn’t be the same for each firm.