EXAMPLE 3Finding the Principal Unit Normal Vector
Find the principal unit normal vector N(t) to the circle r(t)=Rcosti+Rsintj, 0≤t≤2π. (Refer to Example 2.) Graph r=r(t), and show a unit tangent vector and a unit normal vector.
Solution From Example 2, the unit tangent vector T(t) is T(t)=−sinti+costj
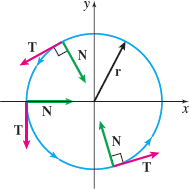
Figure 13  r(t)=Rcosti+Rsintj,0≤t≤2π
r(t)=Rcosti+Rsintj,0≤t≤2π
Since T′(t)=−costi−sintj, the principal unit normal vector N(t) is N(t)=T′(t)‖
Since \mathbf{r}( t) = R ( \cos t\mathbf{i}+\sin t\mathbf{j}), the vector \mathbf{N}( t) is parallel to -\mathbf{r}(t). That is, \mathbf{N}(t) is a unit vector opposite in direction to the vector \mathbf{r}(t), so \mathbf{N} is directed toward the center of the circle, as shown in Figure 13.

