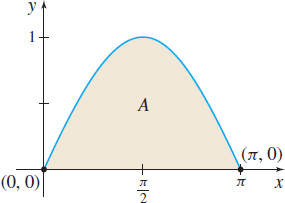
Figure 27 $f(x) = \sin x, 0 \leq x \leq \pi$.

Solution The graph of $f$ is shown in Figure 27. Since $f(x) \geq 0$ for all $x$ in the closed interval $[ 0,\pi ]$, the area $A$ under its graph is given by the definite integral, $\int_{0}^{\pi }\sin x~dx$.

(a) From the Extreme Value Theorem, $f$ has an absolute minimum value and an absolute maximum value on the interval $[0,\pi] .$ The absolute maximum of $f$ occurs at $x=\dfrac{\pi}{2},$ and its value is $f \left( \dfrac{\pi }{2}\right) =\sin \dfrac{\pi }{2}=1.$ The absolute minimum occurs at $x=0$ and at $x=\pi$; the absolute minimum value is $f (0) =\sin 0=0=f (\pi)$. Using the inequalities in (5), the area under the graph of $f$ is bounded as follows:

$$0\leq \int_{0}^{\pi }\sin x~dx\leq \pi$$

(b) The actual area under the graph is $$A= \int_{0}^{\pi}\sin x\,dx= \bigg[-\cos x\bigg] _{0}^{\pi }=-\cos \pi +\cos 0=1+1=2\hbox{ square units}$$