7.1 Integration by PartsPrinted Page 472
472
OBJECTIVES
When you finish this section, you should be able to:
Integration by parts is a technique of integration based on the Product Rule for derivatives: If u=f(x) and v=g(x) are functions that are differentiable on an open interval (a,b), then ddx[f(x)⋅g(x)]=f(x)g′(x)+f′(x)g(x)
Integrating both sides gives ∫ddx[f(x)⋅g(x)]dx=∫[f(x)g′(x)+f′(x)g(x)]dxf(x)⋅g(x)=∫f(x)g′(x)dx+∫f′(x)g(x)dx
Solving this equation for ∫f(x)g′(x)dx yields \bbox[5px, border:1px solid black, #F9F7ED]{\bbox[5pt] {\int f( x) g^\prime ( x) dx = f(x) \cdot g(x) -\int f^\prime ( x) g(x) dx}}
which is known as the integration by parts formula.
Let u=f( x) , v=g( x) . Then we can use their differentials, du=f^{\prime} ( x) dx and dv=g^{\prime} ( x) dx to obtain the formula in the form we usually use: \bbox[5px, border:1px solid black, #F9F7ED]{\bbox[5pt] {\int u~dv=uv-\int v~du}}
IN WORDS
The integral of the product u dv equals u times v minus the integral of the product v du.
1 Integrate by PartsPrinted Page 472
The goal of integration by parts is to choose u and dv so that \int v~du is easier to integrate than \int u~dv.
EXAMPLE 1Integrating by Parts
Find \int x e^x~dx.
Solution Choose u and dv so that \int u dv = \int x e^x~dx
Suppose we choose u = x\qquad \text{and} \quad dv = e^x~dx
Then du = dx\qquad \text{and} \qquad v = \int dv = \int e^x~dx=e^x
Notice that we did not add a constant. Only a particular antiderivative of dv is required at this stage; we will add the constant of integration at the end. Using the integration by parts formula, we have \int \underset{\color{#0066A7}{\hbox{\(u\)}}}{\underbrace{x}} \underset{\color{#0066A7}{dv}}{\underbrace{e^{x} dx}} = \underset{\color{#0066A7}{uv}}{\underbrace{x e^{x}}}-\int \underset{\color{#0066A7}{v}}{\underbrace{e^{x}}}\underset{\color{#0066A7}{\hbox{\(du\)}}} {\underbrace{\hbox{\(dx\)}}}=x e^{x}-e^{x}+C=e^{x}(x-1)+C
473
We intentionally chose u=x and dv=e^{x}dx so that \int v~du in the formula is easy to integrate. Suppose, instead, we chose u = e^x \quad \text{and} \quad dv = x~dx
Then du = e^xdx \quad \text{and} \quad v = \int xdx = \dfrac {x^{2}}{2}
The integration by parts formula yields \int x e^{x} dx=\int \underset{\color{#0066A7}{\hbox{\(u\)}}}{\underbrace{e^{x}}} \underset{\color{#0066A7}{dv}}{\underbrace{x~dx}} = \underset{\color{#0066A7}{uv}}{\underbrace{e^{x} \dfrac{x^{2}}{2}}}-\int \underset{\color{#0066A7}{v}}{\underbrace{\dfrac{x^{2}}{2}}} \underset{\color{#0066A7}{\hbox{\(du\)}}}{\underbrace{e^{x} dx}}
For this choice of u and v, the integral on the right is more complicated than the original integral, indicating an unwise choice of u and dv.
IN WORDS
We choose dv so that it can be easily integrated and choose u so that \int v du is simpler than \int u dv.
NOW WORK
EXAMPLE 2Integrating by Parts
Find \int x sin x~dx.
Solution We use the integration by parts formula with u=x \qquad\hbox{and}\qquad dv=\sin x\,dx\qquad {\color{#0066A7}{\int}} {\color{#0066A7}{udv=}} {\color{#0066A7}{\int x\sin x\,dx}}
Then du = dx \quad and \quad v = \int \sin x~dx = -cos x
and \begin{eqnarray*} \int x\sin x\,dx \underset{\underset{\color{#0066A7}{\hbox{\(\int udv= uv - \int vdu\)}}}{\color{#0066A7}{\uparrow}}} {=} -x\cos x+\int \cos x\,dx=-x\cos x+\sin x+C \\[-9pt] \end{eqnarray*}
NOW WORK
Unfortunately, there are no exact rules for choosing u and dv. But the following guidelines are helpful:
Integration by Parts: Guidelines for Choosing u and dv
- dx is always part of dv.
- dv should be easy to integrate.
- u and dv are chosen so that \int v du is no more difficult to integrate than the original integral \int u dv.
- If the new integral is more complicated, try different choices for u and dv.
Choosing u and dv often involves trial and error. If a selection does not appear to work, try a different choice. If no choice works, it may be that some other technique of integration should be used.
474
EXAMPLE 3Integrating by Parts to Find \int \ln x\, dx
Derive the formula \bbox[5px, border:1px solid black, #F9F7ED]{ \int \ln x\,dx=x\ln x-x+C }
Solution We use the integration by parts formula with \begin{equation*} \hbox{ }u=\ln x\qquad \hbox{and}\qquad dv=dx \end{equation*}
Then du=\frac{1}{x}\,dx\qquad {\rm and}\qquad v=\int dx=x \
Now \int \ln x\,dx= {{x}}\cdot {{\ln x}}-\int {{x}}\cdot {{\frac{1}{x}\,dx}}\,=x\,\ln x-\int dx=x\,\ln x-x+C
NOTE
The integral \int \ln x\,dx can be found in the list of integrals on the inserts at the front and back of the book and is a basic integral.
EXAMPLE 4Integrating by Parts to Find \int \tan^{-1} x\, dx
Derive the formula \bbox[5px, border:1px solid black, #F9F7ED]{ \int \tan ^{-1}x\,dx=x\,\tan ^{-1}x-\dfrac{1}{2}\ln \,(1+x^{2})+C }
Solution We use the integration by parts formula with u=\tan ^{-1}x\qquad \hbox{and }\qquad dv=dx
Then du=\frac{1}{1+x^{2}}\,dx\qquad \hbox{and}\qquad v=\int dx=x and \int \tan ^{-1}x\,dx=x\cdot \tan ^{-1}x-\int \frac{x}{1+x^{2}}dx
To find the integral \int \dfrac{x}{1+x^{2}}dx, we use the substitution t=1+x^{2}. Then dt=2x\,dx, or equivalently, x~dx=\dfrac{dt}{2}. \begin{equation*} \int \frac{x}{1+x^{2}}dx=\frac{1}{2}\int \frac{dt}{t}=\frac{1}{2}\ln \vert \,t\vert =\frac{1}{2}\ln (1+x^{2}) \end{equation*}
As a result, \int \tan ^{-1} x \,dx=x \tan ^{-1}x-\dfrac{1}{2}\ln (1+x^{2})+C.
NEED TO REVIEW?
The method of substitution is discussed in Section 5.6, pp. 387-393.
NOW WORK
The next example shows that sometimes it is necessary to integrate by parts more than once.
EXAMPLE 5Integrating by Parts
Find \int x^{2}\,e^{x}\,dx.
Solution We use the integration by parts formula with u=x^{2}\qquad \hbox{and}\qquad dv=e^{x}\,dx
Then du=2x\,dx\qquad \hbox{and}\qquad v=\int e^{x}\,dx=e^{x}
475
and \int x^{2} e^{x}\,dx=x^{2}e^{x}-2\int xe^{x}\,dx
The integral on the right is simpler than the original integral. To find it, we use integration by parts a second time. (Refer to Example 1.)
\begin{equation*} \int xe^{x}\,dx=xe^{x}-e^{x} \end{equation*}
Then \int x^{2}e^{x}\,dx=x^{2}e^{x}-2( xe^{x}-e^{x}) +C=e^{x}(x^{2}-2x+2)+C
NOW WORK
Table 1 provides guidelines to help choose u and dv for several types of integrals that are found using integration by parts. In the table, n is always a positive integer.
| Integral; n is a positive integer | u | dv |
|---|---|---|
| \left. \begin{array}{l} \int x^{n}e^{ax}\,dx \\ \int x^{n}\cos (ax) \,dx \\ \int x^{n}\sin (ax) \,dx \end{array} \right\} | u=x^{n} | dv= \hbox{what remains} |
| \begin{array}{l} \int x^{n}\sin ^{-1}x\,dx \\ \int x^{n}\cos ^{-1}x\,dx \\ \int x^{n}\tan ^{-1}x\,dx \end{array} | \begin{array}{l} u=\sin ^{-1}x \\ u=\cos ^{-1}x \\ u=\tan ^{-1}x \end{array} | dv=x^{n}\,dx |
| \int x^{m}( \ln x) ^{n}\,dx; m is a real number, m ≠ -1 | u=( \ln x) ^{n} | dv=x^{m}\,dx |
Integration by parts is also used to find certain definite integrals.
EXAMPLE 6Finding the Area Under the Graph of f(x)=x\ln x
Find the area under the graph of f( x) =x\ln x from 1 to 2.
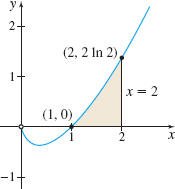
Solution See Figure 1 for the graph of f( x) =x\ln x. The area A under the graph of f from 1 to 2 is A=\int_{1}^{2}x\ln x\,dx. We use the integration by parts formula with \begin{equation*} u=\ln x\qquad \hbox{and}\qquad dv=x\,dx \end{equation*}
Then \begin{equation*} du=\frac{1}{x} dx\qquad \hbox{and}\qquad v=\int x dx=\frac{x^{2}}{2} \end{equation*}
and \begin{eqnarray*} A=\int_{1}^{2}x\ln x\,dx&=&\left[ \frac{x^{2}}{2}\ln x\right] _{1}^{2}-\int_{1}^{2}\frac{x^{2}}{2}\left( \frac{1}{x} dx\right) =2\ln 2-\dfrac{1}{2}\int_{1}^{2}x\,dx\\[5pt] &=&2\ln 2- \dfrac{1}{2}\left[ \frac{x^{2}}{2}\right] _{1}^{2}=2\ln 2-\dfrac{3}{4} \end{eqnarray*}
NOW WORK
2 Derive a Formula Using Integration by PartsPrinted Page 476
476
Integration by parts is also used to derive general formulas involving integrals.
EXAMPLE 7Deriving a Formula
Derive the formula \bbox[5px, border:1px solid black, #F9F7ED]{ \int e^{ax}\cos (bx) \,dx=\dfrac{e^{ax}[b\sin (bx) +a\cos (bx)] }{a^{2}+b^{2}}+C\qquad b ≠ 0} \tag{1}
Solution We use the integration by parts formula with u=e^{ax}\qquad \hbox{and} \qquad dv=\cos (bx) \,dx
Then \begin{equation*} du=ae^{ax}\,dx\qquad \hbox{and}\qquad v=\int \cos (bx) \,dx=\frac{1}{b}\sin (bx) \end{equation*}
and \begin{equation*} \int e^{ax}\cos (bx) \,dx=e^{ax}\,\dfrac{\sin (bx) }{b}-\frac{a}{b}\int e^{ax}\sin (bx) \,dx \tag{2} \end{equation*}
The new integral on the right, \int e^{ax}\sin (bx) \,dx, is different from the original integral, but it is essentially of the same form. We use integration by parts again with this integral by choosing \begin{equation*} u=e^{ax}\qquad \hbox{and}\qquad dv=\sin (bx) \,dx \end{equation*}
Then \begin{equation*} du=ae^{ax}\,dx\qquad \hbox{and}\qquad v=\int \sin (bx) \,dx=- \frac{1}{b}\cos (bx) \end{equation*}
and \begin{equation*} \int e^{ax}\sin (bx) \,dx=-\frac{1}{b}\,e^{ax}\cos (bx) +\frac{a}{b}\int e^{ax}\cos (bx) \,dx\tag{3} \end{equation*}
Substituting the result from (3) into (2), we obtain \begin{eqnarray*} \int e^{ax}\cos (bx) \,dx& =& \frac{1}{b}e^{ax}\sin (bx) -\frac{a}{b}\left[ -\frac{1}{b}e^{ax}\cos (bx) + \frac{a}{b}\int e^{ax}\cos (bx) \,dx\right] \\[8pt] \int e^{ax}\cos (bx) \,dx& =&\frac{1}{b}\,e^{ax}\sin (bx) +\frac{a}{b^{2}}\,e^{ax}\cos (bx) -\frac{a^{2}}{b^{2}}\int e^{ax}\cos (bx) \,dx \end{eqnarray*}
Now we solve for \int e^{ax}\cos (bx) \,dx and simplify. \begin{eqnarray*} \int e^{ax}\cos (bx) \,dx+\frac{a^{2}}{b^{2}}\int e^{ax}\cos (bx) \,dx& =&\frac{1}{b}\,e^{ax}\sin (bx) +\frac{a}{ b^{2}}\,e^{ax}\cos (bx) \nonumber \\[5pt] \left( 1+\frac{a^{2}}{b^{2}}\right) \int e^{ax}\cos (bx) \,dx &=&\frac{1}{b^{2}}\,e^{ax} [b\sin (bx) +a\cos (bx) ] \\ \int e^{ax}\cos (bx) \,dx &=&\frac{e^{ax}[b\sin (bx)+a\cos (bx)]}{a^{2}+b^{2}}+C \end{eqnarray*}
477
For example, to find \int e^{4x}\cos (5x) \,dx, we use (1) with a=4 and b=5. \int e^{4x}\cos (5x) \,dx=\frac{e^{4x}[ 5\sin \,(5x) +4\cos \,(5x) ] }{41}+C
NOW WORK
EXAMPLE 8Deriving a Formula
Derive the formula \bbox[5px, border:1px solid black, #F9F7ED]{ \int \sec ^{n} x\,dx=\dfrac{\sec ^{n-2}x\,\tan \,x}{n-1} +\dfrac{n-2}{n-1}\int \sec ^{n-2} x\,dx\qquad n \geq 3}\tag{4}
Solution We begin by writing \sec ^{n}x=\sec ^{n-2}x\sec ^{2}x, and choose u=\sec ^{n-2}x\qquad \hbox{and}\qquad dv=\sec ^{2}x\,dx
This choice makes \int dv easy to integrate. Then \begin{eqnarray*} du&=&[ (n-2)\sec ^{n-3}x\cdot \sec x\,\tan x] \,dx =[ (n-2)\sec ^{n-2}x\,\tan x] \,dx \\[6pt] v&=&\int \sec^{2}x\,dx=\tan x \end{eqnarray*}
Using integration by parts, we get \begin{equation*} \int \sec ^{n}x\,dx=\sec ^{n-2}x\,\tan x-(n-2)\int \sec ^{n-2}x\,\tan ^{2}x\,dx \end{equation*}
To express the integrand on the right in terms of \sec x, we use the trigonometric identity, \tan ^{2}x+1=\sec ^{2}x, and replace \tan ^{2}x by \sec ^{2}x-1, obtaining \begin{eqnarray*} \int \sec ^{n}x\,dx& =&\sec ^{n-2}x\tan x-(n-2)\int \sec ^{n-2}x(\sec^{2}x-1)\,dx \\[4pt] \int \sec ^{n}x\,dx& =& \sec ^{n-2}x\tan x-(n-2)\int \sec ^{n}x\,dx+(n-2)\int \sec ^{n-2}x\,dx \end{eqnarray*}
Moving the middle term on the right to the left, we obtain (n-1)\int \sec ^{n}x\,dx=\sec ^{n-2}x\,\tan x+(n-2)\int \sec ^{n-2}x\,dx
Finally, divide both sides by n-1: \int \sec ^{n}x\,dx=\frac{\sec ^{n-2}x\tan x}{n-1}+\frac{n-2}{n-1}\int \sec ^{n-2}x\,dx
Formula (4) is called a reduction formula because repeated applications of the formula eventually lead to an elementary integral. For this reduction formula, when n is even, repeated applications lead eventually to \bbox[5px, border:1px solid black, #F9F7ED]{ \int \sec ^{2}x\,dx=\tan x+C}
When n is odd, repeated applications eventually lead to the integral \bbox[5px, border:1px solid black, #F9F7ED]{ \int \sec x\,dx=\ln \vert \sec x+\tan x\vert +C}
478
For example, if n=3, \int \sec^{3}x~dx = \frac{\sec x \tan x}{2}+ \frac{1}{2} \int \sec x~dx=\frac{\sec x \tan x}{2}+\frac{1}{2}\ln \vert \sec x+\tan x\vert + C
