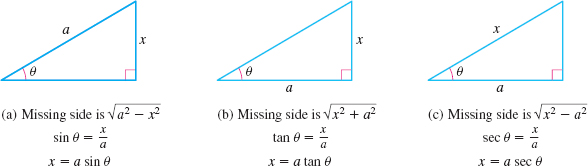
7.3 Integration Using Trigonometric Substitution: Integrands Containing √(a2-x2), √(x2+a2), or √(x2-a2), a > 0
Printed Page 488
488
When an integrand contains a square root of the form √a2−x2, √x2+a2, or √x2−a2, a>0, an appropriate trigonometric substitution will eliminate the radical and sometimes transform the integral into a trigonometric integral like those studied earlier.
The substitutions to use for each of the three types of radicals are given in Table 2.
| Integrand Contains | Substitution | Based on the Identity |
|---|---|---|
| √a2−x2 | x=asinθ, −π2≤θ≤π2 | 1−sin2θ=cos2θ |
| √x2+a2 | x=atanθ, −π2<θ<π2 | tan2θ+1=sec2θ |
| √x2−a2 | x=asecθ, 0≤θ<π2, π≤θ<3π2 | sec2θ−1=tan2θ |
CAUTION
Be careful to use the restrictions on each substitution. They guarantee the substitution is a one-to-one function, which is a requirement for using substitution.
Although the substitutions to use can be memorized, it is often easier to draw a right triangle and derive them as needed. Each substitution is based on the Pythagorean Theorem. By placing the sides a and x on a right triangle appropriately, the third side of the triangle will represent one of the three types of radicals, as shown in Figure 3.
NEED TO REVIEW?
Right triangle trigonometry is discussed in Appendix A.4, pp. A-27 to A-31.
1 Find Integrals Containing √(a2-x2)
Printed Page 488
When an integrand contains a radical of the form √a2−x2, a>0, we use the substitution x=asinθ, −π2≤θ≤π2. Substituting x=asinθ, −π2≤θ≤π2, in the expression √a2−x2 eliminates the radical as follows: √a2−x2=√a2−a2sin2θLet x=asinθ; −π2≤θ≤π2. =a√1−sin2θFactor out a2; a>0.=a√cos2θ1−sin2θ=cos2θ=acosθcosθ≥0 since −π2≤θ≤π2
489
EXAMPLE 1Finding an Integral Containing √4−x2
Find ∫dxx2√4−x2.
NOTE
We exclude θ=−π2 and θ=π2 because they lead to x=±2, resulting in √4−x2=0.
Solution The integrand contains the square root √4−x2 that is of the form √a2−x2, where a=2. We use the substitution x=2sinθ, −π2<θ<π2. Then dx=2cosθdθ. Since √4−x2=↑x=2sinθ√4−4sin2θ=2√1−sin2θ=2√cos2θ=↑cos θ>0 since−π2<θ<π22cosθ
we have ∫dxx2√4−x2=∫2cosθdθ(4sin2θ)(2cosθ)=∫dθ4sin2θ=14∫csc2θdθ=−14cotθ+C
The original integral is a function of x, but the solution above is a function of θ. To express cotθ in terms of x, refer to the right triangles drawn in Figure 4.
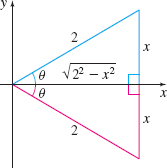
Using the Pythagorean Theorem, the third side of each triangle is √22−x2=√4−x2. So, cotθ=√4−x2x−π2<θ<π2
Then ∫dxx2√4−x2=−14cotθ+C=−14√4−x2x+C=−√4−x24x+C
Alternatively, trigonometric identities can be used to express cotθ in terms of x. Using identities, we get cotθ=cosθsinθ=↑cos2θ=1−sin2θcosθ>0√1−sin2θsinθ=↑x=2sinθsinθ=x2√1−(x2)2x2=2√1−x24x=√4−x2x
NOW WORK
2 Find Integrals Containing √(x2+a2)
Printed Page 489
When an integrand contains a radical of the form √x2+a2, a>0, we use the substitution x=atanθ, −π2<θ<π2. Substituting x=atanθ, −π2<θ<π2, in the expression √x2+a2 eliminates the radical as follows: √x2+a2=↑x=atanθ√a2tan2θ+a2=↑Factor out a,a>0a√tan2θ+1=a√sec2θ=↑sec θ>0since−π2<θ<π2asecθ
490
EXAMPLE 2Finding an Integral Containing √x2+9
Find ∫dx(x2+9)3/2.
Solution The integral contains a square root (x2+9)3/2=(√x2+9)3 that is of the form √x2+a2, where a=3. We use the substitution x=3tanθ, −π2<θ<π2. Then dx=3sec2θdθ. Since (x2+9)3/2=↑x=3tanθ(9tan2θ+9)3/2=93/2(tan2θ+1)3/2=↑tan2θ+1=sec2θ27(sec2θ)3/2=↑secθ>027sec3θ

we have ∫dx(x2+9)3/2=∫3sec2θdθ27sec3θ=19∫dθsecθ=19∫cosθdθ=19sinθ+C
To express the solution in terms of x, use either the right triangles in Figure 5 or identities.
From the right triangles, the hypotenuse is √x2+32=√x2+9. So, sinθ=x√x2+9. Then ∫dx(x2+9)3/2=19sinθ+C=x9√x2+9+C
NOW WORK
NOTE
An integral containing √x2+a2, a>0, can also be found using the substitution x=asinhθ, since √x2+a2=√a2sinh2θ+a2=a√sinh2θ+1=a√cosh2θ=acoshθ
Try it!
EXAMPLE 3Finding the Integral ∫(4x2+9)1/2dx
Find ∫(4x2+9)1/2dx.
Solution ∫(4x2+9)1/2dx=∫√(2x)2+32dx
We use the substitution 2x=3tanθ, −π2<θ<π2. Then dx=32sec2θdθ and ∫(4x2+9)1/2dx=32∫√9tan2θ+9sec2θdθ=92∫√tan2θ+1sec2θdθ=92∫sec3θdθ=92[12secθtanθ+12ln|secθ+tanθ|]+C

To express the solution in terms of x, refer to the right triangles drawn in Figure 6.
Using the Pythagorean Theorem, the hypotenuse of each triangle is √(2x)2+9=√4x2+9. So, secθ=√4x2+93andtanθ=2x3−π2<θ<π2
491
Then ∫(4x2+9)1/2dx=94[secθtanθ+ln|secθ+tanθ|]+C=94[√4x2+93⋅2x3+ln|√4x2+93+2x3|]+C=94[2x√4x2+99+ln2x+√4x2+93]+C
In general, if the integral contains √b2x2+a2, we use the substitution bx=atanθ (x=abtanθ), −π2<θ<π2.
NOW WORK
3 Find Integrals Containing √(x2-a2)
Printed Page 491
NOTE
The substitution x=acoshθ can also be used for integrands containing √x2−a2.
When an integrand contains √x2−a2, a>0, we use the substitution x=asecθ, 0≤θ<π2, π≤θ<3π2. Then
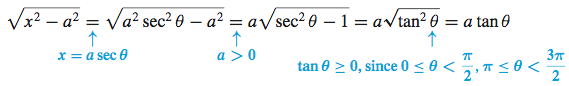
EXAMPLE 4Finding an Integral Containing √x2−4
Find ∫√x2−4xdx.
Solution The integrand contains the square root √x2−4 that is of the form √x2−a2, where a=2. We use the substitution x=2secθ, 0≤θ<π2, π≤θ<3π2. Then dx=2secθtanθ dθ. Since √x2−4=↑x=2secθ√4sec2θ−4=2√sec2θ−1=2√tan2θ=↑tanθ≥0since 0≤θ<π2,π≤θ<3π22tanθ
we have ∫√x2−4xdx=∫(2tanθ)(2secθtanθdθ)2secθ=2∫tan2θdθ=↑tan2θ=sec2θ−12∫(sec2θ−1)dθ=2∫sec2θdθ−2∫dθ=2tanθ−2θ+C
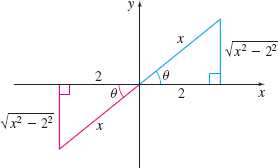
To express the solution in terms of x, we use either the right triangles in Figure 7 or trigonometric identities.
Using identities, we find, tanθ=↑tan2θ=sec2θ−1tanθ≥0√sec2θ−1=√x24−1=12√x2−4
Also since secθ=x2, 0≤θ<π2, π≤θ<3π2, the inverse function θ=sec−1x2 is defined.
492
Then ∫√x2−4xdx=2tanθ−2θ+C=√x2−4−2sec−1x2+C
NOW WORK
4 Use Trigonometric Substitution to Find Definite IntegralsPrinted Page 492
Trigonometric substitution is also useful when finding certain types of definite integrals.
EXAMPLE 5Finding the Area Enclosed by an Ellipse
Find the area A enclosed by the ellipse x24+y29=1.
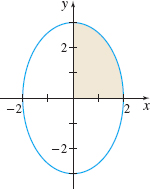
Solution Figure 8 illustrates the ellipse. Since the ellipse is symmetric with respect to both the x-axis and the y-axis, the total area A of the ellipse is four times the shaded area in the first quadrant, where 0≤x≤2 and 0≤y≤3.
We begin by expressing y as a function of x. x24+y29=1y29=1−x24=4−x24y2=94(4−x2)y=32√4−x2y≥0
So, the area A of the ellipse is four times the area under the graph of y=32√4−x2, 0≤x≤2. That is, A=4∫2032√4−x2dx=6∫20√4−x2dx
Since the integrand contains a square root of the form √a2−x2 with a=2, we use the substitution x=2sinθ, −π2≤θ≤π2. Then dx=2cosθdθ. The new limits of integration are:
- When x=0, 2sinθ=0, so θ=0.
- When x=2, 2sinθ=2, so sinθ=1 and θ=π2.
Then A=6∫20√4−x2dx=6∫π/20√4−4sin2θ⋅2cosθdθ=6∫π/202√1−sin2θ⋅2cosθdθ=24∫π/20√cos2θ⋅cosθdθ=↑cosθ≥024∫π/20cos2θdθ=↑cos2θ=1+cos(2θ)2242∫π/20[1+cos(2θ)]dθ=12[θ+12sin(2θ)]π/20=12(π2+0)=6π
The area of the ellipse is 6π square units.
NOW WORK
493
In Example 5, we changed the limits of integration to find the definite integral, so there was no need to change back to the variable x. But it is not always easy to obtain new limits of integration, as we see in the next example.
NEED TO REVIEW?
The two approaches to finding a definite integral using the method of substitution are discussed in Section 5.6, pp. 387-393.
EXAMPLE 6Use Trigonometric Substitution to Find a Definite Integral
Find the area under the graph of y=√x2−1 (the upper half of the right branch of the hyperbola y2=x2−1) from 1 to 3. See Figure 9.
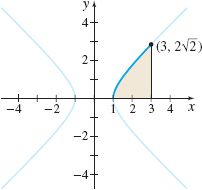
Solution The area A we seek is A=∫31√x2−1dx. The integral contains a square root of the form √x2−a2, where a=1, so we use the trigonometric substitution x=secθ, 0≤θ<π2, π≤θ<3π2. Then dx=secθtanθdθ. Since the upper limit x=3 does not result in a nice angle (θ=sec−13), we find the indefinite integral first and then use the Fundamental Theorem of Calculus.
With x=secθ and dx=secθtanθdθ, we have A=∫√x2−1dx=∫√sec2θ−1secθtanθdθ=∫tanθ⋅secθtanθdθ=∫tan2θsecθdθ
Since tanθ is raised to an even power and secθ to an odd power, we use the identity tan2θ=sec2θ−1.Then A=∫√x2−1dx=∫tan2θsecθdθ=∫(sec2θ−1)secθdθ=∫sec3θdθ−∫secθdθ=12[secθtanθ+ln|secθ+tanθ|]−ln|secθ+tanθ|+C=12secθtanθ−12ln|secθ+tanθ|+C
RECALL
∫sec3θdθ=12[secθtanθ+ln|secθ+tanθ|]. Either integrate by parts, or use the reduction formula.
Now we express tanθ in terms of x=secθ, and apply the Second Fundamental Theorem of Calculus. A=∫31√x2−1dx=↑secθ=xtanθ=√x2−1[12x√x2−1−12ln|x+√x2−1|]31=32√8−12ln(3+√8)=3√2−12ln(3+2√2)